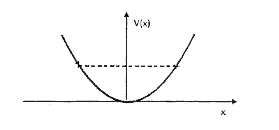
To potential energy function for a particle executing linear simple harmonic motion is given V(x) = kx2/2 , where k is the force constant of the oscillator . For k = 0.5Nm−1 the graph of V(x) versus x is shown in Fig. Show that a particle of total energy 1 J moving under this potential must 'turn back' when it reaches x=±2m
 Solution in Telugu
Solution in Telugu
At any instant, the total energy of an oscillator is the sum of K.E and P.E i.e.
E=K.E+P.E,E=12mu2+12kx2
The particles turns back at the instant, when its velocity becomes zero i.e. u = 0
∴e=0+12kx6(2) as E =1 Joule and K=12N/m
∴1=12×12x2(or)x2=4,x=±2m
Similar Questions
The potential energy function for a particle executing simple harmonic motion is given by V(x)=12kx2, where k is the force constant of the oscillatore. For k=12Nm−1, show that a particle of total energy 1 joule moving under this potential must turn back when it reaches x=±2m.
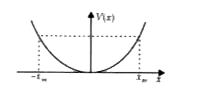
View Solutionसरल रेखीय गति कर रहे किसी कण के लिए स्थितिज ऊर्जा फलन को V(x)=12kx2 के द्वारा निरूपित किया जाता है, जहाँ दोलित्र का बल गुणांक है। k=0.5Nm−1 के लिए, V(x) बनाम x के आरेख को चित्र में दर्शाया गया है। कुल ऊर्जा E का कण उस समय वापस हो जाता है जब यह x=±xm पर पहुँचता है। यदि x=±xm पर किसी कण की स्थितिज व गतिज ऊर्जा को क्रमश: V व K के द्वारा दर्शाया जाता है, तब निम्न में से कौन-सा सही है?
View SolutionThe potential energy function for a particle executing simple harmonic motion is given by V(x)=12kx2, where k is the force constant of the oscillatore. For k=12Nm−1, show that a particle of total energy 1 joule moving under this potential must turn back when it reaches x=±2m.
View SolutionThe potential energy function for a particle executing linear simple harmonic motion is given U(x)=1/2kx^(2) , where k is the force constant of the oscillator. For k=0.5 Nm^(-1) , the graph of U(x) on Y-axis x is shown figure. The particle has total energy 1J. Moving this potential it turns back when it reaches x=+- _________m
View SolutionThe potential energy function for a particle executing linear simple harmonic motion is given by V(x)=kx22 where k is the force constant of the oscillator . For k=0.5Nm−1 , the graph of V(x) versus x is shown in figure . Show that a particle of total energy 1 J movng under this potential must 'turn back ' when it reaches x=±2m .

View SolutionThe potential energy function for a particle executing linear SHM is given by V(x)=12kx2 wher e k is the force constant of the oscillator (figure). For k = 0.5 N/m ,the graph ofV(x) versus x is shown in the figure . A particle of total energy E turns back when it reaches x=±xm . If V and K indicate the PE and KE , respectively of the particle at x=+xm , then which of the following is correct ?

View SolutionThe potential energy function for a particle executing linear SHM is given by V(x)=12kx2 where k is the force constant of the oscillator. For k=0.5Nm−1, the graph of V(x) versus x is shown in the figure A particle of total energy E turns back when it reaches x=±xm.if V and K indicate the potential energy and kinetic energy respectively of the particle at x=+xm,then which of the following is correct?
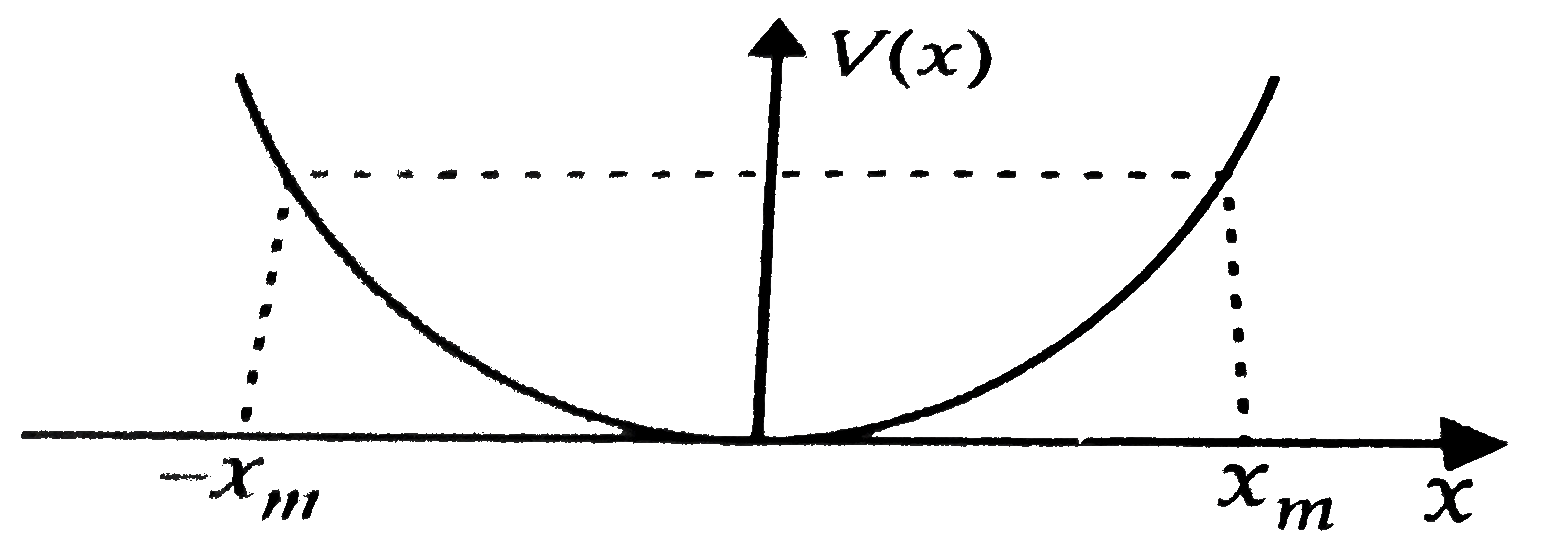
View Solutionसरल आवर्त गति (SHM) करते किसी कण का स्थैितिज ऊर्जा फलन है - V(x)=12kx2 जहाँ k दोलित्र का बल नियतांक है। k = 0.5N/m के लिए V(x) और x का ग्राफ चित्र 6.2 में दर्शाया गया है। E ऊर्जा का कोई कण x=±xm पर पहुँच कर वापस लौटता है। यदि x=+xm पर V एवं K क्रमशः कण की स्थैितिज ऊर्जा (P.E.) एवं गतिज ऊर्जा (K.E.) निरुपित करते हों तो निम्नलिखित में कौन-सा कथन सही है?
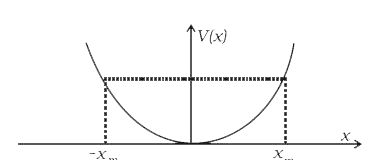
View Solution
