A battery of e.m.f. 15 V and internal resistance 3Ω is connected to two resistors 3 Ω and 6 Ω connected in parallel. Find :
the current in 6 Ω resistor.
To solve the problem step by step, we will follow these steps:
Step 1: Understand the circuit setup
We have a battery with an electromotive force (e.m.f.) of 15 V and an internal resistance of 3 Ω. This battery is connected to two resistors, 3 Ω and 6 Ω, which are connected in parallel.
Step 2: Calculate the equivalent resistance of the parallel resistors
The formula for the equivalent resistance (R_eq) of two resistors (R1 and R2) in parallel is given by:
1Req=1R1+1R2
Substituting the values:
1Req=13+16
Finding a common denominator (which is 6):
1Req=26+16=36=12
Thus, the equivalent resistance is:
Req=2Ω
Step 3: Calculate the total resistance in the circuit
The total resistance (R_total) in the circuit is the sum of the internal resistance of the battery and the equivalent resistance of the parallel resistors:
Rtotal=Rinternal+Req=3Ω+2Ω=5Ω
Step 4: Calculate the total current flowing in the circuit
Using Ohm's law, the total current (I) flowing from the battery can be calculated as:
I=VRtotal=15V5Ω=3A
Step 5: Determine the potential difference across the parallel resistors
The potential drop across the internal resistance of the battery can be calculated as:
Vdrop=I×Rinternal=3A×3Ω=9V
Thus, the potential difference across the parallel resistors is:
Vparallel=Vbattery−Vdrop=15V−9V=6V
Step 6: Calculate the current through the 6 Ω resistor
Using Ohm's law again for the 6 Ω resistor:
I6Ω=VparallelR6Ω=6V6Ω=1A
Final Answer
The current in the 6 Ω resistor is 1 A.
---
We have a battery with an electromotive force (e.m.f.) of 15 V and an internal resistance of 3 Ω. This battery is connected to two resistors, 3 Ω and 6 Ω, which are connected in parallel.
The formula for the equivalent resistance (R_eq) of two resistors (R1 and R2) in parallel is given by:
1Req=1R1+1R2
Substituting the values:
1Req=13+16
Finding a common denominator (which is 6):
1Req=26+16=36=12
Thus, the equivalent resistance is:
Req=2Ω
The total resistance (R_total) in the circuit is the sum of the internal resistance of the battery and the equivalent resistance of the parallel resistors:
Rtotal=Rinternal+Req=3Ω+2Ω=5Ω
Using Ohm's law, the total current (I) flowing from the battery can be calculated as:
I=VRtotal=15V5Ω=3A
The potential drop across the internal resistance of the battery can be calculated as:
Vdrop=I×Rinternal=3A×3Ω=9V
Thus, the potential difference across the parallel resistors is:
Vparallel=Vbattery−Vdrop=15V−9V=6V
Using Ohm's law again for the 6 Ω resistor:
I6Ω=VparallelR6Ω=6V6Ω=1A
The current in the 6 Ω resistor is 1 A.
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - ICSECHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-8(C)
22 Videos
- CURRENT ELECTRICITYBOOK - ICSECHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-8(C) MULTIPLE CHOICE TYPE)
2 Videos
- CURRENT ELECTRICITYBOOK - ICSECHAPTER - CURRENT ELECTRICITYEXERCISE - EXERCISE-8(B) (MULTIPLE CHOICE TYPE)
3 Videos
- CALORIMETRYBOOK - ICSECHAPTER - CALORIMETRYEXERCISE - EXERCISE-11(B) (NUMERICALS)
15 Videos
- ELECTRO-MAGNETISM BOOK - ICSECHAPTER - ELECTRO-MAGNETISM EXERCISE - EXERCISE-10 (C) NUMERICALS
1 Videos
Similar Questions
A battery of e.m.f. 15 V and internal resistance 3Ω is connected to two resistors 3 Ω and 6 Ω connected in parallel. Find :
the current in 3 Ω resistor,
View SolutionA battery of e.m.f. 15 V and internal resistance 3Ω is connected to two resistors 3 Ω and 6 Ω connected in parallel. Find :
the current through the battery,
View SolutionA battery of e.m.f. 15 V and internal resistance 3Ω is connected to two resistors 3 Ω and 6 Ω connected in parallel. Find :
the p.d. between the terminals of the battery,
View SolutionA battery of e.m.f. 12 V and internal resistance 2 Ω is connected with two resistors A and B of resistance 4 Ω and 6 Ω respectively joined in series.
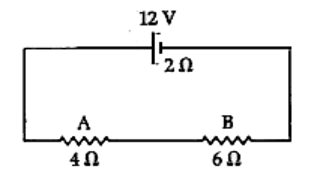
Find The potential difference across 6 Ω Resistor.
View SolutionA battery of e.m.f. 15 V and internal resistance 2 Ω is connected to two resistors of resistances 4 ohm and 6 ohm joined in series. Find the electrical energy spent per minute in 6 ohm resistor.
View SolutionA battery of e.m.f. 12 V and internal resistance 2 Ω is connected with two resistors A and B of resistance 4 Ω and 6 Ω respectively joined in series.
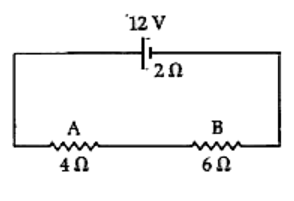
Find Electrical energy spent per minute in 4 Ω Resistor.
View SolutionThese resistors of 10Ω,15Ωand5Ω are connected in parallel. Find their equivalent resistance.
View SolutionTwo resistors having resistance 4 Ω and 6 Ω are connected in parallel. Find their equivalent resistance.
View SolutionA cell of e.m.f. 1.5 V, internal resistance 1 Ω is connected to the resistors of 4 Ω and 20Ω in series. Draw a circuit diagram and calculate :
the current in the circuit,
View SolutionIn the circuit diagram given below, a cell of 9 V and internal resistance of 0.5(Ω) is connected across a resistor A of 2Ω in series and two resistors of 2Ω and 6Ω in parallel.
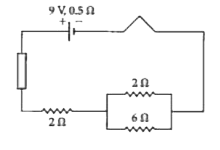
Find
The current in the 6Ω resistor.
View Solution
