A,B and C are three points on a circle. The tagent at C meets BA produced at T. Given thate and that , calculate the angle subtended by AB at the centre of the circle.

The correct Answer is:
Step by step video & image solution for A,B and C are three points on a circle. The tagent at C meets BA produced at T. Given thate /+ATC=36^(@) and that /_ACT=48^(@), calculate the angle subtended by AB at the centre of the circle. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TANGENTS AND INTERSECTING CHORDSBOOK - ICSECHAPTER - TANGENTS AND INTERSECTING CHORDSEXERCISE - EXERCISE 18(A)
24 Videos
- TANGENTS AND INTERSECTING CHORDSBOOK - ICSECHAPTER - TANGENTS AND INTERSECTING CHORDSEXERCISE - EXERCISE 18 (B)
18 Videos
- SPECIMEN QUESTION PAPER (MATHEMATICS)BOOK - ICSECHAPTER - SPECIMEN QUESTION PAPER (MATHEMATICS)EXERCISE - Section C
12 Videos
- TRIGONOMETRICAL IDENTITIESBOOK - ICSECHAPTER - TRIGONOMETRICAL IDENTITIESEXERCISE - QUESTIONS
22 Videos
Similar Questions
In Figure, O is the centre of the circle. Find ∠BAC
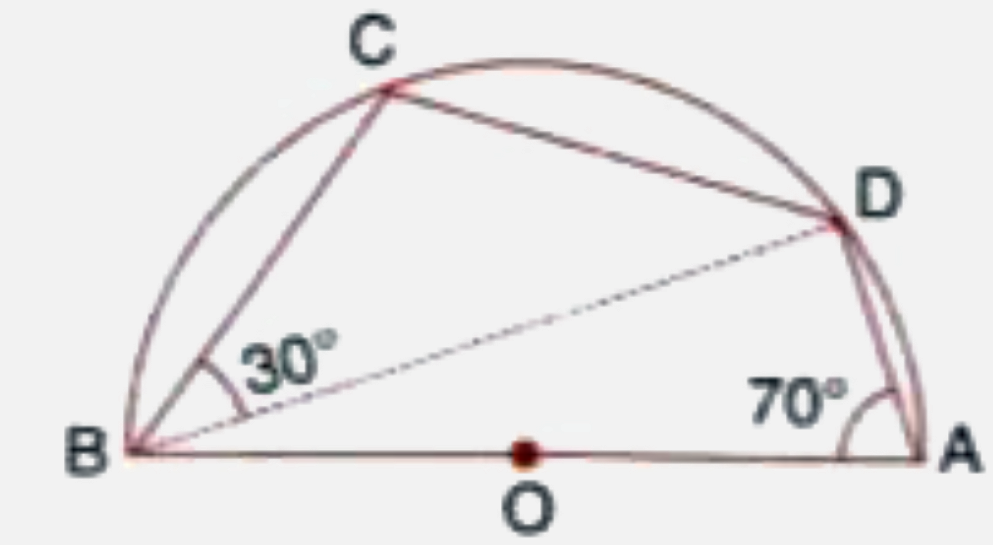
View SolutionIn the given figure, C and D are points on the semi circle described on AB as diameter. Given angle BAD=70∘ and angle DBC=30∘, calculate angle BDC
View SolutionIn the given figure O is the centre of the circle. Tangents at A and B meets at C.
If ∠ACO=30∘, find
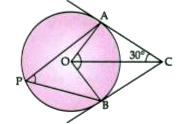
∠BCO
View SolutionIn the given figure O is the centre of the circle. Tangents at A and B meets at C.
If ∠ACO=30∘, find

∠APB
View SolutionIn the given figure O is the centre of the circle. Tangents at A and B meets at C.
If ∠ACO=30∘, find
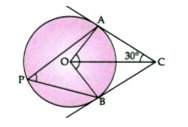
∠AOB
View SolutionTheorem 10.8 : The angle subtended by an arc at the centre is double the angle subtended by it at any point on the remaining part of the circle.
View SolutionABCD is a parallelogram. The circle through A,BandC intersects CD produced at E, prove that AE=AD.
View SolutionIn the given AB is a diameter and DC is tangent which meets AB produced at point. C . If ∠DAC=x∘, find in terms of x∘:
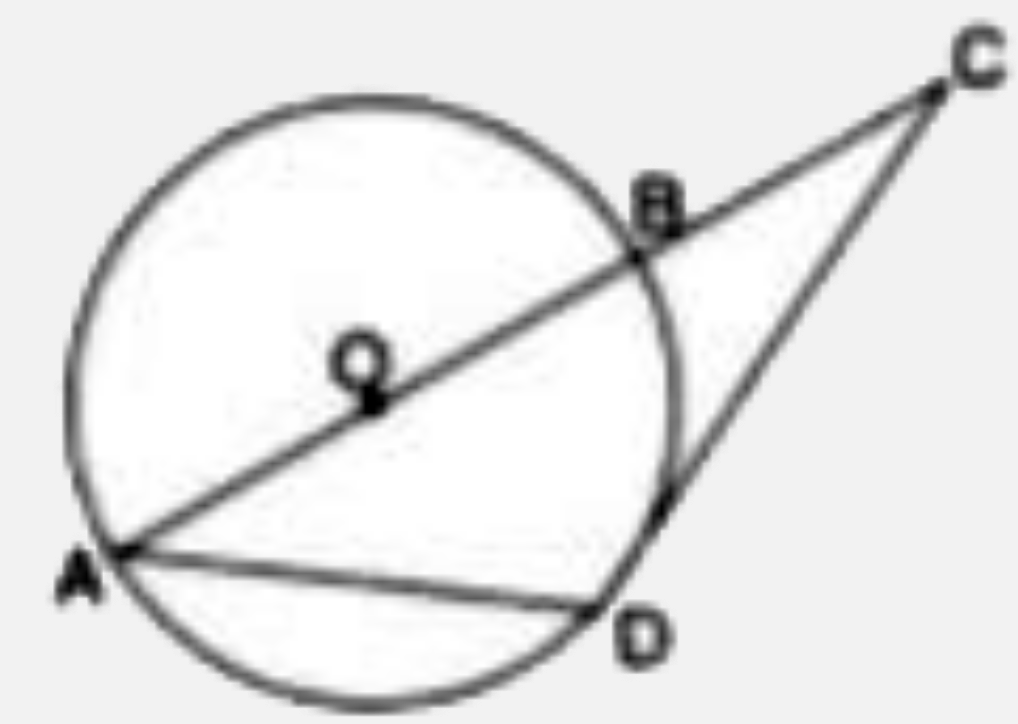
∠DCB
View SolutionIn the given AB is a diameter and DC is tangent which meets AB produced at point. C . If ∠DAC=x∘, find in terms of x∘:

∠DBC
View SolutionIn Fig. 10.36, A,B and C are three points on a circle with centre O such that ∠BOC= 30o and ∠AOB= 60o. If D is a point on the circle other than the arc ABC, find ∠ADC.
View Solution
