A straight line passes through the points A(-5, 2) and B(3,-6). It intersects the co-ordinate axes at points C and D . Find :
the equation of AB.
The correct Answer is:
To find the equation of the line passing through the points A(-5, 2) and B(3, -6), we will follow these steps:
Step 1: Identify the coordinates of points A and B
- A = (-5, 2)
- B = (3, -6)
Step 2: Calculate the slope (m) of the line AB
The formula for the slope (m) between two points (x1, y1) and (x2, y2) is given by:
m=y2−y1x2−x1
Substituting the coordinates of points A and B:
- x1 = -5, y1 = 2
- x2 = 3, y2 = -6
Now, substituting these values into the slope formula:
m=−6−23−(−5)
m=−83+5
m=−88
m=−1
Step 3: Use the point-slope form of the equation of a line
The point-slope form of the equation of a line is:
y−y1=m(x−x1)
Using point A(-5, 2) and the slope m = -1:
y−2=−1(x−(−5))
y−2=−1(x+5)
Step 4: Simplify the equation
Distributing the -1:
y−2=−x−5
Now, adding 2 to both sides:
y=−x−5+2
y=−x−3
Step 5: Rearranging to standard form
To express the equation in standard form (Ax + By + C = 0):
x+y+3=0
Final Equation
Thus, the equation of the line AB is:
x+y+3=0
---
- A = (-5, 2)
- B = (3, -6)
The formula for the slope (m) between two points (x1, y1) and (x2, y2) is given by:
m=y2−y1x2−x1
- x1 = -5, y1 = 2
- x2 = 3, y2 = -6
m=−6−23−(−5)
m=−83+5
m=−88
m=−1
The point-slope form of the equation of a line is:
y−y1=m(x−x1)
y−2=−1(x−(−5))
y−2=−1(x+5)
Distributing the -1:
y−2=−x−5
y=−x−5+2
y=−x−3
To express the equation in standard form (Ax + By + C = 0):
x+y+3=0
Thus, the equation of the line AB is:
x+y+3=0
Topper's Solved these Questions
Similar Questions
A straight line passes through the points A(-5, 2) and B(3,6). It intersects the co-ordinate axes at points C and D . M is a point on AB which divides CD in the ratio 1: 2. Find :
the co-ordinates of points C and D.
View SolutionA straight line passes through the points A(-5, 2) and B(3,6). It intersects the co-ordinate axes at points C and D . M is a point on AB which divides CD in the ratio 1: 2. Find :
the co-ordinates of point M.
View SolutionA straight line passes through the points P(-1, 4) and Q(5,-2). It intersects the co-ordinate axes at points A and B. M is the midpoint of the segment AB. Find :
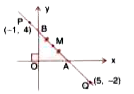
The co-ordinates of A and B.
View SolutionA straight line passes through the points P(-1, 4) and Q(5,-2). It intersects the co-ordinate axes at points A and B. M is the midpoint of the segment AB. Find :
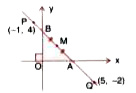
The equation of the line.
View SolutionA straight line passes through the points P(-1, 4) and Q(5,-2). It intersects the co-ordinate axes at points A and B. M is the midpoint of the segment AB. Find :
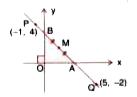
The co-ordinates of M.
View SolutionA straight line passes through the points P(−1,4) and Q(5,−2). It intersects x-axis at point A and y-axis at point B. M is the midpoint of the line segment AB. Find :
the co-ordinates of point M.
View SolutionA straight line passes through the point P(3, 2). It meets the x-axis at point A and the y-axis at point B. If PAPB=23. find the equation of the line that passes through the point P and is perpendicular to line AB.
View SolutionA straight line passes through the point (3,−2) and this point bisects theportion of the line intercepted between the axes, find the equation of the line
View SolutionFind the equation of a plane passes through the point (1,2,3) and cuts equal intercepts on the co-ordinate axes.
View SolutionFind the eqn of lines which pass through the point (1, 2) and equally inclined to the co- ordinate axes.
View Solution
