If OABC is a tetrahedron such that OA2+BC2=OB2+CA2=OC2+AB2 then
OA⊥BC
OB⊥AC
OC⊥AB
AB⊥AC
Let −−→OA=→a,−−→OB=→b,−−→OC=→c
Then from the given conditions.
→a.→a+(→b−→c).(→b−→c)=→b.→b+(→c−→a).(→c−→a)
⇒−2→b.→c=−2→c.→a
⇒→c.(→b−→a)=0
⇒ar(△PQR)=12×4×4=8
Similar Questions
Knowledge Check
- Question 1 - Select One
The OABC is a tetrahedron such that OA2+BC2=OB2+CA2=OC2+AB2,then
AOA⊥BCBOB⊥ACCOC⊥ABDAB⊥AC - Question 2 - Select One
If AD, BE and CF are the medians of a ABC ,then (AD2+BE2+CF2):(BC2+CA2+AB2) is equal to
A4:3B3:2C3:4D2:3 - Question 3 - Select One
OABC is a parallelogram such that OA=a,OB=bandOC=c, then the value →OA.→BA is
Aa2+3b2+c22Ba2−b2+3c22Ca2+3b2−c22D3a2+b2−c22
From a point O in the interior of a ABC , perpendiculars OD, OE and OF are drawn to the sides BC, CA and AB respectively. Prove that: (i) AF2+BD2+CE2=OA2+OB2+OC2−OD2−OE2−OF2 (ii) AF2+BD2+CE2=AE2+CD2+BF2
View SolutionO is any point inside a rectangle ABCD. Prove that OB2+OD2=OA2+OC2.
DEDUCTION In the given figure, O is a point inside a rectangle ABCD such that OB=6cm,OD=8cmandOA=5cm, find the length of OC.
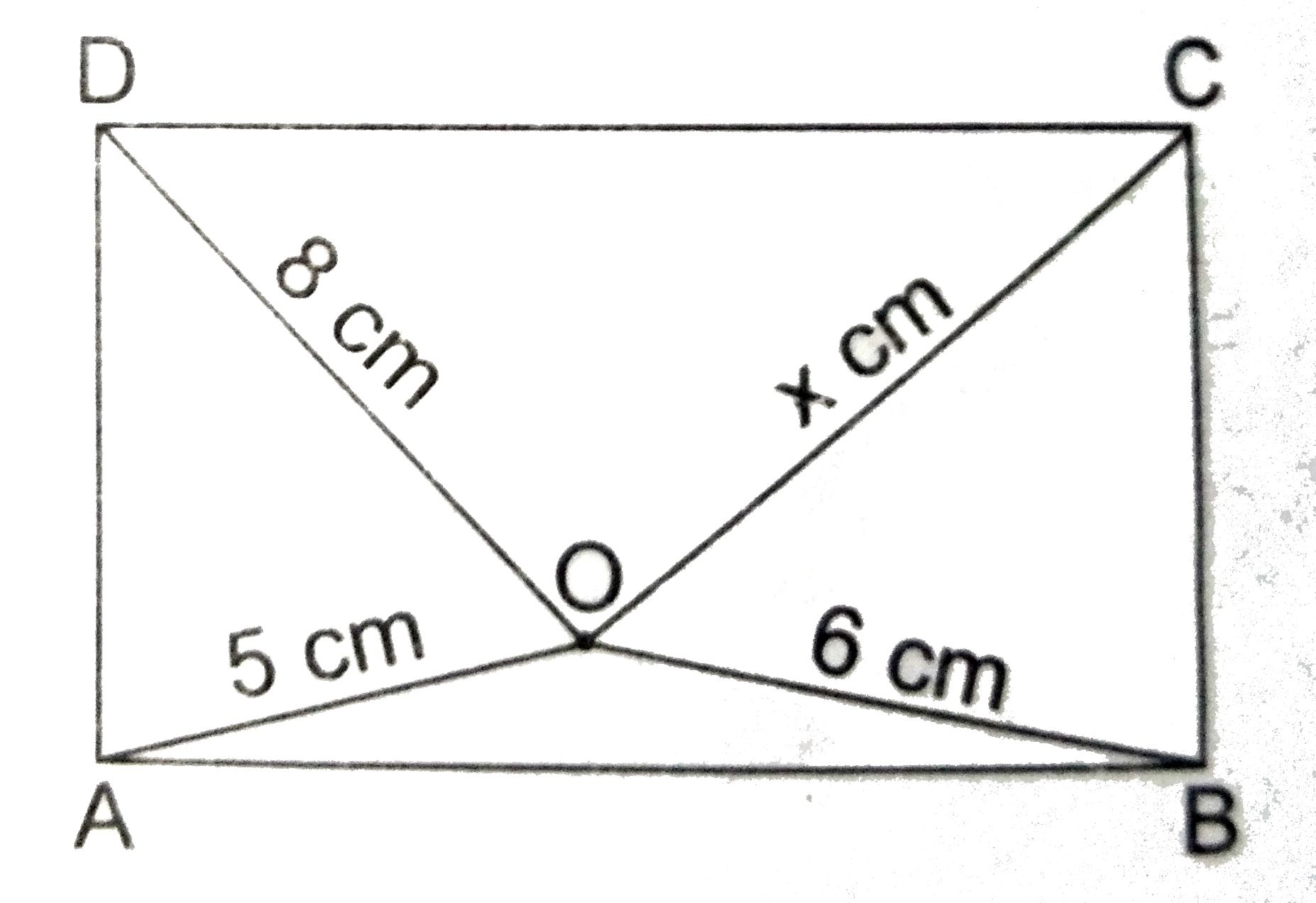 View Solution
View SolutionO is a point in the interior of ΔABC,OD⊥BC,OE⊥ACandOF⊥AB, as shown in the figure,
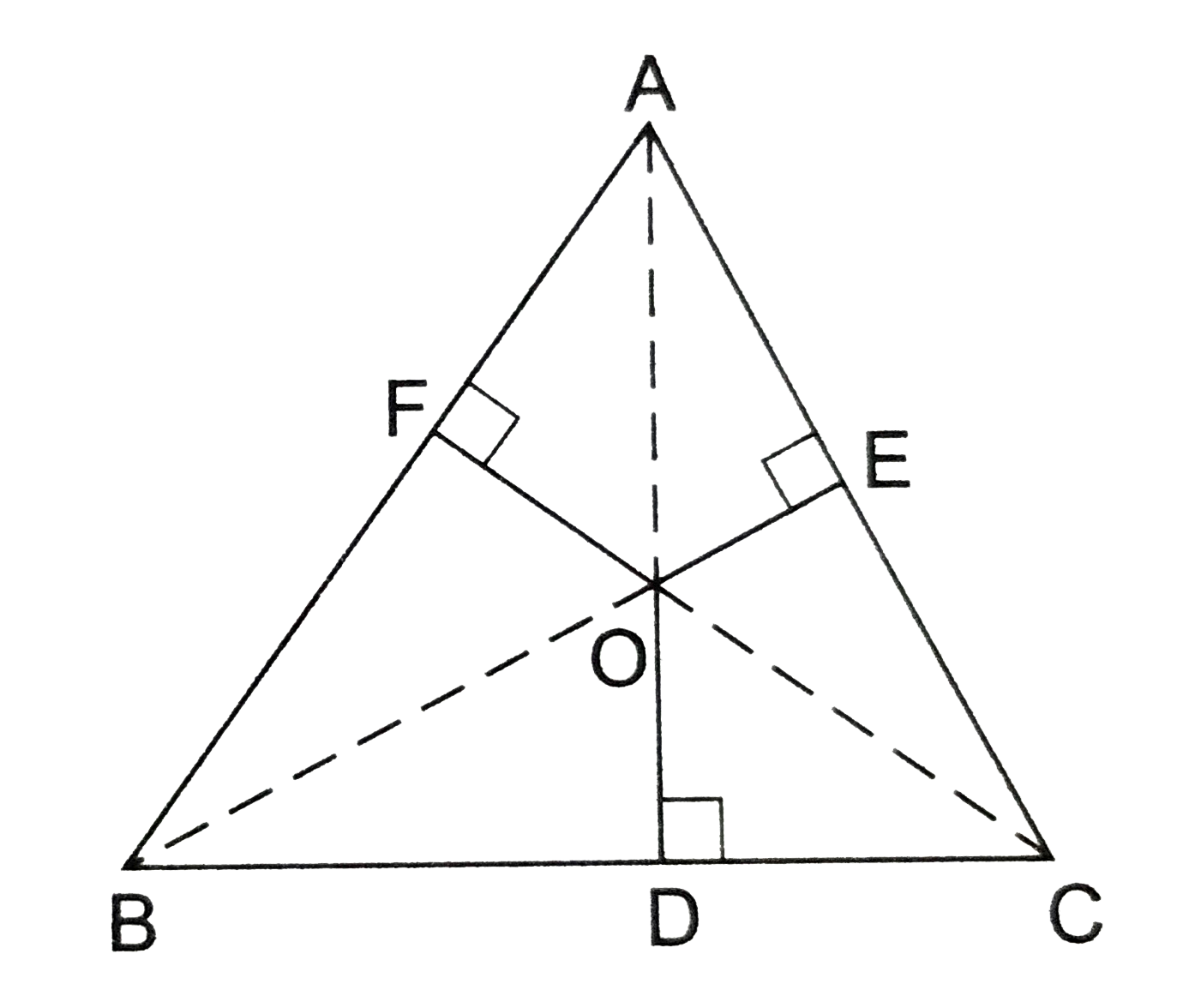
Prove that :
(i)OA2+OB2+OC2−OD2−OE2−OF2=AF2+BD2+CE2
(ii) AF2+BD2+CE2=AD2+BF2+CD2View SolutionIf G be the centroid of the ΔABCandO be any other point in theplane of the triangle ABC, then prove that: OA2+OB2+OC2=GA2+GB2+GB2+GC2+3GO2
View SolutionIf E is a point on side r:A of an equilateral triangle ABC such that BE⊥CA, then prove that AB2+BC2+CA2=4BE2.
View Solution
