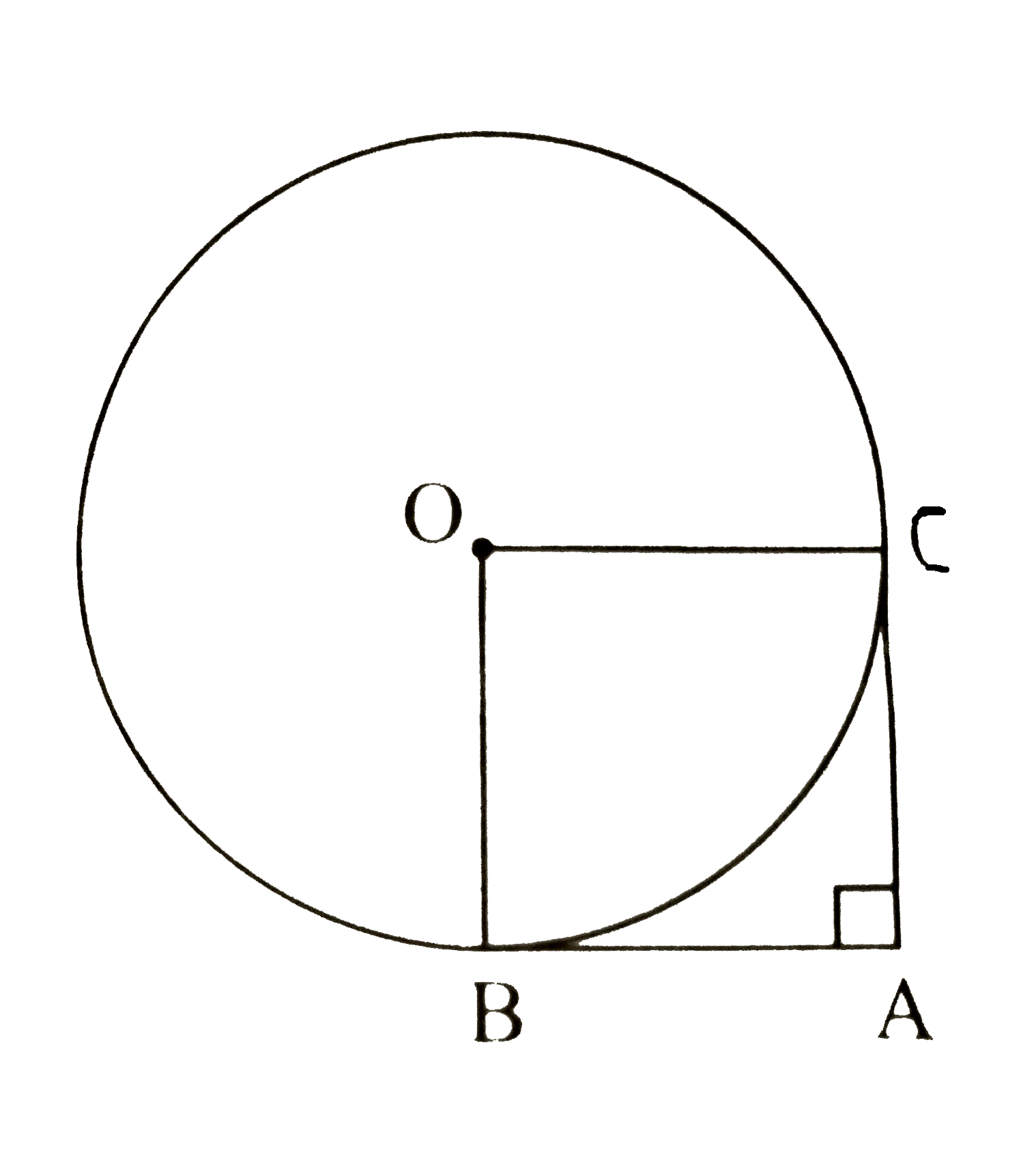
In, the picture, 0 is the centre of the circle and 'A, B, C', are points on it.
'(##VPU_TTT_MAT_X_P01_C02_E03_005_Q01##)'
Prove that '/_ O A C+/_ A B C=90^circ'Solution in Malayalam
Step by step video & image solution for In, the picture, 0 is the centre of the circle and 'A, B, C', are points on it. '(##VPU_TTT_MAT_X_P01_C02_E03_005_Q01##)' Prove that '/_ O A C+/_ A B C=90^circ' by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
One cubic centimetre of iron weighs 7.8 grams. Write as sequences, the volumes of weights of Iron cubes of sides 1 centimetre, 2 centimetres and so on.
View SolutionFind the following:
i) The y coordinate of any point on the 'x' axis.
ii) The 'x' coordinate of any point on the 'y' axis.
iii) The coordinates of the origin.
iv) The y coordinate of any point on the line through (0,1), parallel to the 'x' axis.
v) The x coordinate of any point on the line through (1,0), parallel to the 'y' axis.
View SolutionIf AB, BC and CD are equal chords of a circle with O as centre and AD diameter, then ∠AOB= 600 (b) 900 (c) 1200 (d) none of these
View SolutionIn the figure,tangents at B and C of the circle with centre O intersect at point A. If ∠BAC=90∘ then prove □BACO is a square
View SolutionAOB is a diameter of a circle with centre O and C is any point on the circle, joining A, C, B, C, and O, C, prove that
tan∠ABC=cot∠ACO
View SolutionAOB is a diameter of a circle with centre O and C is any point on the circle, joining A, C, B, C, and O, C, prove that
sin2∠BCO+sin2∠ACO=1
View SolutionAOB is a diameter of a circle with centre O and C is any point on the circle, joining A, C, B, C, and O, C, prove that
cosec2∠CAB−1=tan2∠ABC.
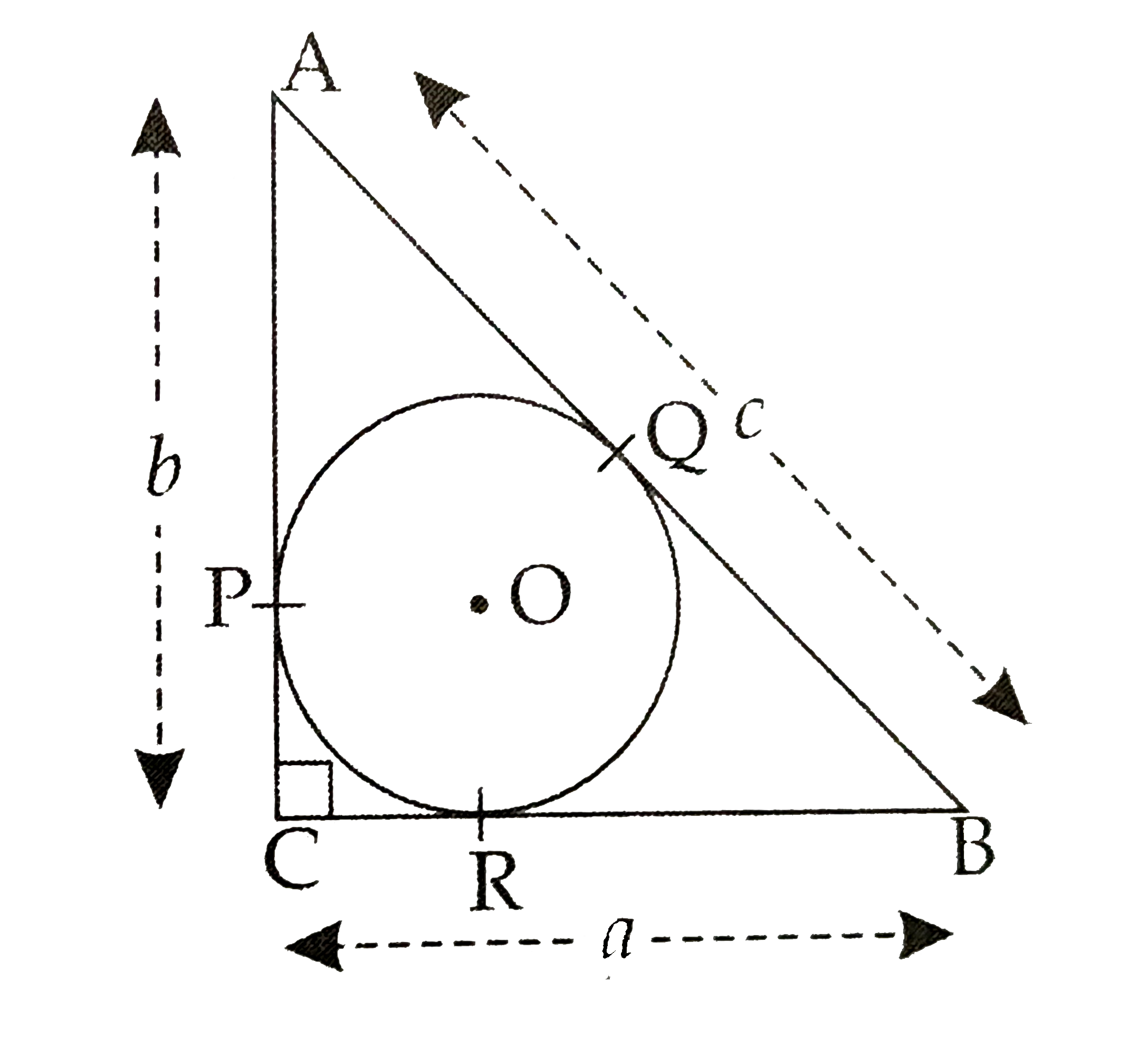
View SolutionA circle with centre 'O' is incircle of ΔABC.ΔBCA=90∘ . Radius of the circle is r.
Prove that : 2r = a + b - c.
View SolutionXYand X'Y'are two parallel tangents to a circle with centre O and another tangent AB with point of contact C intersecting XYat A and X'Y'at B. Prove that ∠AOB = 900
View SolutionIf two chords AB and AC of a circle with centre O are such that the centre O lies on the bisector of ∠BAC, prove that AB=AC , i.e. the chords are equal.
View SolutionIn Figure, O is the centre of the circle and BCD is tangent to it at C. Prove that ∠BAC+∠ACD=900 .
View SolutionIn Figure, ˆAB≅ˆAC and O is the centre of the circle. Prove that OA is the perpendicular bisector of BC
View SolutionIn Figure, AB=CB and O is the centre of the circle. Prove that BO bisects ∠ABC.
View SolutionIf AB, BC and CD are equal chords of a circle with O as centre and AD diameter, then ∠AOB= (a) 600 (b) 900 (c) 1200 (d) none of these
View SolutionAn equilateral triangle ABC is inscribed in a circle with centre O. The measures of ∠BOC is
View SolutionIf A, B, C are three points on a circle with centre O such that ∠AOB=900 and ∠BOC=1200, then ∠ABC= (a)600 (b) 750 (c) 900 (d) 1350
View Solution
