A light ray enters into a medium whose refractive index varies along the x-axis as n(x)=n0√1+x4 where n0=1. The medium is bounded by the plates x=0,x=1 & y=0. It the ray enters at the origin at an angle 30∘ with x-axis

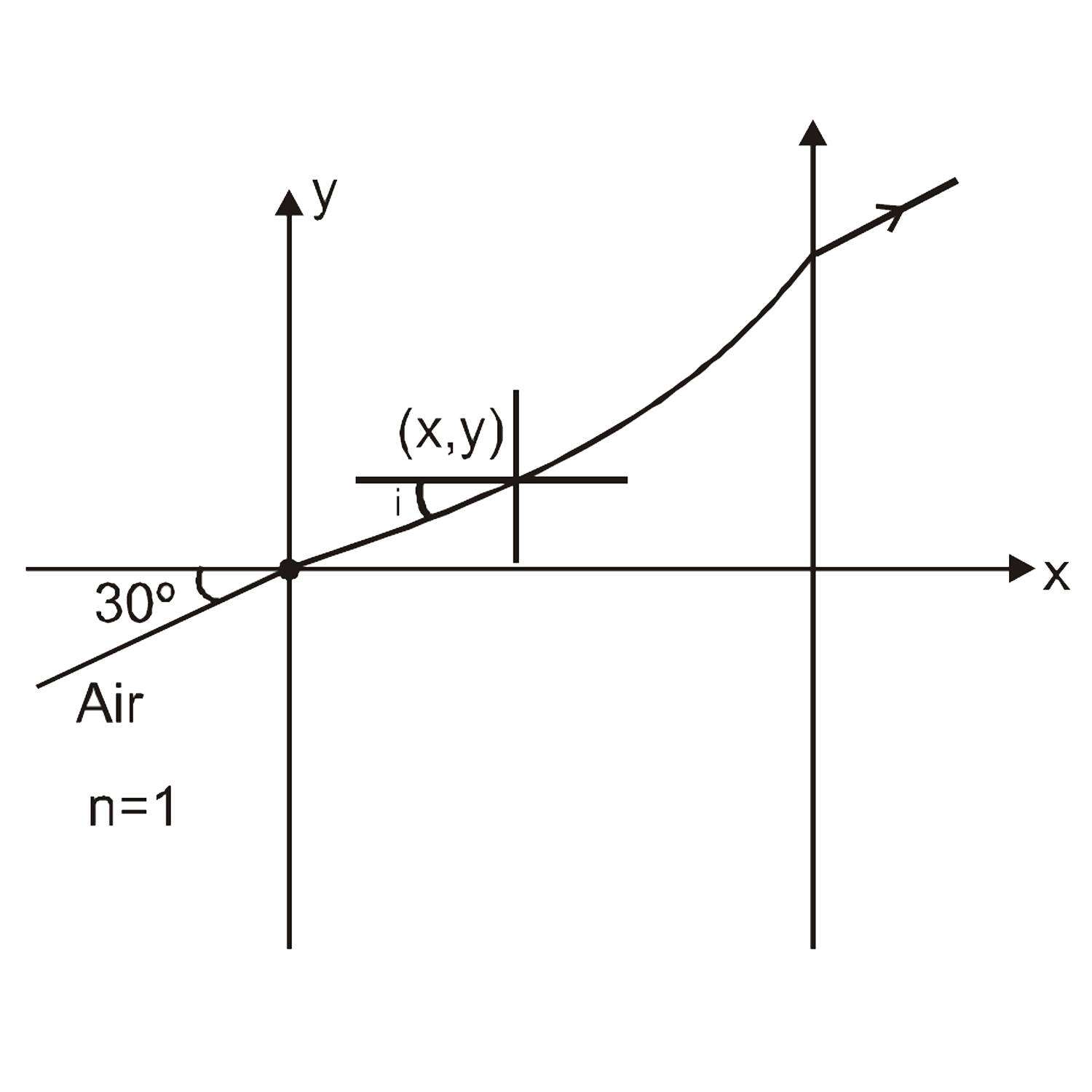
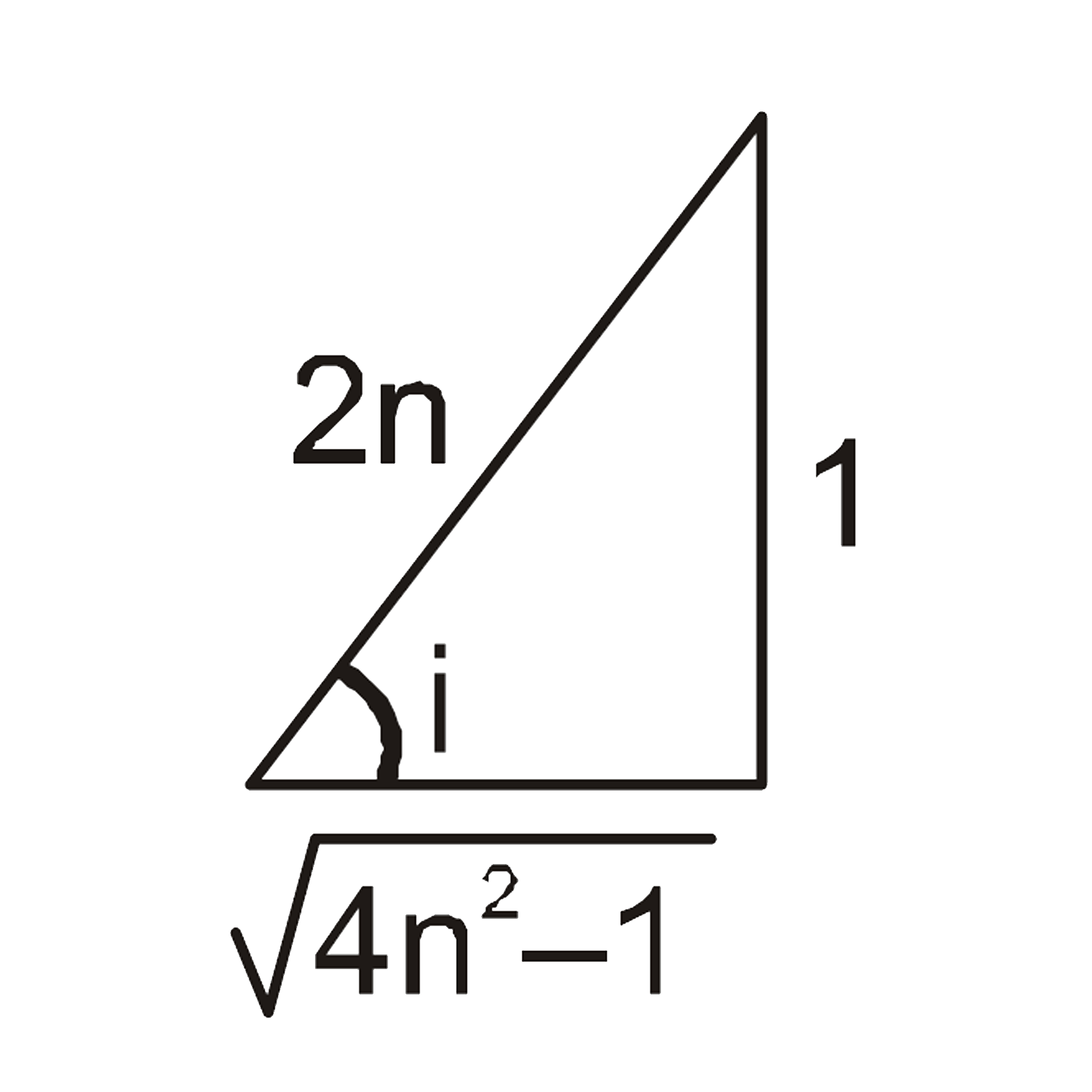
(a). 1×sin30∘=nsini
sini=12n
tani=1√4n2−1
dydx=1√x+3
∫y0dy=∫x0(x+3)−1/2dx
y=2(√1+3−√3) y=2(2−√3)
∴ position at which ray comes out of the medium is (1,2(2−√3))
Topper's Solved these Questions
Similar Questions
A system of coordinatees is drawn in a medium whose refractive index vaires as μ=21+Y2 where 0≤y≤1. A ray of light is incident at origin at an angle 60∘ with y-axis as shown in figure. At point P, the ray becomes parallel to x-axis, the volue of H is -
 View Solution
View SolutionThe area bounded by y=||x|−1| with the x - axis from x=0 to x=1 is k square units, then 4k is equal to
View Solution
Knowledge Check
- Question 1 - Select One
if x0=3andxn+1=√4+xn, then x3=
A2.65B2.58C2.56D2.55 - Question 2 - Select One
If x0=3 and xn+1=xn√xn+1 , then x3=
A15.9B31.7C44.9D65.2 - Question 3 - Select One
If x0=1 and xn+1=3√2xn,thenx_3 =
A1.26B1.361C1.396D1.408
If the tangent to the curve xy+ax+by=0 at (1,1) is inclined at an angle tan−12 with x-axis, then find aandb?
View SolutionLight of wavelength λ0 in air enters a medium of refractive index n. If two points A and B in this medium lie along the path of this light at a distance x, then phase difference ϕ0 between these two point is
View SolutionA ray of light, travelling in a medium of refractive index 'mu_, is incident at an angle i on a composite transparent plate consisting of three plates of refractive indices μ1,μ2andμ3. The ray emerges from the composite plate into a medium of refractive index μ4, at angle x. Then,
View SolutionA ray of light passes from vaccum into a medium of refractive index n. If the angle of incidence is twice the angle of refraction, then the angle of incidence is
View SolutionFind the area bounded by the curve y=cosx, x-axis and the ordinates x=0 and x=2π.
View Solution

