Find the distance travelled by a body having velocity from t = 0 to t = 2 sec .
The correct Answer is:B
To find the distance traveled by a body with a velocity function v(t)=1−t2 from t=0 to t=2 seconds, we will follow these steps:
Step 1: Set up the integral for distance
The distance s traveled by the body can be calculated using the integral of the velocity function over the given time interval. The formula for distance is:
s=∫t2t1v(t)dt
In our case, t1=0 and t2=2. Thus, we have:
s=∫20(1−t2)dt
Step 2: Evaluate the integral
Now, we will evaluate the integral:
s=∫20(1−t2)dt=∫201dt−∫20t2dt
Calculating each part separately:
1. The integral of 1 from 0 to 2:
∫201dt=(t)20=2−0=2
2. The integral of t2 from 0 to 2:
∫20t2dt=(t33)20=233−033=83−0=83
Step 3: Combine the results
Now we can combine the results of the two integrals:
s=2−83
To combine these, we need a common denominator:
s=63−83=6−83=−23
Step 4: Interpret the result
Since distance cannot be negative, we need to check the velocity function over the interval (0,2). The velocity v(t)=1−t2 becomes zero when t=1 (i.e., v(1)=0).
This means the body travels forward until t=1 and then reverses direction from t=1 to t=2. Therefore, we need to calculate the distance traveled in both segments:
1. From t=0 to t=1:
s1=∫10(1−t2)dt
Calculating this:
s1=∫10(1−t2)dt=(t−t33)10=(1−13)=23
2. From t=1 to t=2:
s2=∫21(1−t2)dt=(t−t33)21
Calculating this:
s2=(2−83)−(1−13)=(2−83)−(1−13)
Calculating s2:
s2=(2−83)−(33−13)=(63−83)−(23)=(−23)−(23)=−43
Final Distance Calculation
Thus, the total distance traveled is:
Total Distance=s1+|s2|=23+43=2 meters
Conclusion
The distance traveled by the body from t=0 to t=2 seconds is 2 meters.
---
The distance s traveled by the body can be calculated using the integral of the velocity function over the given time interval. The formula for distance is:
Now, we will evaluate the integral:
Now we can combine the results of the two integrals:
Since distance cannot be negative, we need to check the velocity function over the interval (0,2). The velocity v(t)=1−t2 becomes zero when t=1 (i.e., v(1)=0).
Thus, the total distance traveled is:
The distance traveled by the body from t=0 to t=2 seconds is 2 meters.
Topper's Solved these Questions
- TEST PAPERSBook:RESONANCE ENGLISHChapter:TEST PAPERSExercise:PART - II PHYSICS
Explore 106 Videos
- SIMPLE HARMONIC MOTION Book:RESONANCE ENGLISHChapter:SIMPLE HARMONIC MOTION Exercise:Advanced Level Problems
Explore 13 Videos
- TEST SERIESBook:RESONANCE ENGLISHChapter:TEST SERIESExercise:PHYSICS
Explore 130 Videos
Similar Questions
The distance travelled by a body moving along a line in time t is proportional to t3. The acceleration - time (a, t) graph for the motion of the body will be
View SolutionVelocity time equation of a particle moving in a straight line is v=2t−4 for t≤2s and v=4−2t for t>2.The distance travelled by the particle in the time interval from t=0 to t=4s is (Here t is in second and v in m/s)
View SolutionThe distance travelled by an object along the axes are given by x=2t2,y=t2−4t,z=3t−5. The initial velocity of the particle is .
View SolutionPosition of a point object is given by x=23t3−4t2+6t+7 (x is in meter and t is in seconds). What would be the distance travelled by object from t = 0 to instant when particle its direction of velocity for last time.
View SolutionA particle starts sliding down a frictionless inclined plane. If Sn is the distance travelled by it from time t=n−1sec, to t=nsec, the ratio Snsn+1 is
View SolutionA particle starts sliding down a frictionless inclined plane. If Sn is the distance travelled by it from time t=n−1sec, to t=nsec, the ratio Snsn+1 is
View SolutionIf x=at+bt2 , where x is the distance travelled by the body in kilometer while t is the time in seconds , then find the units of b.
View SolutionIn the given v-t graph, the distance travelled by the body in 5 sec will be :
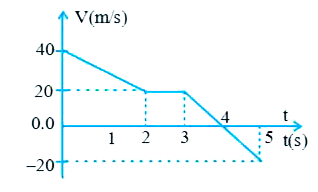
View Solutionbody moves along x-axis with velocity v = 5 sin(2t)m/s starting from xi=−2.5mRatio of displacement to distance traveled by body from t=0stot=3π/2sec is :
View SolutionThe velocity 'v' of a particle moving along straight line is given in terms of time t as v=3(t2−t) where t is in seconds and v is in m/s.
The distance travelled by particle from t=0 to t=2seconds is :
View Solution
