The following sets of values for Cv and Cp of an ideal gas have been reported by different students. The units are cal mole−1K−1. Which of these sets is most reliable?
Cv=3,Cp=5
Cv=4,Cp=6
Cv=3,Cp=2
Cv=3,Cp=4.2
The correct Answer is:A, B
To determine which set of values for Cp and Cv of an ideal gas is most reliable, we can use the relationship between Cp, Cv, and the universal gas constant R. The relationship is given by:
Cp−Cv=R
1. Identify the value of R:
The universal gas constant R is given as 8.314Jmol−1K−1. We need to convert this value into calories since the given values for Cp and Cv are in calories.
2. Convert R to calories:
We know that 1cal=4.186J. Therefore, to convert R from joules to calories, we divide by 4.186:
R=8.314Jmol−1K−14.186J/cal≈1.98calmol−1K−1≈2calmol−1K−1
3. Apply the relationship Cp−Cv=R:
Now that we have R≈2calmol−1K−1, we can check each set of values for Cp and Cv to see if they satisfy the equation Cp−Cv=2.
4. Evaluate each set:
- Set 1: Cp=5, Cv=3
Cp−Cv=5−3=2(Valid)
- Set 2: Cp=6, Cv=4
Cp−Cv=6−4=2(Valid)
- Set 3: Cp=2, Cv=3
Cp−Cv=2−3=−1(Invalid)
- Set 4: Cp=4.2, Cv=3
Cp−Cv=4.2−3=1.2(Invalid)
5. Conclusion:
The most reliable sets are Set 1 and Set 2, as they both satisfy the equation Cp−Cv=2.
The universal gas constant R is given as 8.314Jmol−1K−1. We need to convert this value into calories since the given values for Cp and Cv are in calories.
We know that 1cal=4.186J. Therefore, to convert R from joules to calories, we divide by 4.186:
Now that we have R≈2calmol−1K−1, we can check each set of values for Cp and Cv to see if they satisfy the equation Cp−Cv=2.
- Set 1: Cp=5, Cv=3
Cp−Cv=5−3=2(Valid)
- Set 2: Cp=6, Cv=4
Cp−Cv=6−4=2(Valid)
- Set 3: Cp=2, Cv=3
Cp−Cv=2−3=−1(Invalid)
- Set 4: Cp=4.2, Cv=3
Cp−Cv=4.2−3=1.2(Invalid)
The most reliable sets are Set 1 and Set 2, as they both satisfy the equation Cp−Cv=2.
Topper's Solved these Questions
- KTG & THERMODYNAMICSBook:RESONANCE ENGLISHChapter:KTG & THERMODYNAMICSExercise:PART -IV
Explore 9 Videos
- KTG & THERMODYNAMICSBook:RESONANCE ENGLISHChapter:KTG & THERMODYNAMICSExercise:Exercise-3
Explore 1 Video
- KTG & THERMODYNAMICSBook:RESONANCE ENGLISHChapter:KTG & THERMODYNAMICSExercise:PART -I
Explore 15 Videos
- KINETIC THEORY OF GASES AND THERMODYNAMICSBook:RESONANCE ENGLISHChapter:KINETIC THEORY OF GASES AND THERMODYNAMICSExercise:Exercise
Explore 64 Videos
- MAGNETIC FIELD AND FORCESBook:RESONANCE ENGLISHChapter:MAGNETIC FIELD AND FORCESExercise:Exercise
Explore 64 Videos
Similar Questions
V vs T curves at different pressures P1 and P2 for an ideal gas are shown below:
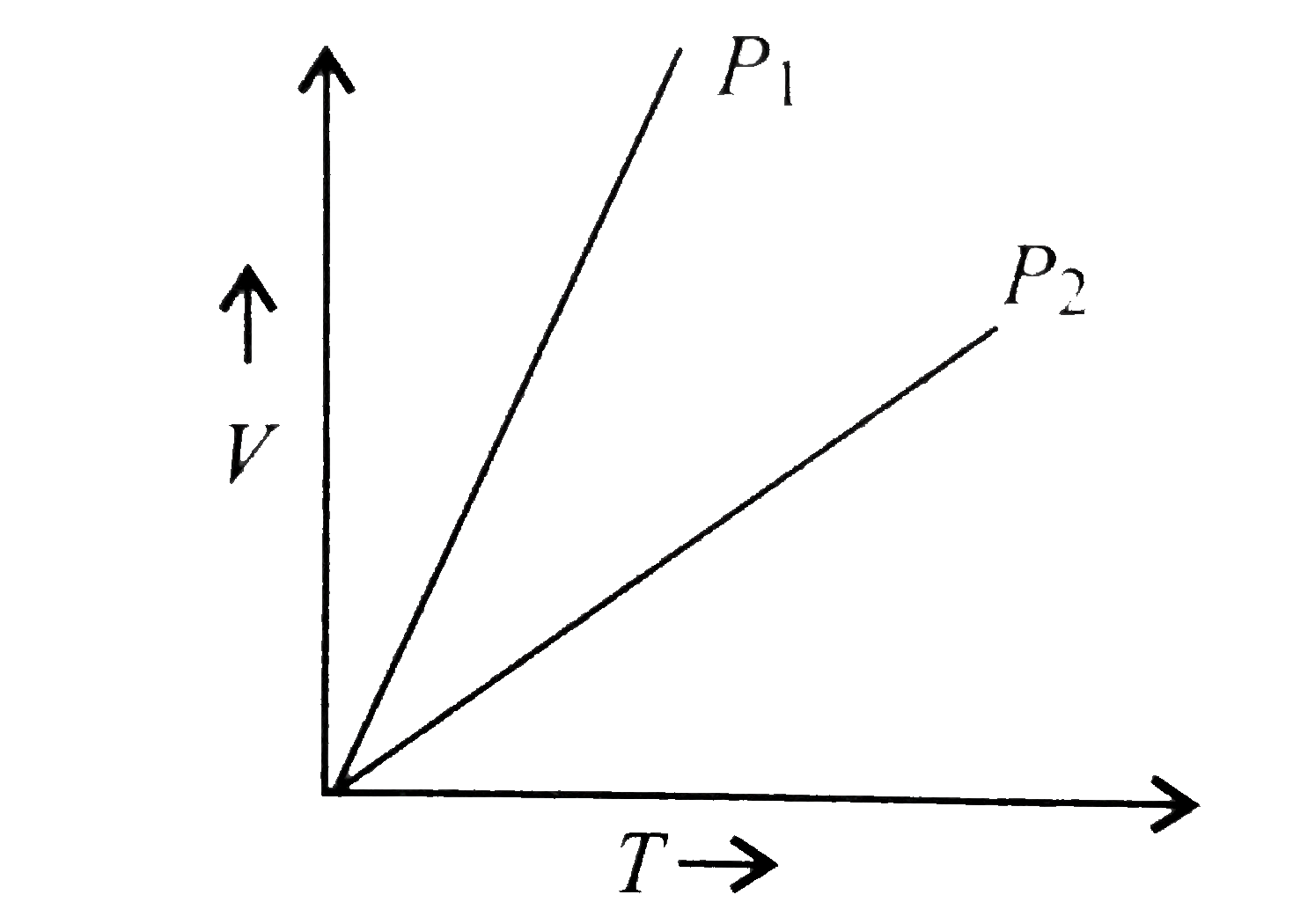
Which one of the following is correct?
View SolutionThe molar specific heats of an ideal gas at constant volume and constant pressure are respectively 4.98 and 6.96 cal mol−1K−1. If the molecular weight of the gas be 32, then calculate the root means square speed of the molecule of the gas at 120∘C. (1 cal = 4.2 J)
View Solution
Knowledge Check
The kinetic enerrgy of 4 mole sof nitrogen gas at 127∘C is (R=2 cal mol−1K−1)
A4400 calB3200 calC4800 calD1524 cal
The specific heats, CP and CV of a gas of diatomic molecules, A are given (in units of Jmol−1K−1) by 29 and 22 , respectively. Another gas of diatomic molecules , B has the corresponding values 30 and 21. if they are treated as ideal gases, then :
View SolutionWhen 5 moles of an ideal gas is expended at 350 K reversibly from 5 L to 40 L, then the entropy change for the process is (R = 2 cal mol−1K−1)
View SolutionLet (Cv)and(Cp) denote the molar heat capacities of an ideal gas at constant volume and constant pressure respectively . Which of the following is a universal constant?
View Solution1 mol of an ideal gas undergoes different thermodynamical process in P−V digram given below :

If temperature at point C is TK then :
View SolutionOne mole of an ideal gas at 300K is expanded isothermally from an inital volume of 1 litre to 10 litres. The ΔE for this process is (R=2calmol−1K−1)
View SolutionThe molar heat capacity of a gas at constant volume is found to be 5calmol−1K−1. Find the ratioγ=CpCv for the gas. The gas constant R=2 cal mol^(-1) K^(-1).
View SolutionIf CpandCv denote the specific heats (per unit mass of an ideal gas of molecular weight M), then
where R is the molar gas constant.
View Solution