An ideal gas (CpCv=γ)has initial volume V0 is kept in a vessel. It undergoes a change and follows the following relation P=kV2 (where P is pressure, and V is volume) find the change in internal energy of the gas if its final pressure is P0:
The correct Answer is:kγ−1((P0k)3/2−V30)
To solve the problem, we will follow a systematic approach to find the change in internal energy of the ideal gas under the given conditions.
1. Understanding the Relationship Between Cp and Cv:
We know that for an ideal gas, the ratio of specific heats is given by:
CpCv=γ
We also have the relation:
Cp=Cv+R
From this, we can express Cv in terms of R and γ:
γ=Cv+RCv⟹Cv(γ−1)=R⟹Cv=Rγ−1
2. Internal Energy Formula:
The change in internal energy ΔU for an ideal gas is given by:
ΔU=nCvΔT
Substituting the expression for Cv:
ΔU=n(Rγ−1)ΔT
3. Using the Ideal Gas Law:
The ideal gas law states:
PV=nRT
Rearranging gives us:
nR=PVT
For a change, we can express this as:
Δ(PV)=nRΔT
4. Relating Pressure and Volume:
From the problem, we know that:
P=kV2
Therefore, we can express the change in PV as:
Δ(PV)=PfVf−PiVi
where Pf and Pi are the final and initial pressures, and Vf and Vi are the final and initial volumes.
5. Substituting Values:
Given that the final pressure Pf=P0 and the initial pressure Pi=kV20, we can write:
Vf=√P0k
Therefore, substituting these values into the equation for ΔU:
ΔU=Δ(PV)γ−1=PfVf−PiViγ−1
6. Calculating ΔU:
Now substituting the values:
ΔU=P0√P0k−kV20V0γ−1
Simplifying this gives:
ΔU=P3/20/√k−kV30γ−1
Final Expression:
Thus, the change in internal energy of the gas is:
ΔU=P3/20/√k−kV30γ−1
We know that for an ideal gas, the ratio of specific heats is given by:
CpCv=γ
We also have the relation:
Cp=Cv+R
From this, we can express Cv in terms of R and γ:
γ=Cv+RCv⟹Cv(γ−1)=R⟹Cv=Rγ−1
The change in internal energy ΔU for an ideal gas is given by:
ΔU=nCvΔT
Substituting the expression for Cv:
ΔU=n(Rγ−1)ΔT
The ideal gas law states:
PV=nRT
Rearranging gives us:
nR=PVT
For a change, we can express this as:
Δ(PV)=nRΔT
From the problem, we know that:
P=kV2
Therefore, we can express the change in PV as:
Δ(PV)=PfVf−PiVi
where Pf and Pi are the final and initial pressures, and Vf and Vi are the final and initial volumes.
Given that the final pressure Pf=P0 and the initial pressure Pi=kV20, we can write:
Vf=√P0k
Therefore, substituting these values into the equation for ΔU:
ΔU=Δ(PV)γ−1=PfVf−PiViγ−1
Now substituting the values:
ΔU=P0√P0k−kV20V0γ−1
Simplifying this gives:
ΔU=P3/20/√k−kV30γ−1
Thus, the change in internal energy of the gas is:
ΔU=P3/20/√k−kV30γ−1
Topper's Solved these Questions
- KTG & THERMODYNAMICSBook:RESONANCE ENGLISHChapter:KTG & THERMODYNAMICSExercise:Advancel Level Problems
Explore 1 Video
- KINETIC THEORY OF GASES AND THERMODYNAMICSBook:RESONANCE ENGLISHChapter:KINETIC THEORY OF GASES AND THERMODYNAMICSExercise:Exercise
Explore 64 Videos
- MAGNETIC FIELD AND FORCESBook:RESONANCE ENGLISHChapter:MAGNETIC FIELD AND FORCESExercise:Exercise
Explore 64 Videos
Similar Questions
The pressure p and volume V of an ideal gas both increase in a process.
View SolutionThe pressure P and volume V of an ideal gas both decreases in a process.
View SolutionOne mole of an ideal monotomic gas undergoes a linear process from A to B in which its pressure p and its volume V change as shown in figure.
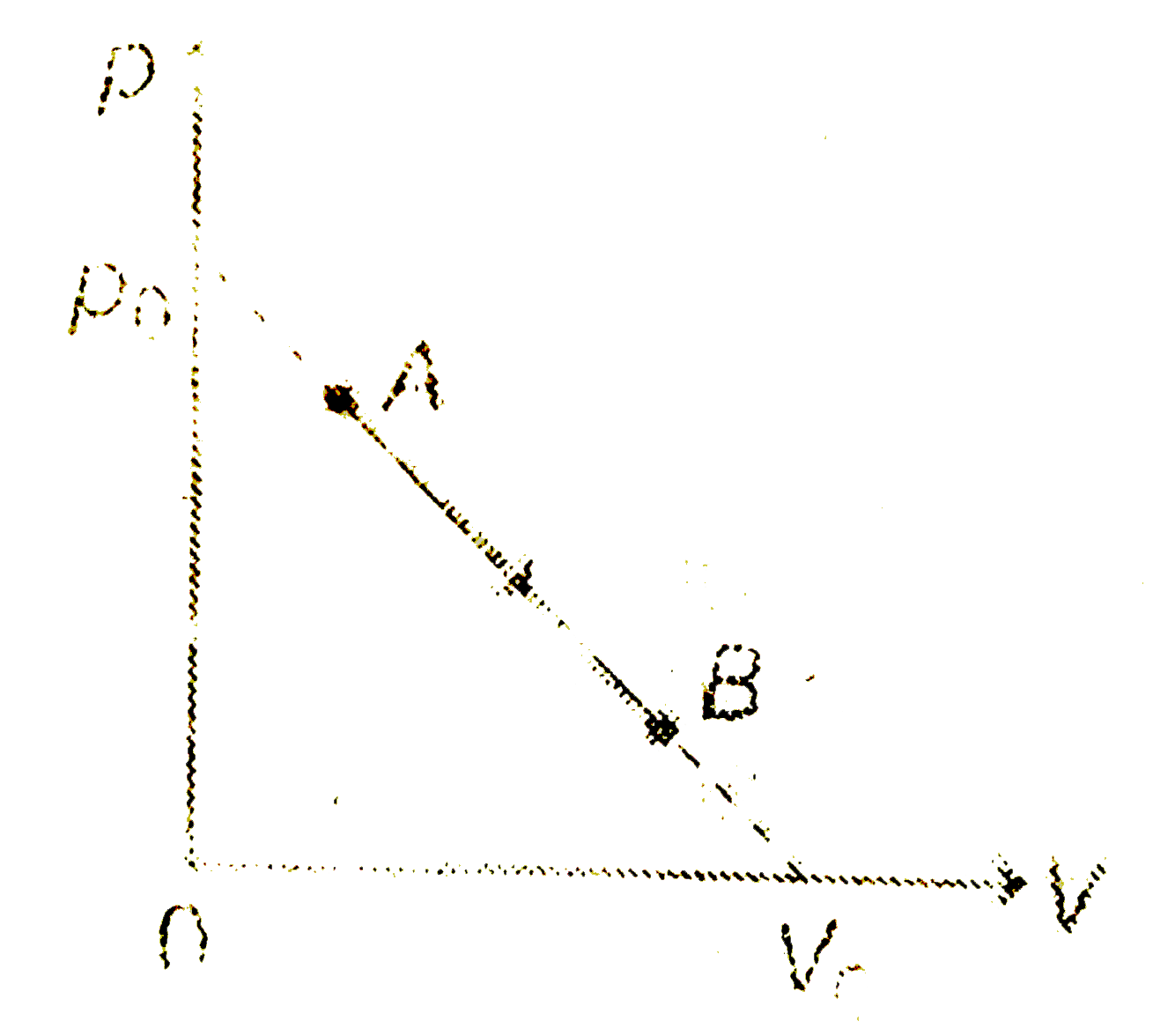
The maximum temperature of the gas during this process is
View SolutionThe energy density uV of an ideal gas is related to its pressure P as
View SolutionUnit mass of liquid of volume V1 completely turns into a gas of volume V2 at constant atmospheric pressure P and temperature T. The latent heat of vaporization is "L". Then the change in internal energy of the gas is
View SolutionAn ideal gas (Cp/Cv=γ) having initial pressure P0 and volume V0 (a) The gas is taken isothermally to a pressure 2P0 and then adiabatically to a pressure 4P0. Find the final volume. (b) The gas is brought back to its initial state. It is adiabatically taken to a pressure 2P0 and then isothermally to a pressure 4P0. Find the final volume.
View SolutionIf the ratio of specific heat of a gas of constant pressure to that at constant volume is γ, the change in internal energy of the mass of gas, when the volume changes from V to 2V at constant pressure p is
View SolutionTwo moles of a gas at temperature T and volume V are heated to twice its volume at constant pressure. If CpCv=γ then increase in internal energy of the gas is-
View SolutionOne mole of an ideal gas passes through a process where pressure and volume obey the relation P=P0[1−12(V0V)2] Here P0 and V0 are constants. Calculate the change in the temperature of the gas if its volume changes from V0 to 2V0.
View SolutionOne mole of an ideal monatomic gas undergoes a linear process from A to B , in which is pressure P and its volume V change as shown in figure .
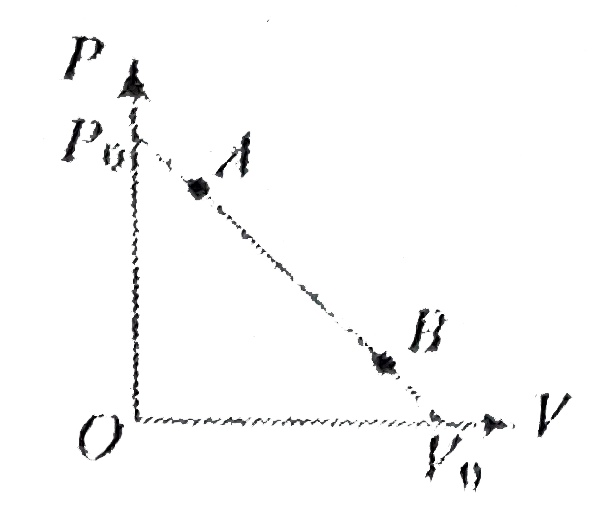
The absolute temperature T versus volume V for the given process is
View Solution