The ratio of thermal conductivites of two different metals is 5:3. In order to have the same thermal resistance in these metals of equal thickness what should be the ratio of their length?
To solve the problem step by step, we will use the relationship between thermal resistance, thermal conductivity, length, and cross-sectional area.
Step 1: Understand the formula for thermal resistance
The thermal resistance R of a material can be expressed using the formula:
R=LkA
where:
- R is the thermal resistance,
- L is the length of the material,
- k is the thermal conductivity,
- A is the cross-sectional area.
Step 2: Write the expressions for thermal resistance for both metals
Let’s denote the two metals as Metal 1 and Metal 2. The thermal resistances for the two metals can be expressed as:
R1=L1K1A
R2=L2K2A
where K1 and K2 are the thermal conductivities of Metal 1 and Metal 2, respectively.
Step 3: Set the thermal resistances equal
According to the problem, the thermal resistance of both metals is the same:
R1=R2
This implies:
L1K1A=L2K2A
Step 4: Simplify the equation
Since the cross-sectional area A is the same for both metals, we can cancel A from both sides:
L1K1=L2K2
Step 5: Rearrange to find the ratio of lengths
Rearranging the equation gives us:
L1L2=K1K2
Step 6: Substitute the given ratio of thermal conductivities
We know from the problem that the ratio of thermal conductivities K1:K2=5:3. Thus:
L1L2=53
Conclusion
The ratio of the lengths of the two metals should be:
L1:L2=5:3
The thermal resistance R of a material can be expressed using the formula:
R=LkA
where:
- R is the thermal resistance,
- L is the length of the material,
- k is the thermal conductivity,
- A is the cross-sectional area.
Let’s denote the two metals as Metal 1 and Metal 2. The thermal resistances for the two metals can be expressed as:
R1=L1K1A
R2=L2K2A
where K1 and K2 are the thermal conductivities of Metal 1 and Metal 2, respectively.
According to the problem, the thermal resistance of both metals is the same:
R1=R2
This implies:
L1K1A=L2K2A
Since the cross-sectional area A is the same for both metals, we can cancel A from both sides:
L1K1=L2K2
Rearranging the equation gives us:
L1L2=K1K2
We know from the problem that the ratio of thermal conductivities K1:K2=5:3. Thus:
L1L2=53
The ratio of the lengths of the two metals should be:
L1:L2=5:3
Topper's Solved these Questions
- HEAT TRANSFERBook:RESONANCE ENGLISHChapter:HEAT TRANSFERExercise:Exercise-1
Explore 1 Video
- HEAT TRANSFERBook:RESONANCE ENGLISHChapter:HEAT TRANSFERExercise:Exercise
Explore 91 Videos
- HEAT TRANSFERBook:RESONANCE ENGLISHChapter:HEAT TRANSFERExercise:Example
Explore 10 Videos
- GRAVITATIONBook:RESONANCE ENGLISHChapter:GRAVITATIONExercise:Exercise
Explore 21 Videos
- KINEMATICSBook:RESONANCE ENGLISHChapter:KINEMATICSExercise:Exercise
Explore 65 Videos
Similar Questions
The ratio of the thermal conductivities of two different materials is 1:2. The thermal resistance of the rods of these materials having the same thickness are equal. Find the ratio of the length of rods.
View SolutionThe ratio of thermal conductivity of two rods of different material is 5:4. The two rods of same area of cross-section and same thermal resistance will have the lengths in the ratio
View Solution
Knowledge Check
Two waves of the same pitch have amplitudes in the ratio 1 : 3. What will be the ratio of their :
frequencies?A1:9B9:1C1:1Dno change
Two waves of the same pitch have amplitudes in the ratio 1 : 3. What will be the ratio of their :
intensities and
View SolutionTwo waves of the same pitch have amplitudes in the ratio 1:3. What will be the ratio of their
loudness
View SolutionIf the radii of circular path of two particles are in the ratio of 1:2, then in order to have same centripetal acceleration, their speeds should be in the ratio of :
View SolutionThe ratio of the diameters of two metallic rods of the same material is 2:1 and their lengths are in the ratio 1:4. If the temperature difference between their ends are equal, the rate of flow of heat in them will be in the ratio
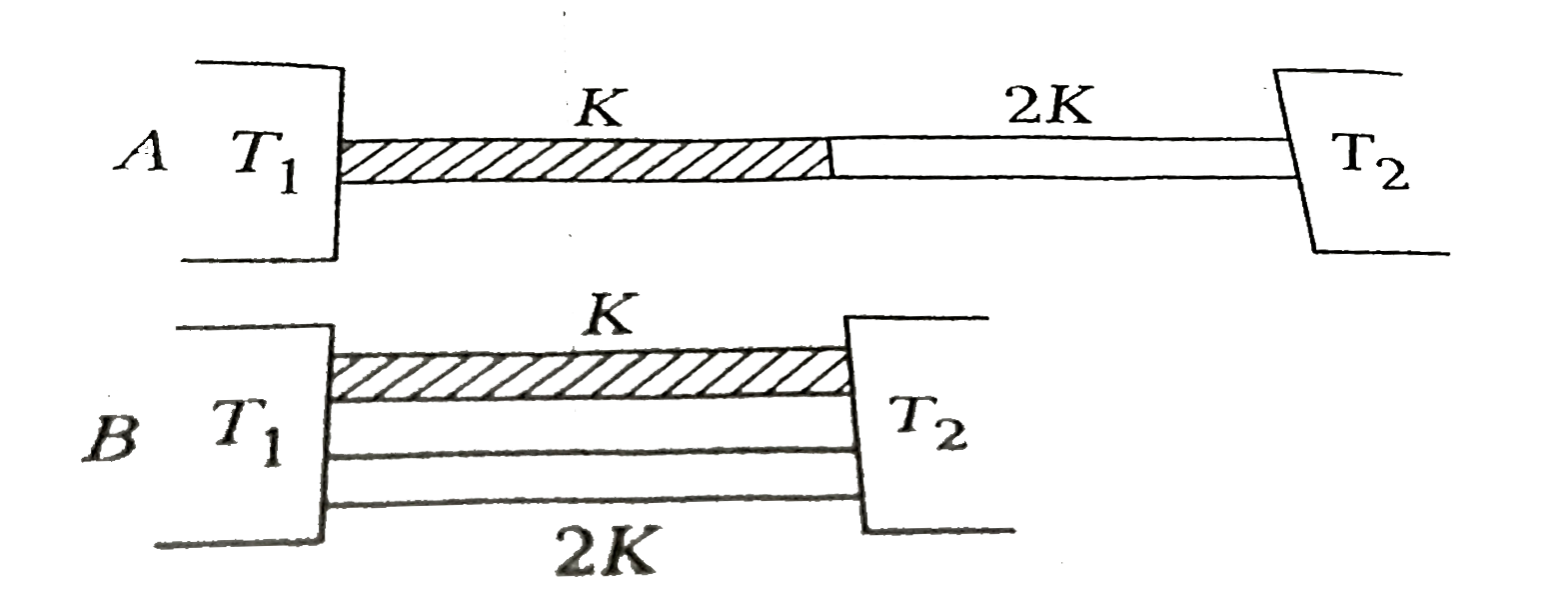
View SolutionTwo rods with the same dimensions have thermal conductivities in the ratio 1 : 2. they are arranged between heat reservoirs with the same temperature difference, in two different configurations, A and B. The rates of heat flow in A and B are IAandIB respectively. The ratio IAIB) is equal to
View SolutionThe ratio of the densities of the two bodies is 3:4 and the ratio of specific heats is 4:3 Find the ratio of their thermal capacities for unit volume?
View SolutionThe ratio of the densities of the two bodies is 3:4 and the ratio of specific heats is 4:3 Find the ratio of their thermal capacities for unit volume?
View Solution
