Define coefficient of thermal conductivity or thermal conductivity of a substance.
The correct Answer is:The coefficient of thermal conductivity of a substance is defined as the amount of heat that flows in one second across the opposite faces of a unit cube whose faces are kept at a temperature difference of 1∘C
Step-by-Step Text Solution:
1. Understanding Thermal Conductivity:
- Thermal conductivity refers to the ability of a material to conduct heat. It is a measure of how well heat is transferred through a substance.
2. Defining Coefficient of Thermal Conductivity:
- The coefficient of thermal conductivity (often denoted as k) is defined as the rate of heat transfer through a unit area of a material per unit temperature difference across that area.
3. Mathematical Expression:
- The coefficient of thermal conductivity can be expressed mathematically as:
k=QA⋅ΔT
where:
- k = coefficient of thermal conductivity
- Q = amount of heat transferred
- A = area through which heat is transferred
- ΔT = temperature difference across the material
4. Practical Example:
- Consider a cubical material with two faces (Phase 1 and Phase 2). When heat is supplied to Phase 1, it flows to Phase 2. The coefficient of thermal conductivity can be understood as the amount of heat required to raise the temperature of the material by 1 degree Celsius when the heat is transferred from Phase 1 to Phase 2.
5. Conclusion:
- In summary, the coefficient of thermal conductivity is a crucial property of materials that indicates how effectively they can conduct heat, which is essential in various applications in physics and engineering.
- Thermal conductivity refers to the ability of a material to conduct heat. It is a measure of how well heat is transferred through a substance.
- The coefficient of thermal conductivity (often denoted as k) is defined as the rate of heat transfer through a unit area of a material per unit temperature difference across that area.
- The coefficient of thermal conductivity can be expressed mathematically as:
k=QA⋅ΔT
where:
- k = coefficient of thermal conductivity
- Q = amount of heat transferred
- A = area through which heat is transferred
- ΔT = temperature difference across the material
- Consider a cubical material with two faces (Phase 1 and Phase 2). When heat is supplied to Phase 1, it flows to Phase 2. The coefficient of thermal conductivity can be understood as the amount of heat required to raise the temperature of the material by 1 degree Celsius when the heat is transferred from Phase 1 to Phase 2.
- In summary, the coefficient of thermal conductivity is a crucial property of materials that indicates how effectively they can conduct heat, which is essential in various applications in physics and engineering.
Topper's Solved these Questions
- HEAT TRANSFERBOOK - RESONANCE ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - Exercise-1
1 Videos
- HEAT TRANSFERBOOK - RESONANCE ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - Exercise
91 Videos
- HEAT TRANSFERBOOK - RESONANCE ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - Example
10 Videos
- GRAVITATIONBOOK - RESONANCE ENGLISHCHAPTER - GRAVITATIONEXERCISE - Exercise
21 Videos
- KINEMATICSBOOK - RESONANCE ENGLISHCHAPTER - KINEMATICSEXERCISE - Exercise
65 Videos
Similar Questions
SI unit of coefficient of thermal conductivity
View SolutionCoefficient of thermal conductivity has the dimensions:
View SolutionThe unit of thermal conductivity is :
View SolutionThe unit of thermal conductivity is :
View SolutionWrite the coefficient of thermal coonductivity of a perfect heat conductor and that of a perfect heat insulator.
View SolutionThe dimensions of coefficient of thermal conductivity is
View SolutionCoefficient of thermal conductivity is the product of heat, distance and reciprocal of (area x difference in temperature x time). The new value of a unit of coefficient of thermal conductivity, if fundamental units are 21.6 kg, 1 decimetre, 4 K and 1 minute will be ______ ×10−6 new units.
View SolutionDescribe an experiment to determine the coefficient of thermal conductivity of a good conductor by Searle's method.
View SolutionTwo vessels of different materials are identical in size and wall-thickness. They are filled with equal quantities of ice at 0∘C. If the ice melts completely in 10 and 25 minutes respectively, compare the coefficients of thermal conductivity of the materials of the vessels.
View Solution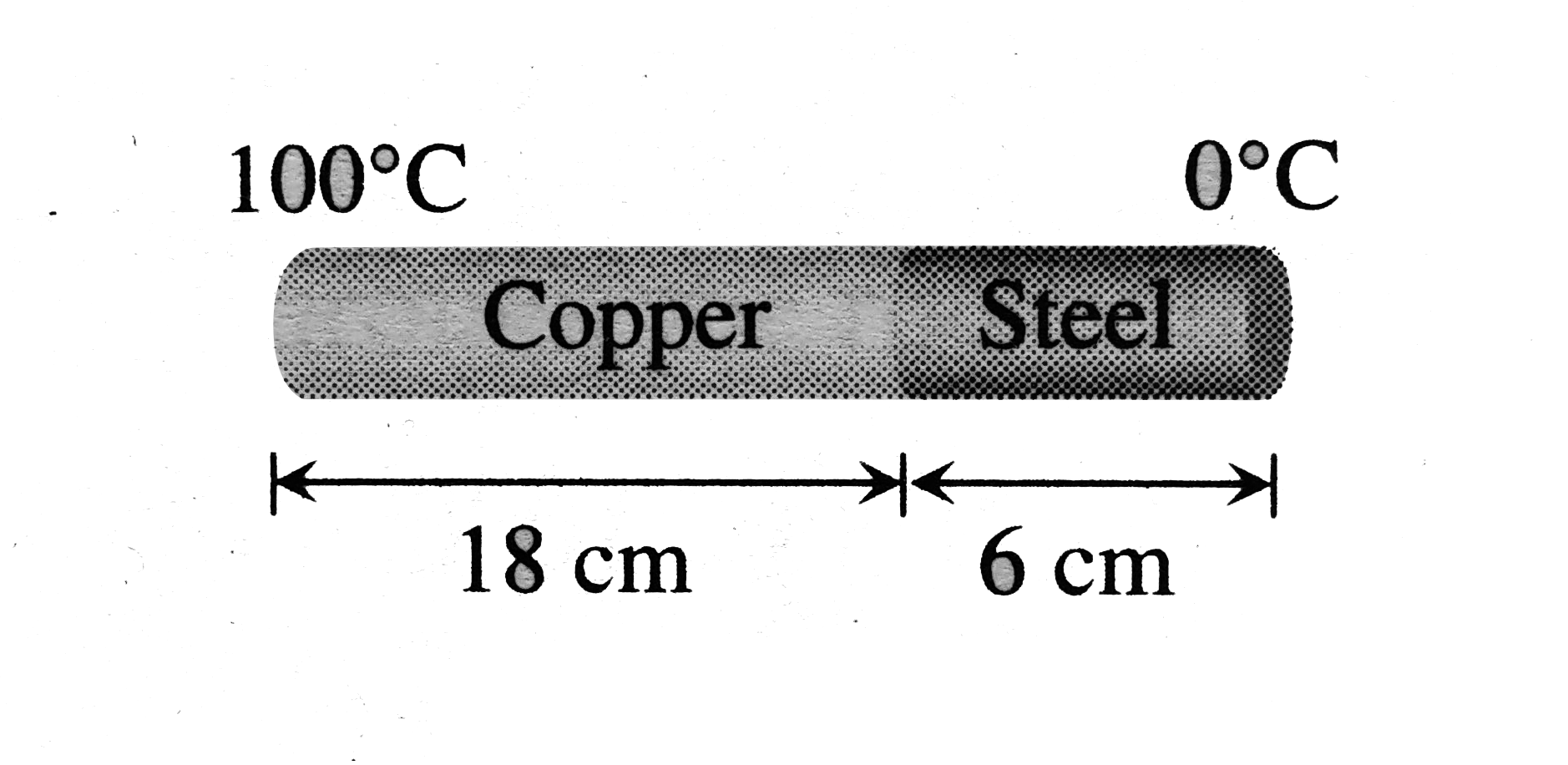
The coefficient of thermal conductivity of copper is nine times that of steel. In the composite cylindrical bar shown in Fig. what will be the temperature at the junction of copper and steel ?
View Solution
