A particle is dropped from a finite height H above the ground level under gravity . Due to air resistance acceleration of particle becomes a=g−kv in the direction of velocity. Where k= positive constant &v= speed of particle. Then which of the following graph(s) is / are possible (t0= time when particle touches the ground ):
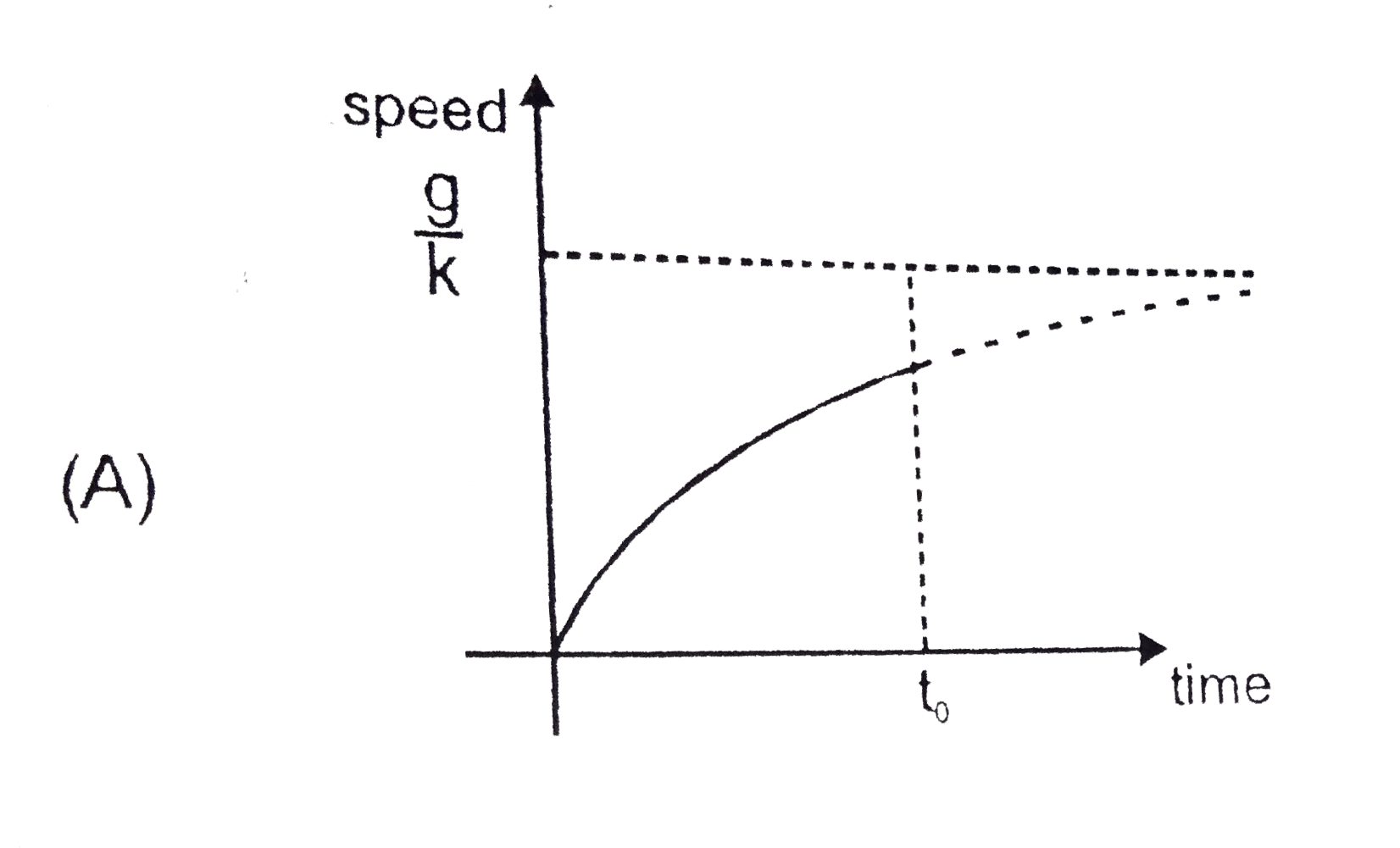
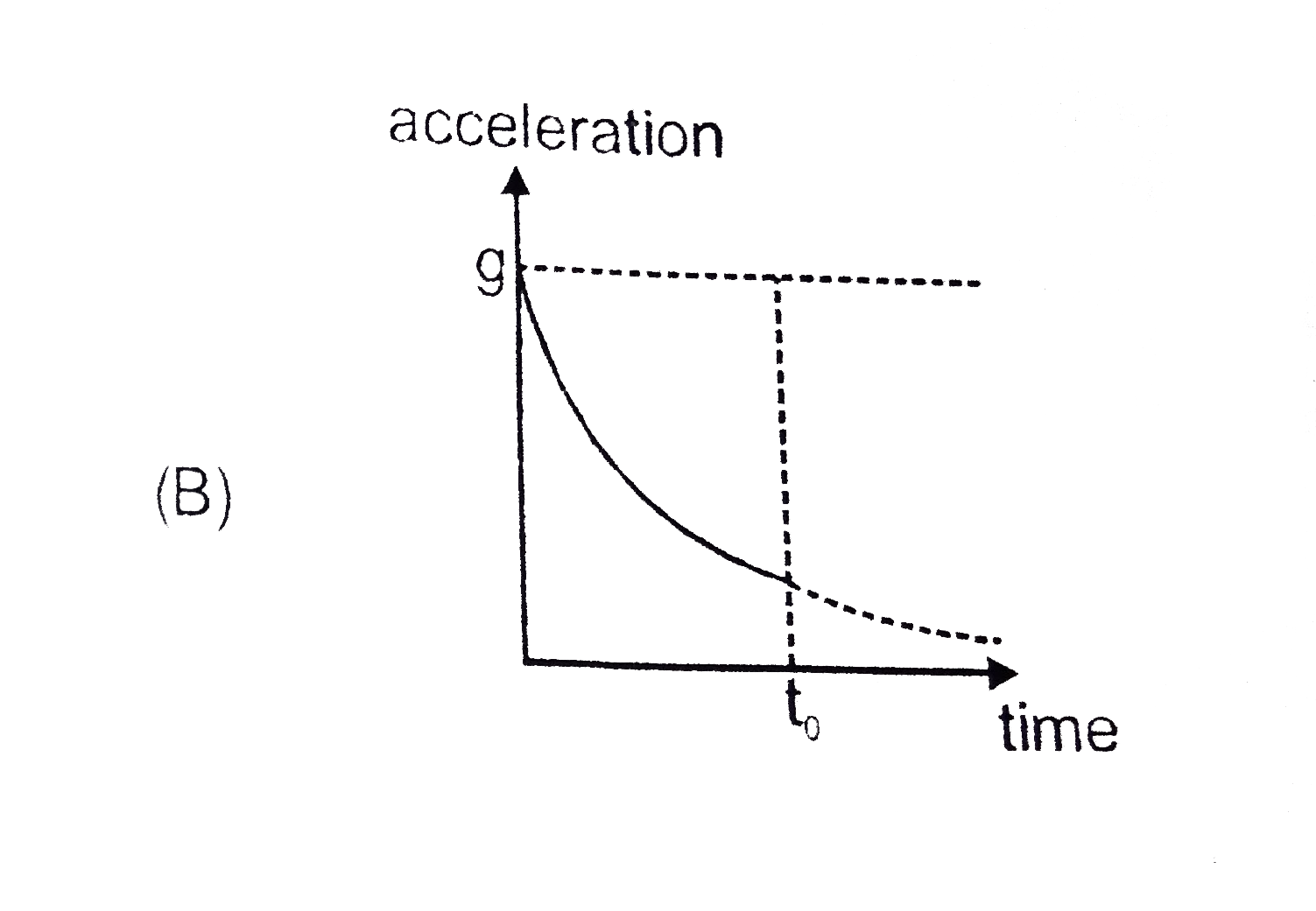
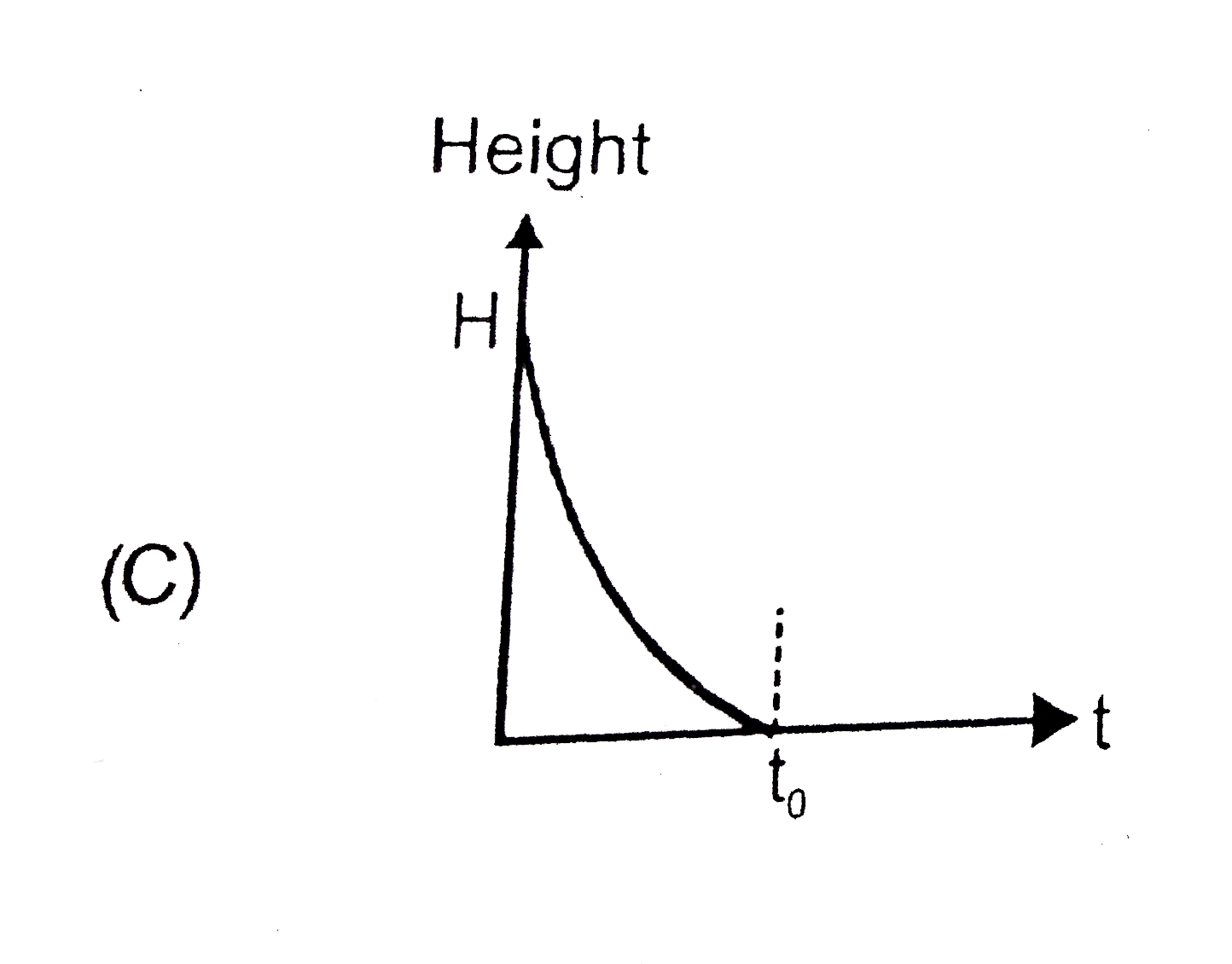
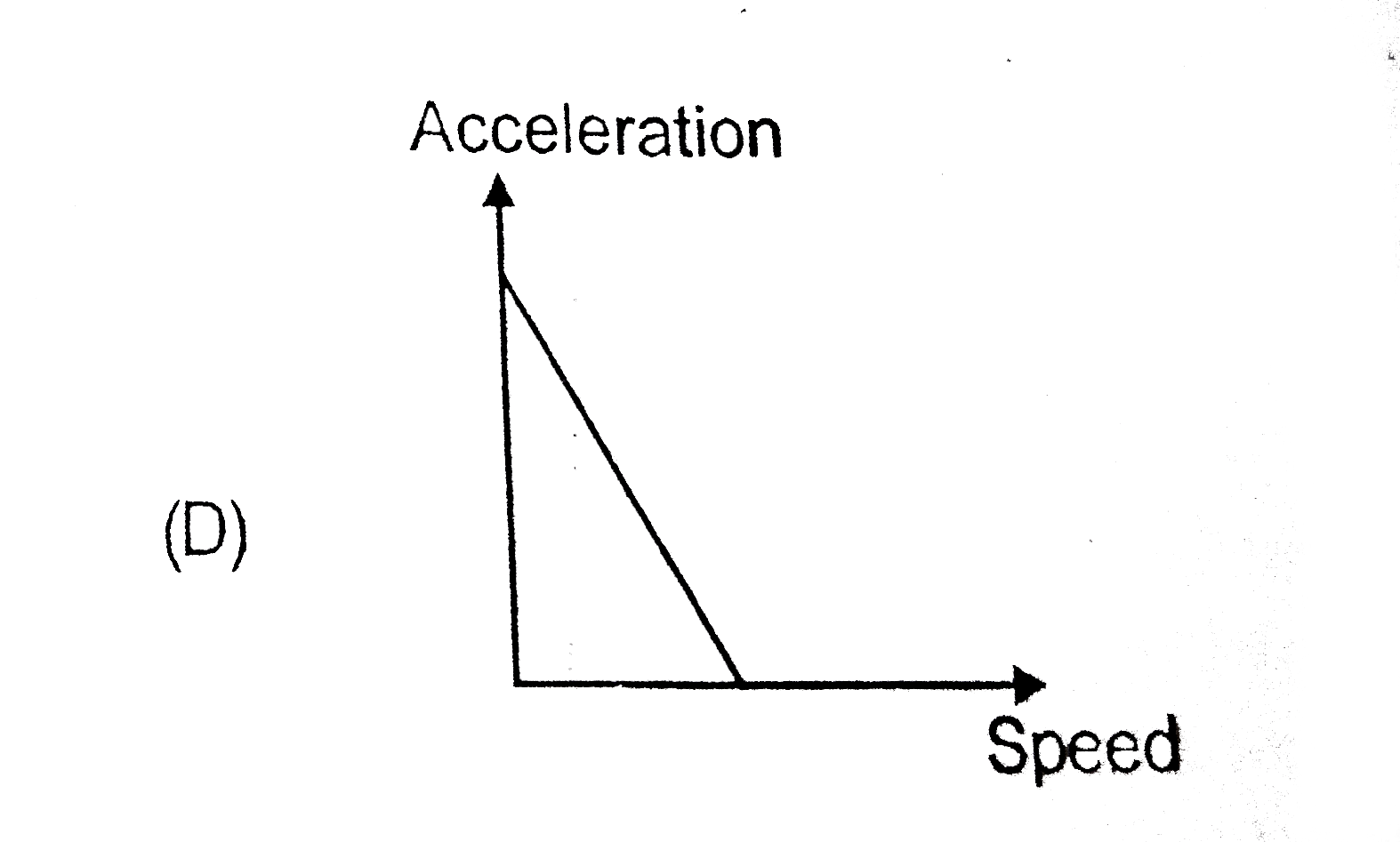
The correct Answer is:A, B, D
dvdt=g−kvv∫0dvg−kv=t∫t=0dt
−1−kvln(g−kvg)=t
g−kv=≥−ktv=gk[1−e−kt]
a=gk[0−e−kt(−k)]
=ge−kt
V=gk−ak=−ak+ak
V−gk=−ak
kv−g=−a
a=g−kv
=−kv+g
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMSBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 15
8 Videos
- DAILY PRACTICE PROBLEMSBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 16 physics
8 Videos
- DAILY PRACTICE PROBLEMSBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - Dpp no. 13 physics
5 Videos
- CURRENT ELECTRICITYBOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise
53 Videos
- ELASTICITY AND VISCOCITYBOOK - RESONANCE ENGLISHCHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Advanced Level Problems
9 Videos
Similar Questions
Particle is dropped form the height of 20m from horizontal ground. There is wind blowing due to which horizontal acceleration of the particles becomes 6ms−2. Find the horizontal displacement of the particle till it reaches ground.
View SolutionA particle is thrown vertically upwards with a velocity v. It returns to the ground in time T. which of the following graphs correctly represents the motion ?
View Solution
Knowledge Check
Particle is dropped form the height of 20m form horizontal ground. There is wind blowing due to which horizontal acceleration of the particles becomes 6ms−2. Find the horizontal displacement of the particle till it reaches ground.
A6mB10mC12mD24mA particle is thrown vertically upwards with a velocity v. It returns to the ground in time T. which of the following graphs correctly represents the motion ?
A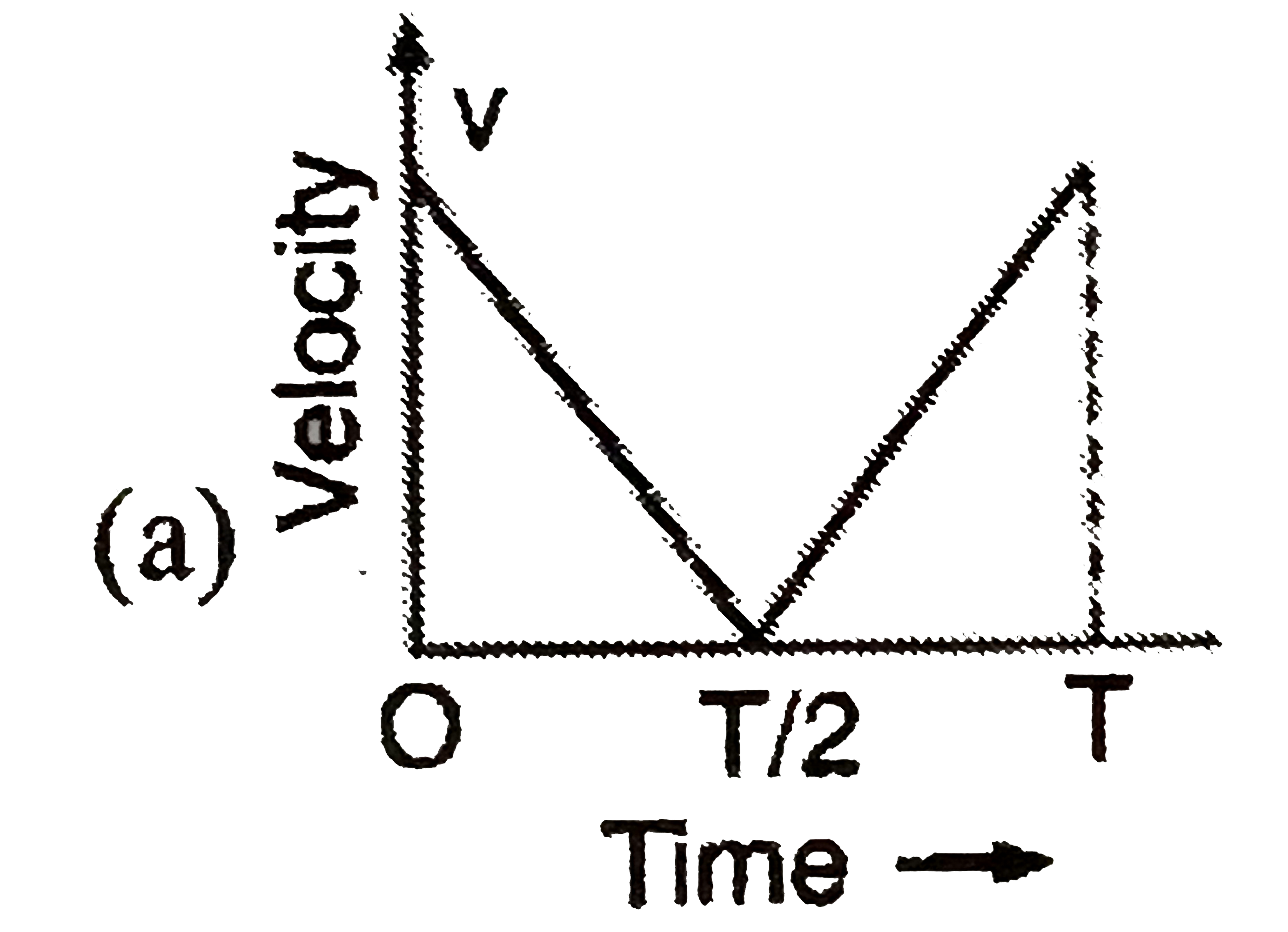 B
B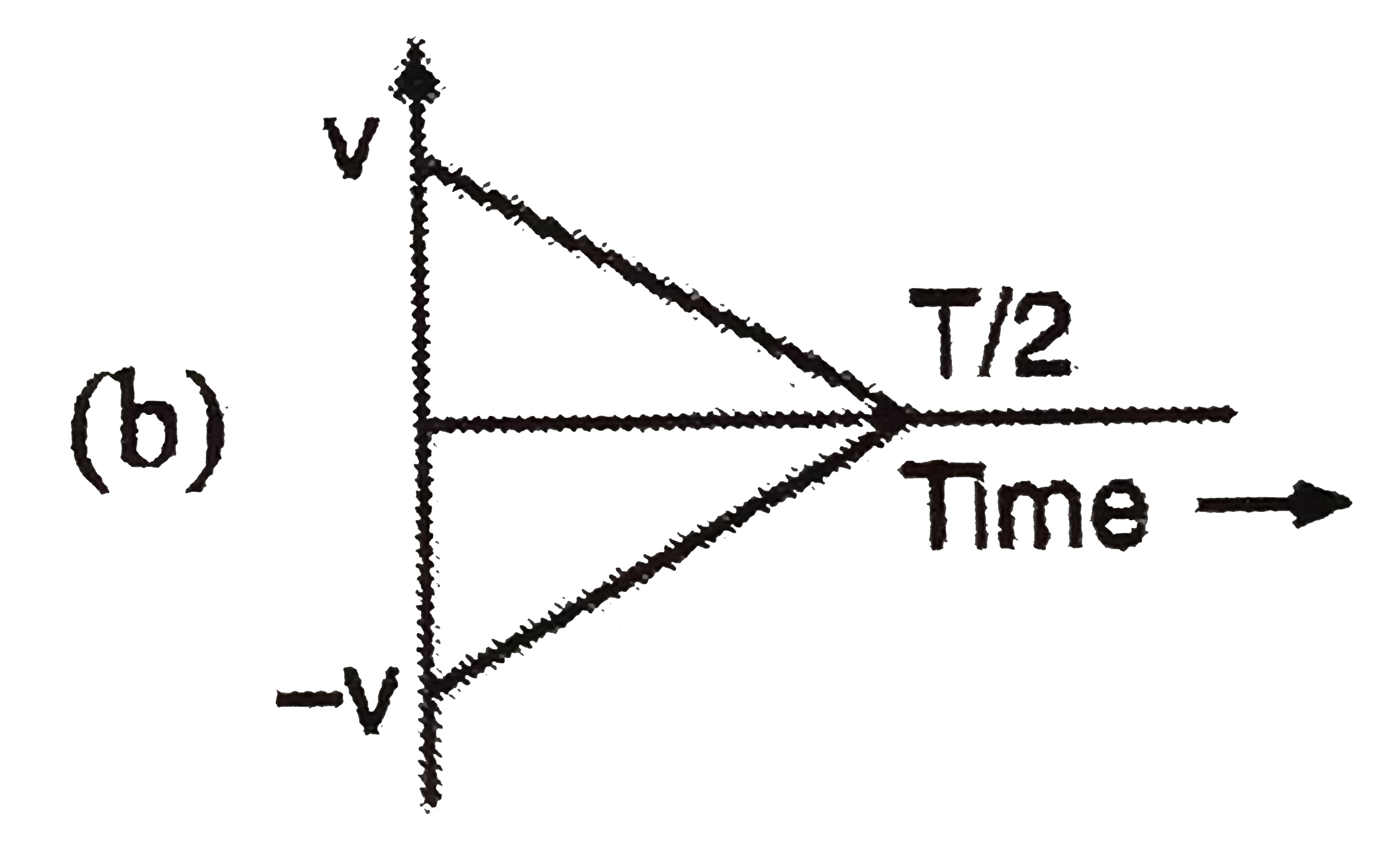 C
C D
D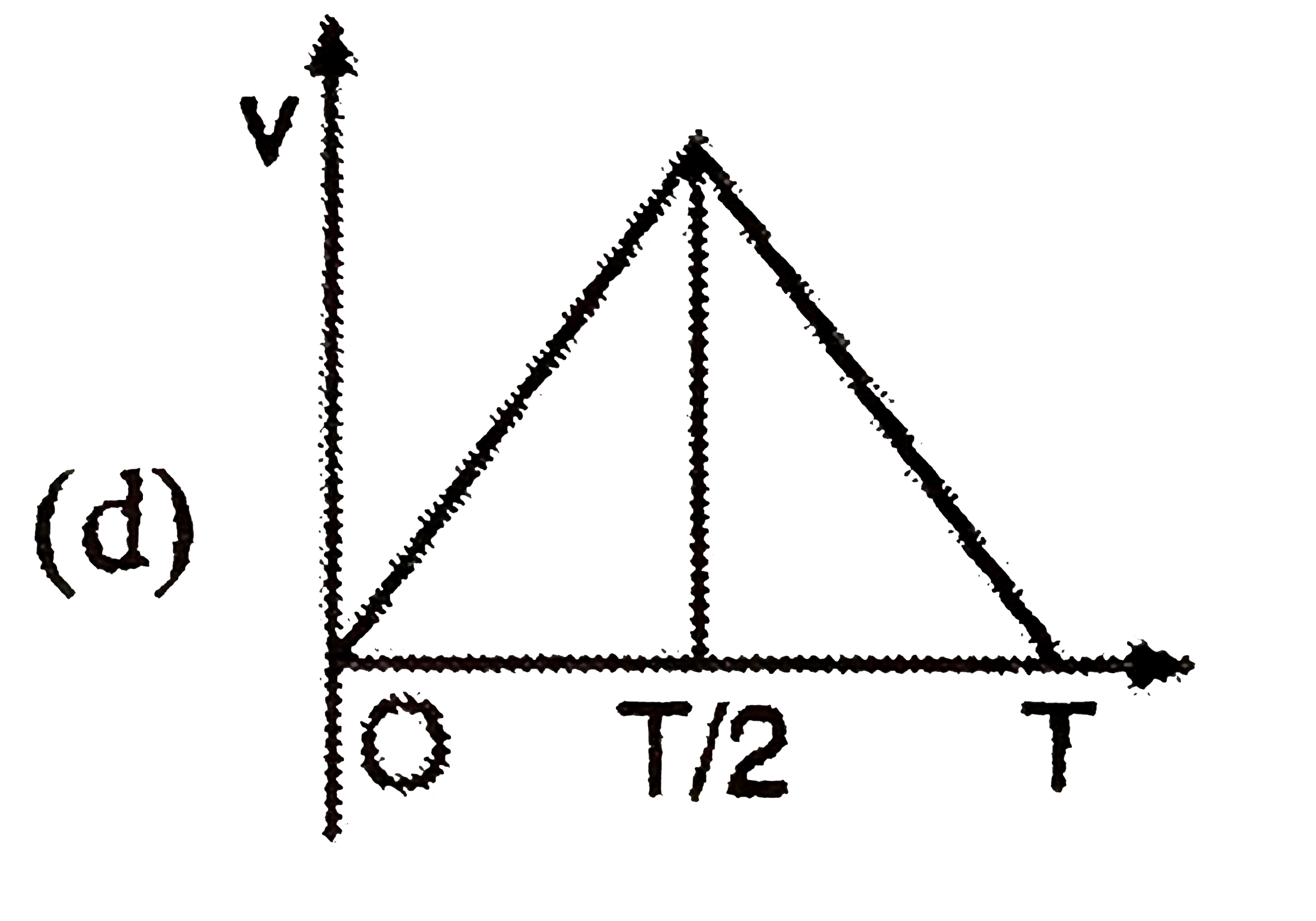
A particle thrown up vertically reaches its highest point in time t1 and returns to the ground in a further time t2. The air resistance exerts a constant force on the particle opposite to its direction of motion.
At1>t2Bt1=t2Ct1<t2Dmay be (A) or (C) depending on the ratio of the force of air resistance to the wight of the particle.
A particle is dropped from the top of a tower of height 80m. Find the time of jurney and the speed with which it strikes the ground.
View SolutionThe velocity of a particle moving in the positive direction of the X-axis varies as V=K√S where K is a positive constant. Draw V−t graph.
View SolutionA particle is projected vertically upwards with a velocity v. It returns to the ground in time T. Which of the following graphs correctly represents the motion.
View SolutionThe velocity of a particle moving in the positive direction of the X-axis varies as V=K√S where K is a positive constant. Draw V-t graph.
View SolutionThe acceleration of a particle travelling along a straight line is a=kv, where k is constant (k>0). If at t=0,V=V0 then speed of particle at t=V202.
View Solution
