Question

Two men of masses $m$ and $m/2$ starts climbing up on two masseless strings fixed at the ceiling with acceleration $g$ and $g/2$ respectively. The ratio of tensions in the two strings will be $:$

Options

A. `2:1`
B. `4:1`
C. `4:3`
D. `8:3`

Answer

D

Explanation

FBD of man of mass `(m)`

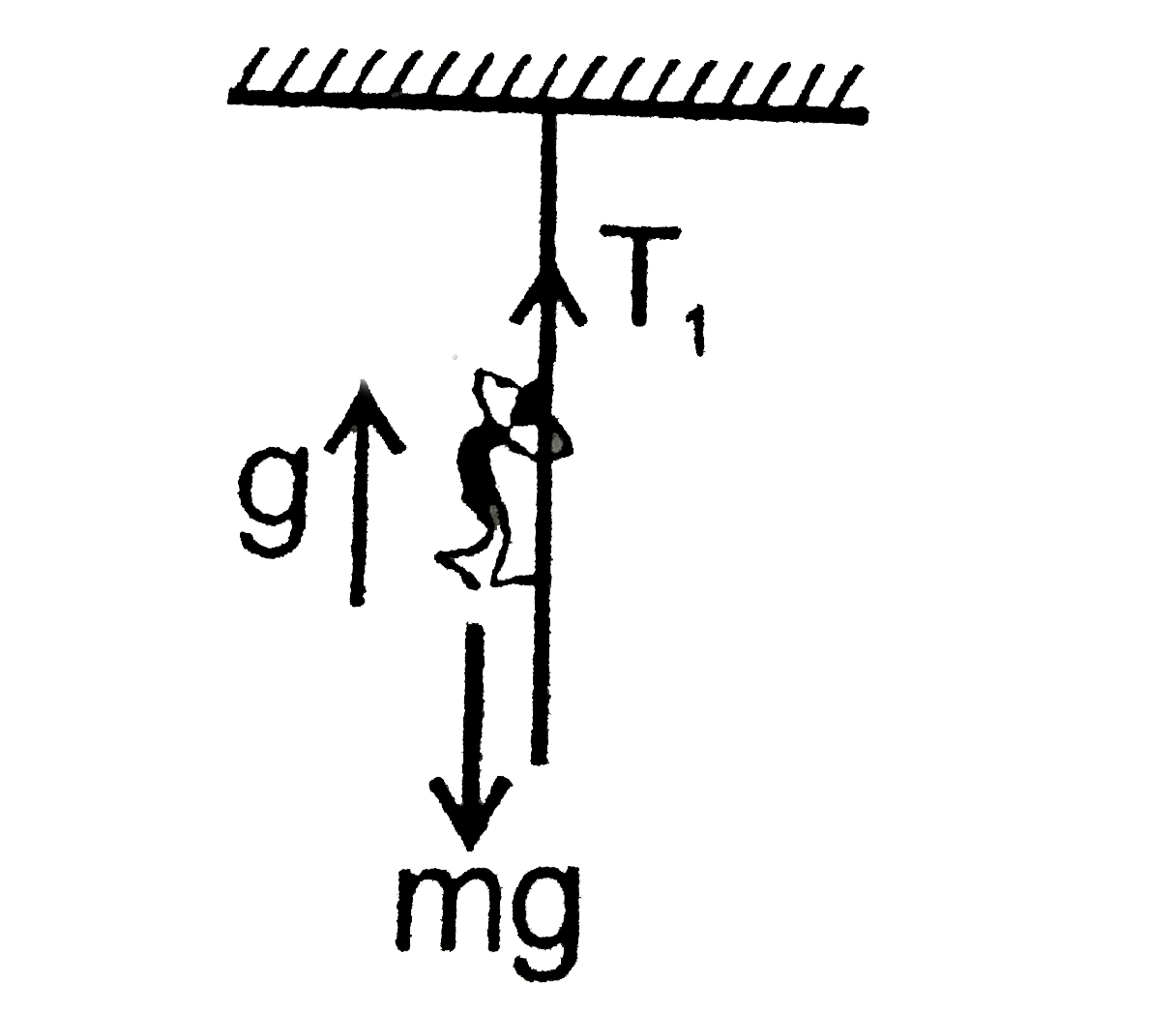
`T_(1)=mg+mg`
`T_(1)=2mg`
`rArr T_(1):T_(2): : 8:3`
FBD of man of mass `(m//2)`

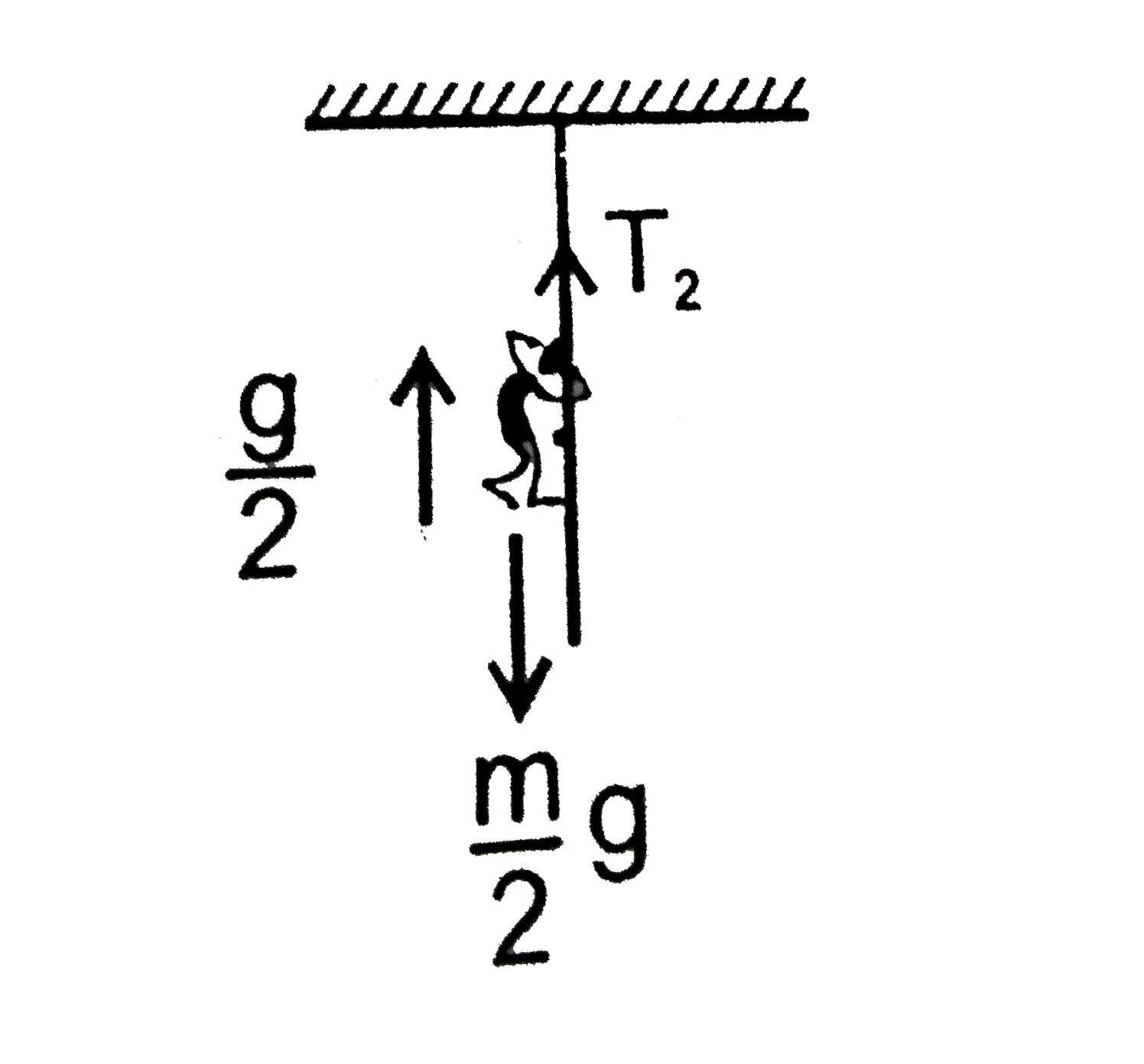
`T_(2)=(m)/(2)g+(m)/(2).(g)/(2)`
`T_(2)=(mg)/(2)[(3)/(2)]=(3mg)/(4)`