Question

A ring of radius $R$ is made of a thin wire of material of density $\rho$ having cross section area $a.$ The ring rotates with angular velocity $\omega$ about an axis passing through its centre and perpendicular to the plane. If we consider a small element of the ring,it rotates in a circle. The required centripetal force is provided by the component of tensions on the element towards the centre. A small element of length $dl$ of angular width $d\theta$ is shown in the figure.

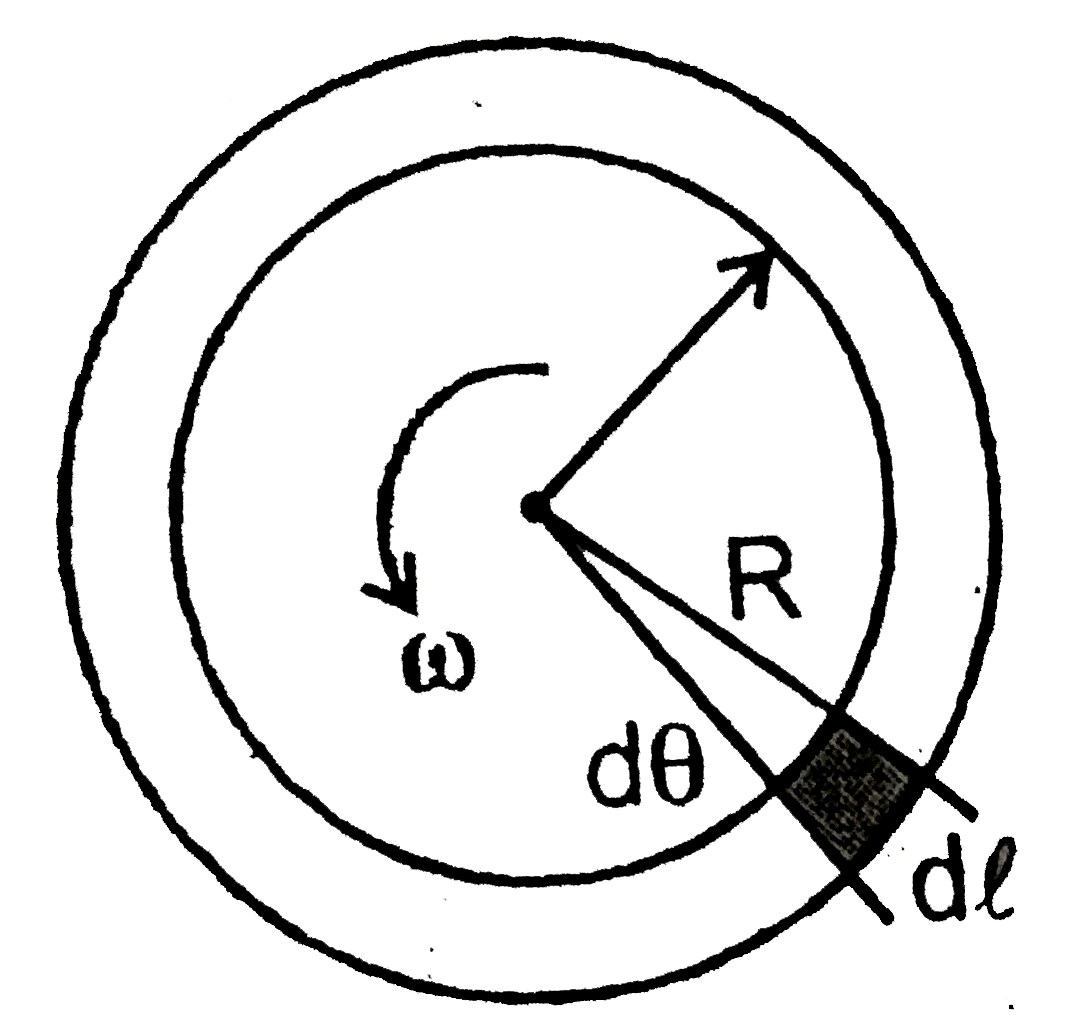
If $T$ is the tension in the ring, then

Options

A. `(a.rhodlomega^(2)R)`
B. `R^(2) d theta.omega^(2)`
C. `(1)/(2)a rho d l omega^(2) R`
D. zero

Answer

A

Explanation

As the small element `(dm =a.rho.dl)` in rotating int the circle, centripetal force
`F_(C)=d m omega^(2)R=a rho d l . Omega^(2)R`