There are four arrangements of a solid cylinder and a plank as shown in the figures. Some surfaces are smooth and some are rough as indicated . There is no slipping at each rough surface. The plank and / or centre of cylinder are given a horizontal constant velocity as shown in each of the situations. Usinn this information fill in the blanks.
![There are four arrangements of a solid cylinder and a plank as shown in the figures. Some surfaces are smooth and some are rough as indicated . There is no slipping at each rough surface. The plank and // or centre of cylinder are given a horizontal constant velocity as shown in each of the situations. Usinn this information fill in the blanks. The speed of center of mass of the cylinder is .......................... (ii) , The angular velocity of the cylinder is ........................ (iii),(RESPHYDPP04XIE01537Q03.png]() The speed of center of mass of the cylindr is ............... . (iv), The angular velocity of the cylinder is ............ . (a) V (b) V//R (c) 2V//R (d) 4V//R (e) cannot be determined from the given information (f) Zero." loading="eager" src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/RES_PHY_DPP_67_XI_E01_537_Q01.png" width="80%" class="mx-auto" style="background:white;"> The speed of center of mass of the cylinder is ..........................
The speed of center of mass of the cylindr is ............... . (iv), The angular velocity of the cylinder is ............ . (a) V (b) V//R (c) 2V//R (d) 4V//R (e) cannot be determined from the given information (f) Zero." loading="eager" src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/RES_PHY_DPP_67_XI_E01_537_Q01.png" width="80%" class="mx-auto" style="background:white;"> The speed of center of mass of the cylinder is ..........................
(ii) , The angular velocity of the cylinder is ........................
The angular velocity of the cylinder is ........................
(iii),(RESPHYDPP04_XIE01537_Q03.png width=80%>Thespeedofcenterofmassofthecyl∈dris................<br>(iv),<imga≤ocr_imageload∈g=lazysrc=https://d10lpgp6xz60nq.cloudfront.net/physics_images/RES_PHY_DPP_04_XI_E01_537_Q04.pngwh=80%mx-autosty≤=background:white;>Theanga––rvelocityofthecyl∈deris.............<br>(a) V<br>(b) V//R<br>(c) 2V//R<br>(d) 4V//R<br>(e)ca∩otbedeterminedomthegiven∈formation<br>(f) Zero.
(i)d(ii)b(ii)f(iv)c
(i)e(ii)v(iii)f(iv)b
(i)e(ii)d(iii)f(iv)c
(i)e(ii)v(iii)f(iv)a
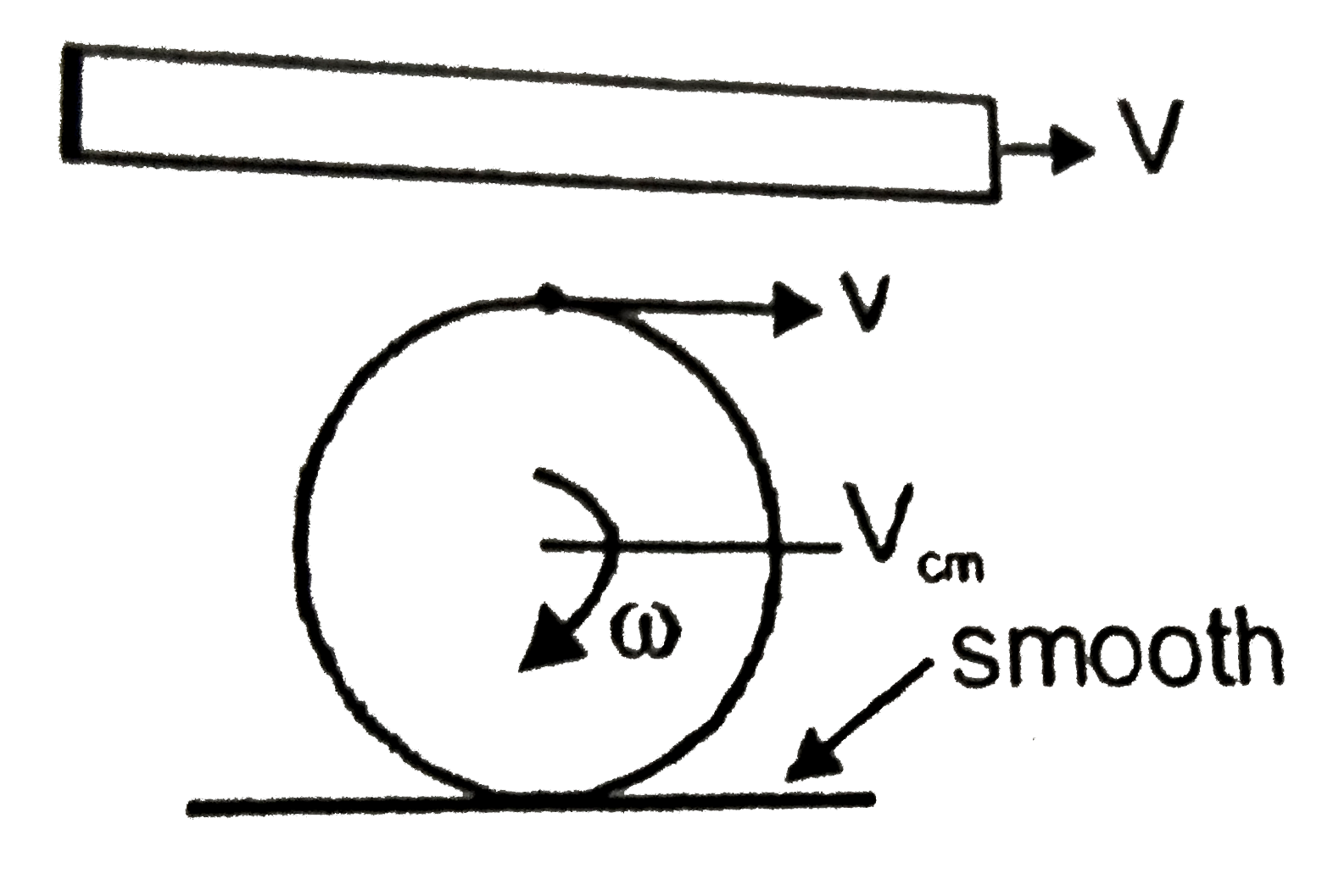
Vcm=ωR=V,Vcm=V−ωR
ω depends on value of friction between plank & cylinder, hence Vcm is undetermined.
(ii)ω=2v2R=VR
(ii)ω=2V2R=VR, hence Vcm=0
(iv)ωA/C=3V+VR=4VR⇒ω=4VR
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMSBook:RESONANCE ENGLISHChapter:DAILY PRACTICE PROBLEMSExercise:dpp 68
Explore 7 Videos
- DAILY PRACTICE PROBLEMSBook:RESONANCE ENGLISHChapter:DAILY PRACTICE PROBLEMSExercise:dpp 69
Explore 5 Videos
- DAILY PRACTICE PROBLEMSBook:RESONANCE ENGLISHChapter:DAILY PRACTICE PROBLEMSExercise:dpp 66
Explore 4 Videos
- CURRENT ELECTRICITYBook:RESONANCE ENGLISHChapter:CURRENT ELECTRICITYExercise:Exercise
Explore 53 Videos
- ELASTICITY AND VISCOCITYBook:RESONANCE ENGLISHChapter:ELASTICITY AND VISCOCITYExercise:Advanced Level Problems
Explore 9 Videos
Similar Questions
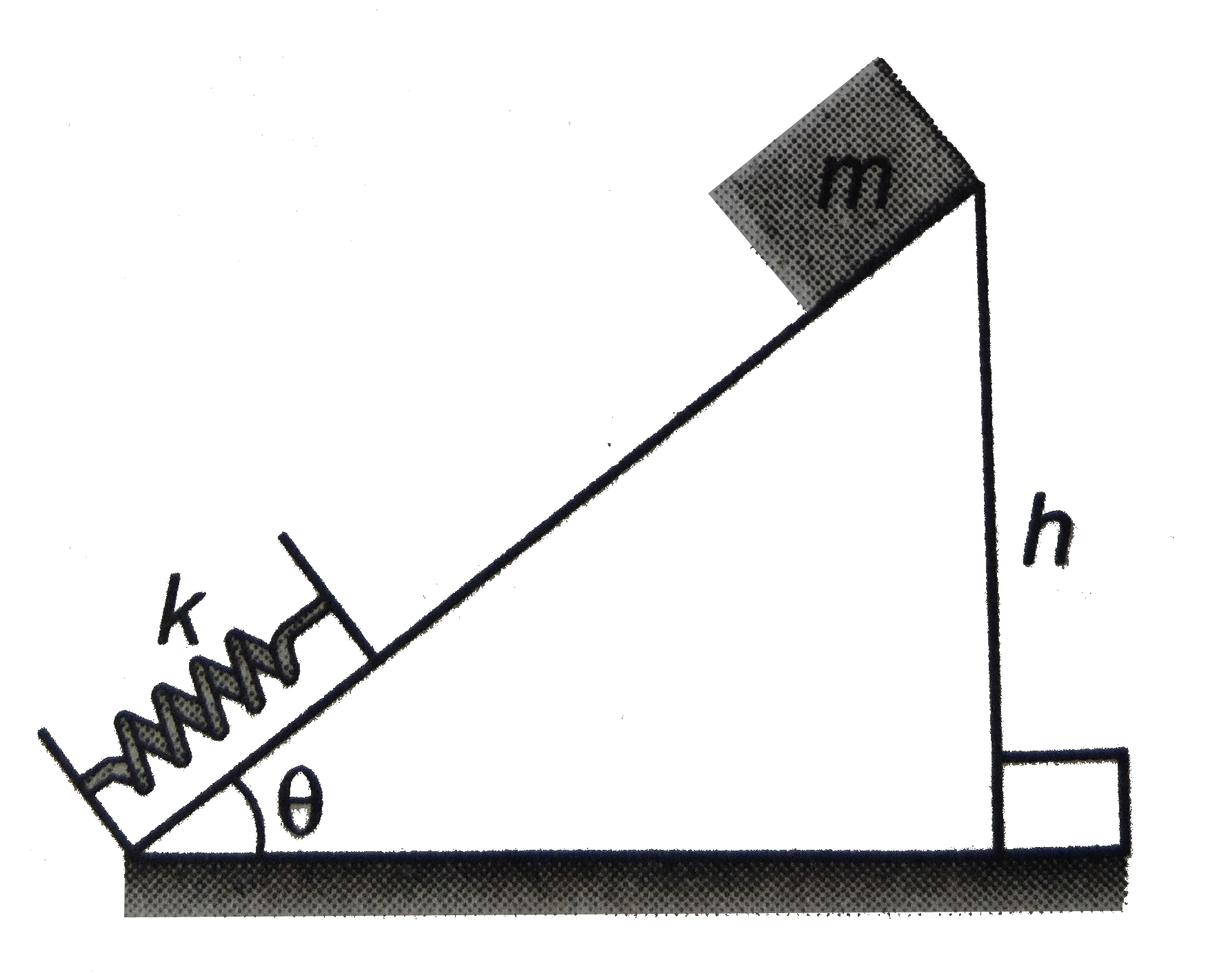
A body of mass m is released from a height h on a smooth inclined plane that is shown in the figure. The following can be true about the velocity of the block knowing that the wedge is fixed.
 .
.
View SolutionA uniform disc of mass 300 kg is rotating freely about a vertical axis through its centre at a constant angular velocity ω0. A boy of mass 30 kg starts from the centre and moves along a radius to the edge of the disc. Angular velocity of the disc ill now become
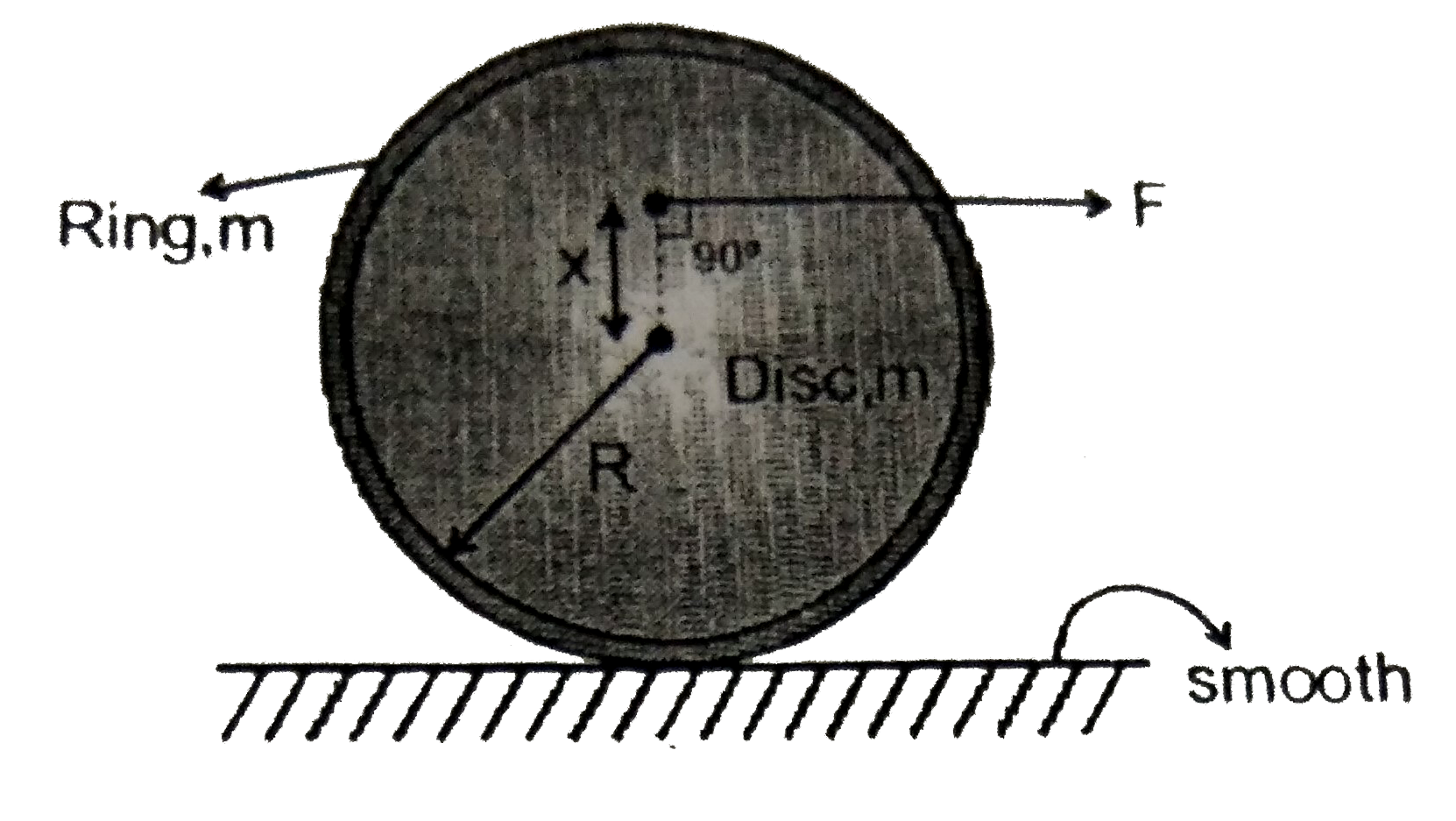
View SolutionA ring and a disc of same mass m and same radius R are joinged concentrically. This system is placed on a smooth plane with the common axis parallel to the plane as shown in figure. A horizontal force F is applied on the system at a point which is at a distance x from the centre. The value of x so that it starts pure rolling is
View SolutionSpecific resistance of a wire depends on the
View SolutionA wire loaded by a weight of density 7.8g/cc is found to be of length 100cm. On immersing the weight in water. The length decrease by 0.20 cm find the original length of the wire.
View Solution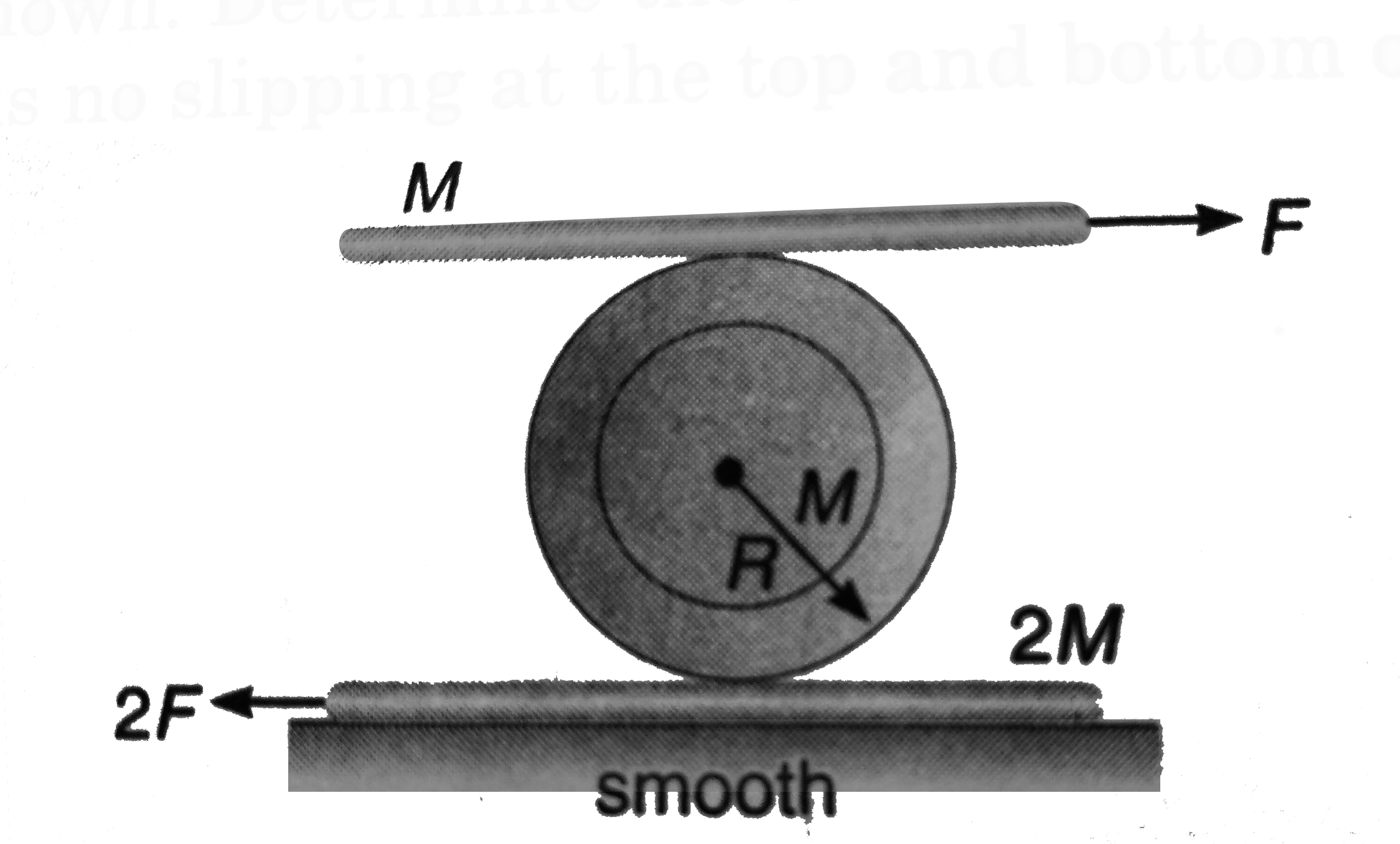
A cylinder is sandwiched between two planks. Two constant horizontal forces F and 2F are applied on the planks as shown. Determine the acceleration of the centre of mass of cylinder and the top plank. If there is no slipping at the top and bottom of cylinder.
View SolutionA cylinder executes pure rolling without slipping with a constant velocity on a plank, whose upper surface is rough enough, but lower surface is smooth. The plank is kept at rest on a smooth horizontal surface by the application of an external force F . Choose the correct alternative:
View SolutionIn the following problems, indicate the correct direction of friction force acting on the cylinder, which is pulled on a rough surface by a constant force F . A cylinder is placed on a rough plank which in turn is placed on a smooth surface. The plank is pulled with a constant force F . The friction force can be given by which of the following diagrams. .
View SolutionConsider a cylinder of mass M=1 kg and radius R=1 m lying on a rough horizontal plane. It has a plank lying on its stop as shown in the figure. A force F=55N is applied on the plank moves and causes the cylinder to roll. The plank always remains horizontal. there is no slipping at any point of contact. The acceleration of cylinder is
View SolutionThere are four arrangements of a solid cylinder and a plank as shown in the figures. Some surfaces are smooth and some are rough as indicated . There is no slipping at each rough surface. The plank and / or centre of cylinder are given a horizontal constant velocity as shown in each of the situations. Usinn this information fill in the blanks.
 The speed of center of mass of the cylinder is ..........................
The speed of center of mass of the cylinder is ..........................
(ii) , The angular velocity of the cylinder is ........................
The angular velocity of the cylinder is ........................
(iii),(RESPHYDPP04_XIE01537_Q03.png width=80%>Thespeedofcenterofmassofthecyl∈dris................<br>(iv),<imga≤ocr_imageload∈g=lazysrc=https://d10lpgp6xz60nq.cloudfront.net/physics_images/RES_PHY_DPP_04_XI_E01_537_Q04.pngwh=80%mx-autosty≤=background:white;>Theanga––rvelocityofthecyl∈deris.............<br>(a) V<br>(b) V//R<br>(c) 2V//R<br>(d) 4V//R<br>(e)ca∩otbedeterminedomthegiven∈formation<br>(f) Zero.
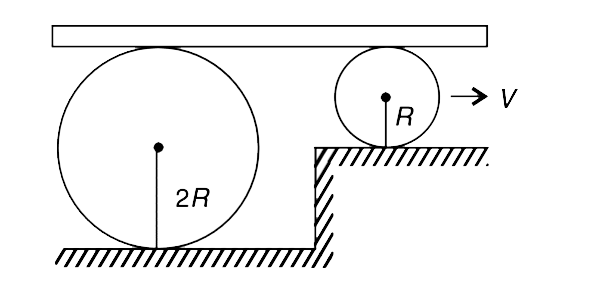
View SolutionIn the figure the plank resting on two cylinders is horizontal. The plank is pulled to the right such that the centre of smaller cylinder moves with a constant velocity v. Friction is large enough to prevent slipping at all surfaces. Find-
(a) The velocity of the centre of larger cylinder.
(b) The ratio of accelerations of the points of contact of the two cylinders with the plank.
View SolutionIn the figure shown a plank of mass m is lying at rest on a smooth horizontal surface. A cylinder of same mass m and radius r is rotated to an angular speed w0 and then gently placed on the plank. It is found that by the time the slipping between the plank and the cylinder cease, 50% of total kinetic energy of the cylinder and plank system is lost. Assume that plank is long enough and um is the coefficient of friction between the cylinder and the plank.
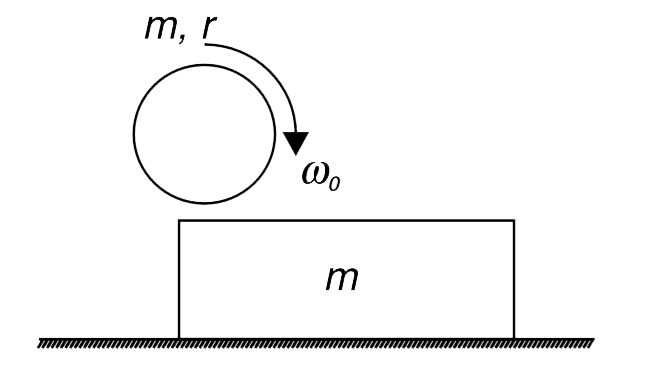
(a) Find the final velocity of the plank.
(b) Calculate the magnitude of the change in angular momentum of the cylinder about its centre of mass.
(c) Distance moved by the plank by the time slipping ceases between cylinder and plank.
View SolutionA plank of mass M is placed on a smooth surface over which a cylinder of mass m and radius R is placed as shown in figure-5.63. Now the plank is pulled towards right with an external force F. If cylinder does not slip over the surface of plank find the linear acceleration of plank and cylinder and the angular acceleration of the cylinder.
View SolutionComprehension A solid cylinder of mass mis rotating brought in contact with a plank of ma cylinder and plank because of which there is no slipping between cylinder and plank m is rotating with angular velocity foxy about its fixed axis of symmetry. It is at with a plank of mass m placed on a smooth om a smooth surface. There exist friction between se of which plank starts moving. After some time plank moves.such that in friction does the work m Rough surface 7772 ZEITEN Smooth surface The final velocity of the plank when there is no slipping between cylinder and plank is: (0) Ros Roo 3RW (A) 2Roo Roo (B) 4
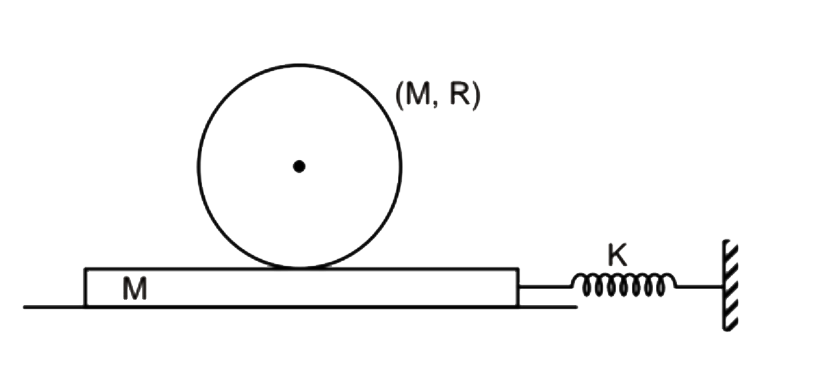
View SolutionA cylinder of mass M and radius R lies on a plank of mass M as shown. The surface between plank and ground is smooth, and between cylinder and plank is rough. Assuming no slipping between cylinder and plank, the time period of oscillation (When displaced from equilibrium) of the system is
View SolutionA force F is applied horizontally on a cylinder in the line of centre as shown in the figure. The cylinder is on a rough surface of coefficient of fricition mu . The direction of the friction force acting on the cylinder will be
View Solution
