Which of the following relations is correct for a spherical mirror if a point object is kept on the principal axis ['P' is pole 'C' is centre object is at point 'O'. Image is at point 'I'].
OPOC=IPIC
OPIC=IPOC
PCPO=PIPC
IOCP=IPCO
The correct Answer is:A
To solve the problem regarding the correct relation for a spherical mirror when a point object is placed on the principal axis, we can follow these steps:
1. Understand the Setup:
- We have a spherical mirror with a pole (P), center of curvature (C), an object located at point O, and an image formed at point I.
2. Identify the Triangles:
- We can form two triangles based on the positions of the object and the image. The first triangle is formed by points O, O' (the image of the object), and P (the pole of the mirror). The second triangle is formed by points I, I' (the image of the image), and P.
3. Establish Similarity of Triangles:
- Since both triangles share the angle at P and the angles at O and I are equal (due to the law of reflection), we can conclude that triangle OOP is similar to triangle IIP.
4. Write the Ratio from Similar Triangles:
- From the similarity of triangles, we can write the following relation:
OO′II′=OPIP
5. Consider the Other Set of Triangles:
- Similarly, we can consider triangles OOC (where C is the center of curvature) and IIC. These triangles are also similar because they share the angle at C and have right angles at O and I.
- Thus, we can write:
OO′II′=OCIC
6. Combine the Relations:
- Since both ratios are equal to OO′II′, we can equate them:
OPIP=OCIC
7. Final Relation:
- Rearranging gives us:
OPOC=ICIP
- This shows the relationship between the distances of the object and image from the pole and center of curvature.
Conclusion:
The correct relation for a spherical mirror when a point object is kept on the principal axis is:
OPOC=ICIP
- We have a spherical mirror with a pole (P), center of curvature (C), an object located at point O, and an image formed at point I.
- We can form two triangles based on the positions of the object and the image. The first triangle is formed by points O, O' (the image of the object), and P (the pole of the mirror). The second triangle is formed by points I, I' (the image of the image), and P.
- Since both triangles share the angle at P and the angles at O and I are equal (due to the law of reflection), we can conclude that triangle OOP is similar to triangle IIP.
- From the similarity of triangles, we can write the following relation:
OO′II′=OPIP
- Similarly, we can consider triangles OOC (where C is the center of curvature) and IIC. These triangles are also similar because they share the angle at C and have right angles at O and I.
- Thus, we can write:
OO′II′=OCIC
- Since both ratios are equal to OO′II′, we can equate them:
OPIP=OCIC
- Rearranging gives us:
OPOC=ICIP
- This shows the relationship between the distances of the object and image from the pole and center of curvature.
The correct relation for a spherical mirror when a point object is kept on the principal axis is:
OPOC=ICIP
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.10
9 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.11
20 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.8
9 Videos
- CURRENT ELECTRICITYBOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)
19 Videos
- ELECTRO MAGNETIC WAVESBOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 3
27 Videos
Similar Questions
The image of an exend object placed perpendicular to the principal axis of a mirror, will be erect if
View SolutionAn object is placed on principal axis of concave mirror at distance of 1.5 f Image will be at
View SolutionAn object is 40 cm form a spherical mirror, along the central axis. The image produced is inverted. What is the power of the mirror?
View SolutionAn object is between the principal focus and the centre of curvature of a concave mirror. The image formed is real and :
View SolutionA convex lens of focal length f and a plane mirror are y distance apart. An object O is kept on the principal axis of the lens at a distance x from the lens. The values of x and y for the final image of O to fall exactly (position and size) on the object O are :

View SolutionIn the figureshownm the image of a real object is formed at point I. AB is the principal axis of the mirror. The mirror must be
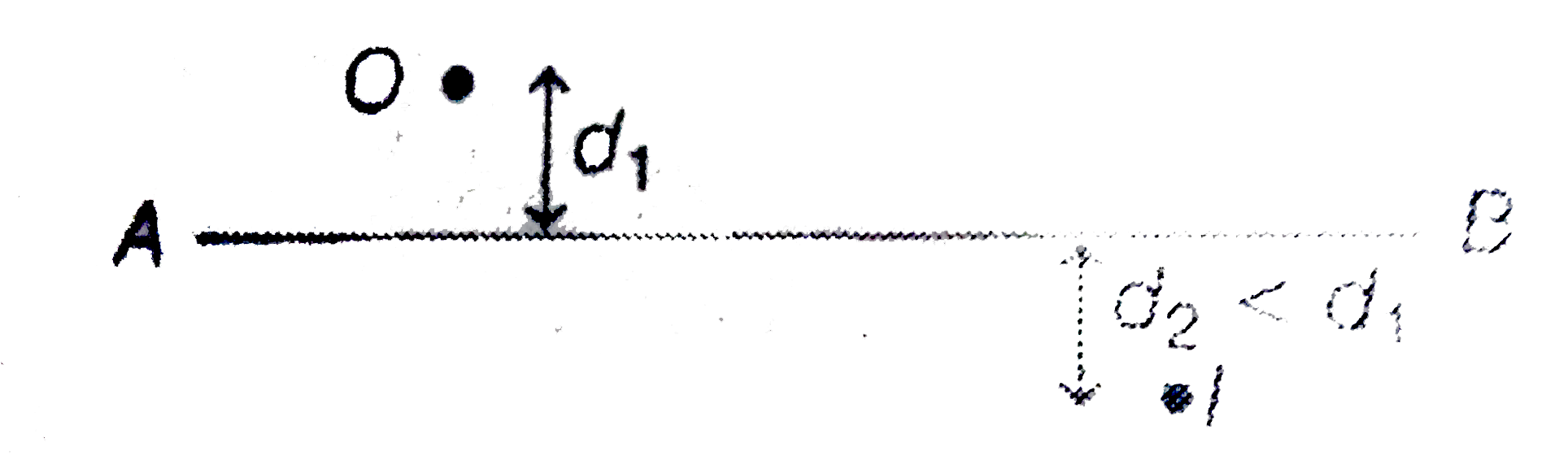
View SolutionAB is the principal axis of a spherical mirror. I is the point image corresponding to a point object O. Choose the correct options.

View SolutionA point object at a distance 5R/3 from the pole of a concave mirror. R is the radius of curvature of mirror. Point object oscillates with amplitude of 1mm perpendicular to the principal axis.
Position of image when object is at O is :
(3/7)R
(5/7)R
(2/7)R
(4/7)R
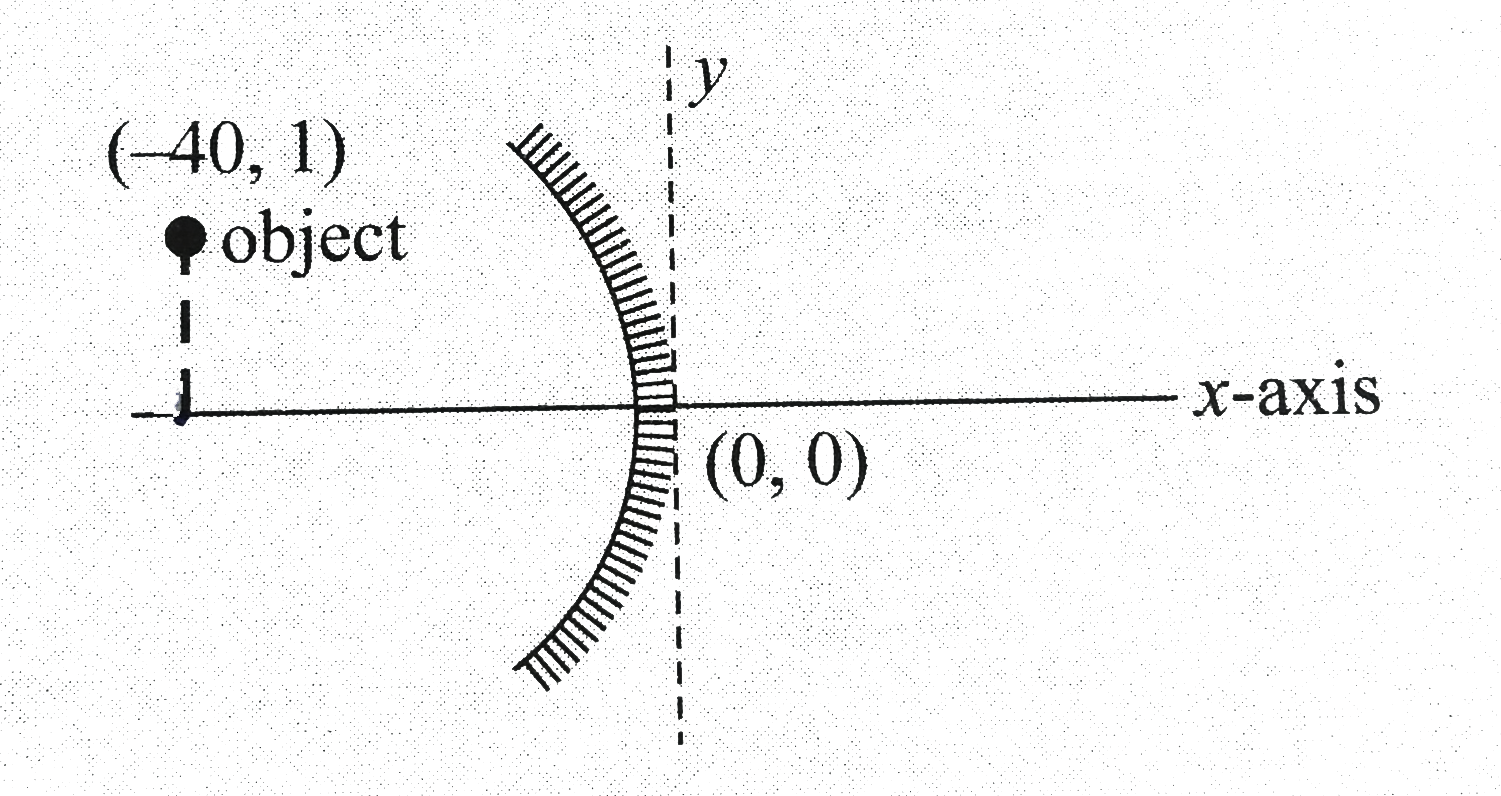
View SolutionFigure shows a shperical concave mirror of focal length f=5cm with its pole at (0,0) and principal axis along x-axis. There is a point object at (-40cm, 1cm), find the postion of image.
View SolutionA convex lens of focal length f and a plane mirror are y distance apart. An object O is kept on the principal axis of the lens at a distance x from the lens. The values of x and y for the final image of O to fall exactly (position and size) on the object O are :

View Solution