Magnetic field along x-axis varies according to the relation →B=B0xˆi. Given a coil of area A with its axis along x-axis is connected over the top of a plastic trolly which moves along x-axis with velocity v. If the resistance of coil is R, then (at t= 0, coil is at x = 0 and v=v0)
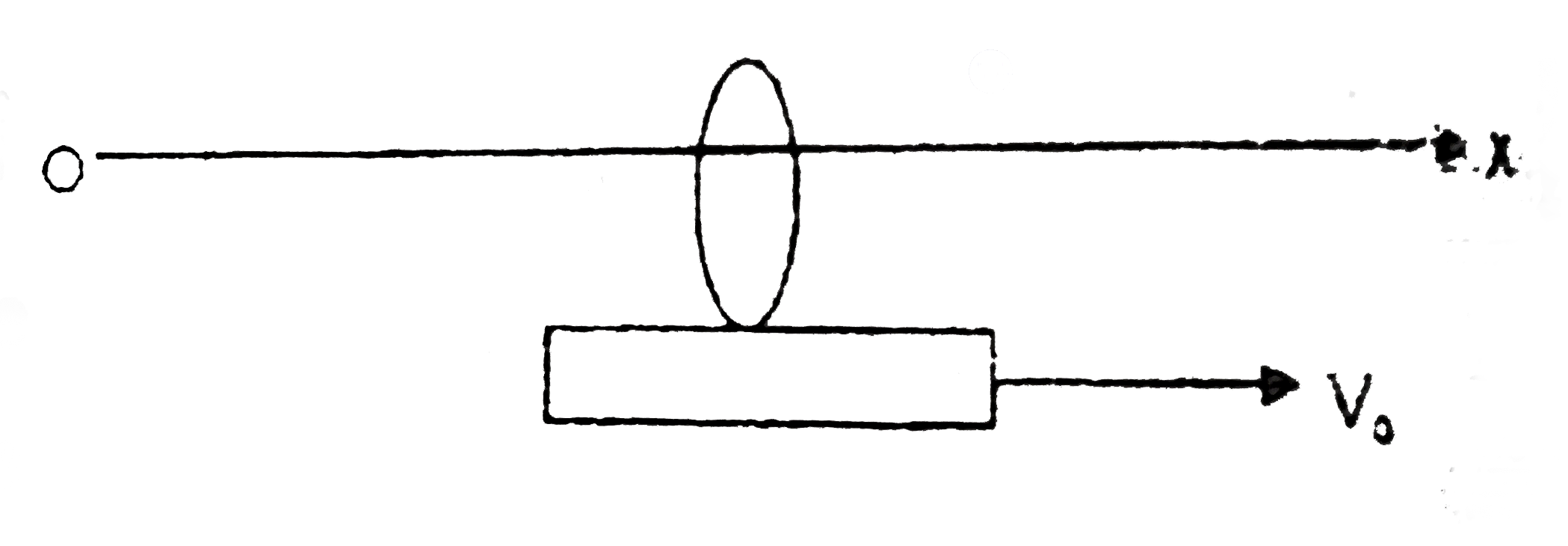
The flux linked with the coil at any position x is B0xA
An observer at origin 'O' finds the induced current as B0Av0R anticlockwise it trolly moves with constant velocity v=v0
If the trolly has acceleration α then the induced current as a function of time t is given as B0A(v0+αt)R anticlockwise
If the trolly has acceleration α then the induced current as a function of position x is given as B0A(√v20+2αx)R anticlockwise
The correct Answer is:A, B, C, D
ϕ=→B.→A=B0xA⇒dϕdt=B0Adxdt=B0Av
apply e=dϕdt
i=|e|R=B0v.AR anticlock wise
∴v=v0+αtB0A(v0+αt)R anticlockwise
v2=u2+2αx,i
=B0A(√V20+2αx)R anticlock wise.
Topper's Solved these Questions
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.559videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.5620videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.5320videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)19videos
CURRENT ELECTRICITY
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 327videos
ELECTRO MAGNETIC WAVES
Similar Questions
The electric potetnial in a region along x-axis varies with x according to the releation V(x)=4×5x2. Then the incorrect statement is
View SolutionA particle is moving along x-axis. Its velocity v with x co-ordinate is varying as v=√x. Then
View SolutionA particle moves along x-axis according to relation x=1+2sinωt. The amplitude of S.H.M. is
View SolutionFor motion of an object along the x-axis the velocity v dipends on the displacement x an v=3x2, then what is the acceleration at x=2m.
View SolutionThe position of a particle moving along x- axis is given by x=x0cos2(ωt) . Its when it is at mean position is
View SolutionIf v is the velocity of a body moving along x-axis, then acceleration of body is
View SolutionIn a region there exists a magnetic field B0 along positive x-axis. A metallic wire of length 2a, one side along x-axis and one side parallel of y-axis is rotates about y-axis with a angular velocity. Then at the instant shown
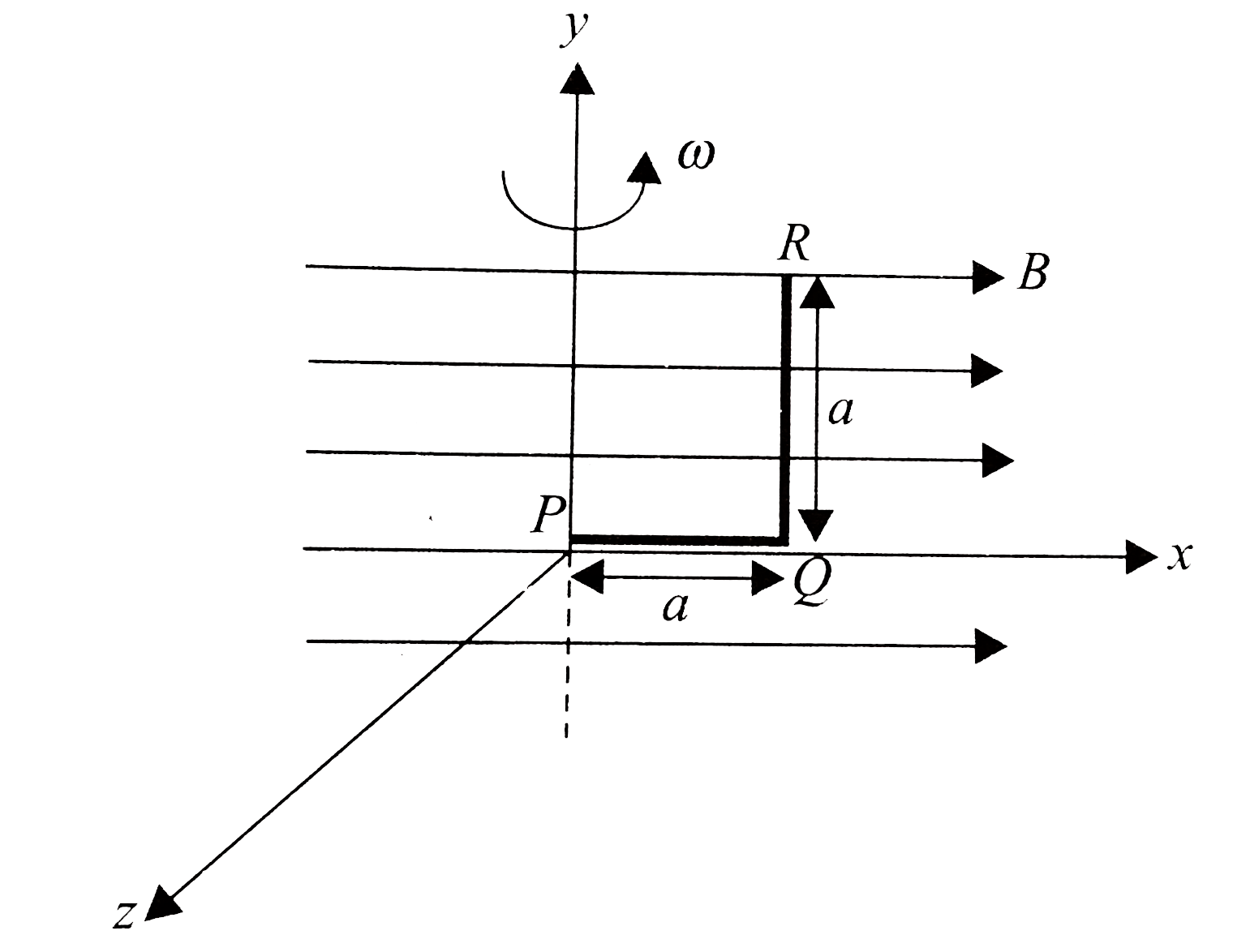 View Solution
View SolutionThe velocity of a particle moving in the positive direction of x-axis veries as v=10√x. Assuming that at t=0, particle was at x=0
View SolutionA time varying force, F=2t is acting on a particle of mass 2kg moving along x-axis. velocity of the particle is 4m/s along negative x-axis at time t=0. Find the velocity of the particle at the end of 4s.
View SolutionThe magnetic field in a region is given by →B=B0(1+xa)ˆk. A square loop of edge length 'd' is placed with its edge along X-axis and Y-axis. The loop is moved with a constant velocity →V=V0ˆi. The emf induced in the loop is
View Solution

