A wire AB (of length 1m, area of cross section πm2) is used in potentiometer experiment to calculate emf and internal resistance (r) of battery.
The emf and internal resistance of driving battery are 15 V and 3Ω respectively.The resistivity of wire AB varies as ρ=ρ0x (where x is distance form A in meters and ρ0=24πΩ)
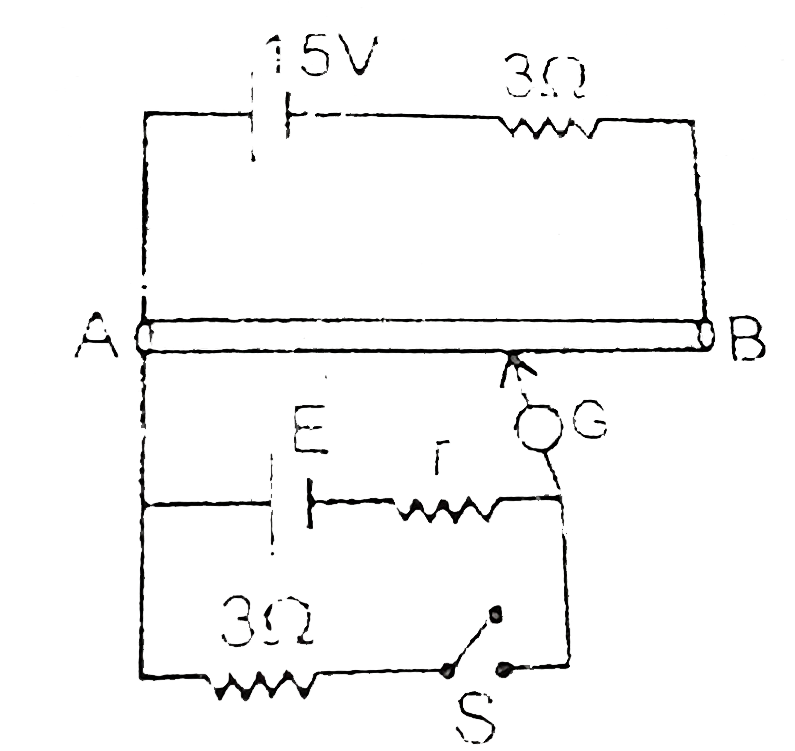
The distance of null point from A obtained at √23m when switch 'S' is open and at 1√2m m when switch is closed.
The emf (E) of battery is -
4 V
6V
8V
10 V
The correct Answer is:C
Resitance of wire AB is -
RAB=(ρ02)lA=24π2π=12Ω ⎧⎪⎨⎪⎩R=∫0ρdxA⎫⎪⎬⎪⎭
Current in wire AB is I=1512+3=1A
when switch is open, null point at C(AC=x)
RAC=(ρ0x2)(xA)=ρ0x22A=⎛⎝24π232π⎞⎠8Ω
EMF E=1×8=8V
when switch closed null point at D(AD=x)
RAD=(ρ02)(xA)=ρ0x22A=⎛⎝24−122π⎞⎠=θΩ
ΔVbattery=6×1
8−8r+3r=6
r=1Ω
Topper's Solved these Questions
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.6120videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.629videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.599videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)19videos
CURRENT ELECTRICITY
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 327videos
ELECTRO MAGNETIC WAVES
Similar Questions
A wire AB (of length 1m, area of cross section πm2) is used in potentiometer experiment to calculate emf and internal resistance (r) of battery.
The emf and internal resistance of driving battery are 15 V and 3Ω respectively.The resistivity of wire AB varies as ρ=ρ0x (where x is distance form A in meters and ρ0=24πΩ)
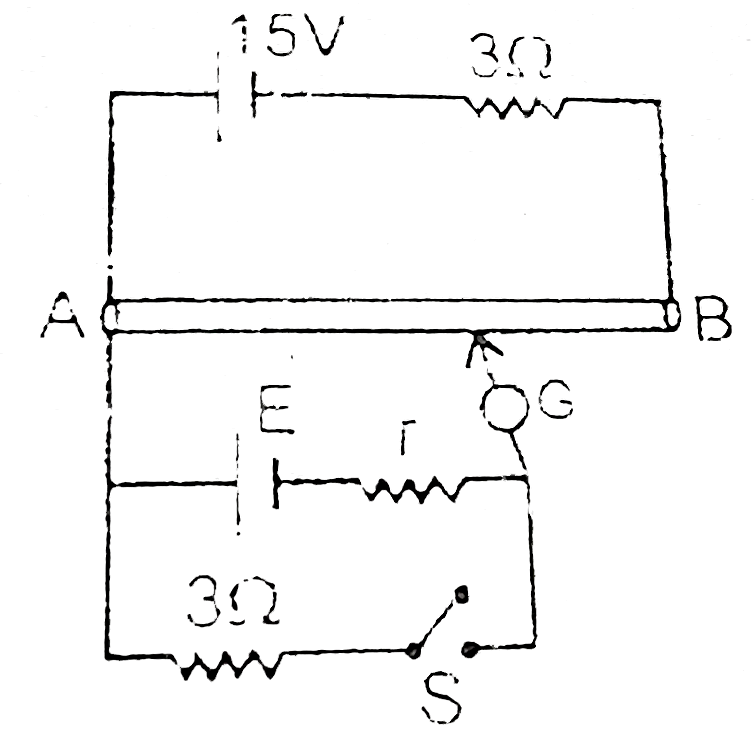
The distance of null point from A obtained at √23m when switch 'S' is open and at 1√2m m when switch is closed.
Internal resistance of battery E is -View SolutionA wire AB (of length 1m, area of cross section πm2) is used in potentiometer experiment to calculate emf and internal resistance (r) of battery.
The emf and internal resistance of driving battery are 15 V and 3Ω respectively.The resistivity of wire AB varies as ρ=ρ0x (where x is distance form A in meters and ρ0=24πΩ)
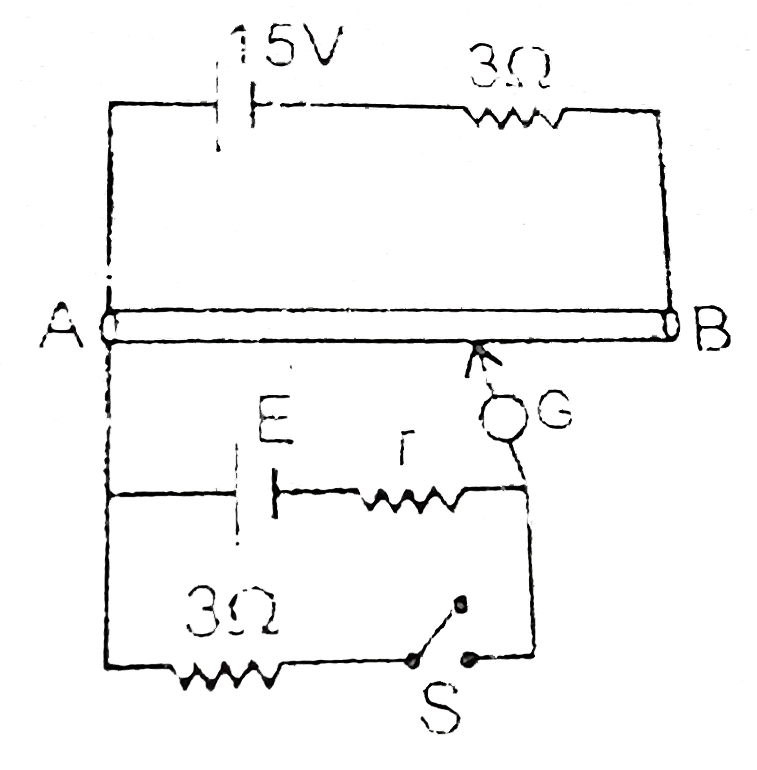
The distance of null point from A obtained at √23m when switch 'S' is open and at 1√2m m when switch is closed.
The resistance of whole wire AB is-View SolutionA wire AB (of length 1m, area of cross section πm2) is used in potentiometer experiment to calculate emf and internal resistance (r) of battery.
The emf and internal resistance of driving battery are 15 V and 3Ω respectively.The resistivity of wire AB varies as ρ=ρ0x (where x is distance form A in meters and ρ0=24πΩ)

The distance of null point from A obtained at √23m when switch 'S' is open and at 1√2m m when switch is closed.
The current through 15 V battery (with IG=0)View SolutionTwo batteries, one of emf 18V and internal resistance 2Ω and the other of emf 12 and internal resistance 1Ω , are connected as shown. The voltmeter V will record a reading of
 View Solution
View SolutionIf three uniform spheres, each having mass M and radius R, are kept in such a way that each touches the other two, the magnitude of the gravitational force on any sphere due to the other two is:
View SolutionA battery of EMF E produces currents 4 A and 3 A when connected to external resistance 1Ω and 2Ω respectively. The internal resistance of the battery is
View SolutionA potentiometer wire is 10 m long and has a resistance of 18Ω. It is connected to a battery of emf 5 V and internal resistance 2Ω. Calculate the potential gradient along the wire.
View SolutionA simple potentiometer circuit is shown in the figure. The internal resistance of 5 V battery is 0.6Ω wire of length 4m and resistance 0.5Ω per meter. The length AB for which galvanometer shows zero deflection is
_PHY_E05_097_Q01.png) View Solution
View SolutionA 5 V battery with internal resistance 1Ω and a 2 V battery with internal resistance 0.5Ω are connected to a 3Ω resistor as shown in the figure.The current in the 3Ω resistor is
View SolutionIn the ciruit the cells E1andE2 have emfs of 4V and 8V and internal resistance 0.5Ωand1.0Ω, respectively. Calculate the current through 6Ω resistance.
 View Solution
View Solution


