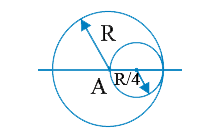
The centre of mass of the shaded portion of the disc is :
(The mass is uniformly distributed in the shaded portion)
R20 to the left of A
R12 to the left of A
R20 to the right of A
R12 to the right of A
The correct Answer is:A
A1=πR2,A2=πR216
x1=0,x2=3R4
xcen=0−πR216×3R4πR2−πR216=−R20
Topper's Solved these Questions
Similar Questions
The centre of mass of the shaped portion of the disc is (The mass is uniformly distributed in the shaded portion)
 View Solution
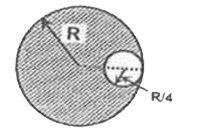
View SolutionFind the centre of mass of the shaded portion of a disc.
 View Solution
View SolutionFind the centre of mass of the section. Consider the mass of the lamina to be uniformly distributed.
 View Solution
View SolutionWrite the fraction of the shaded portion in the following figures.
 View Solution
View SolutionWrite the fraction of the shaded portion in the following figures.
 View Solution
View SolutionWrite the fraction of the shaded portion in the following figures.
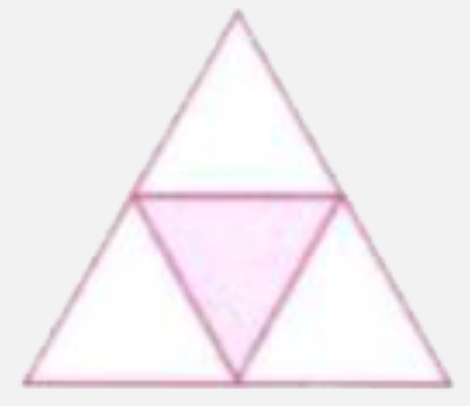 View Solution
View SolutionWrite the fraction of the shaded portion in the following figures.
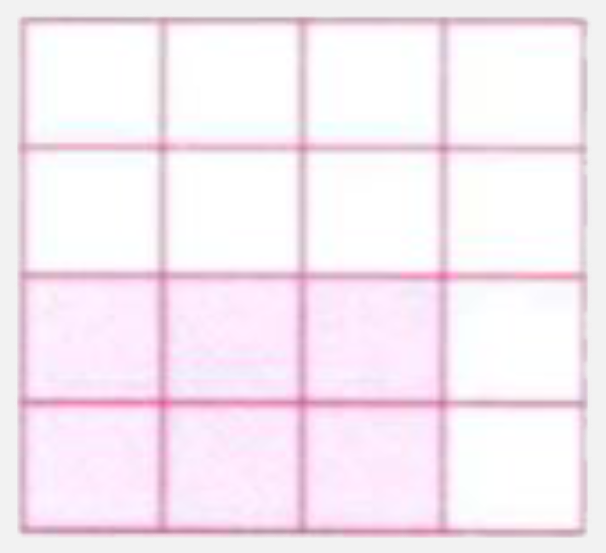 View Solution
View SolutionWrite the fraction of the shaded portion in the following figures.
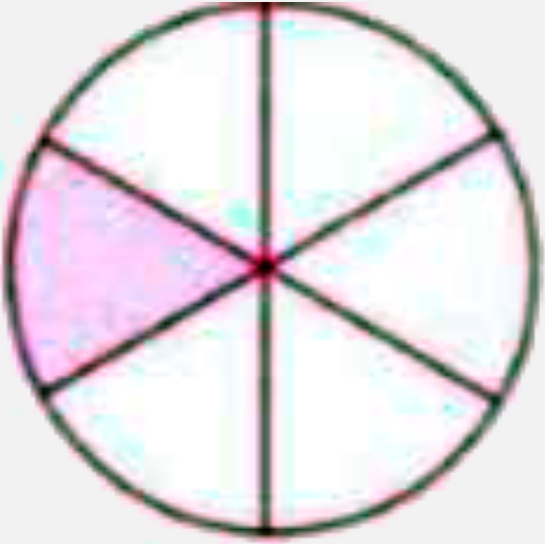 View Solution
View SolutionWrite the fraction of the shaded portion in the following figures.
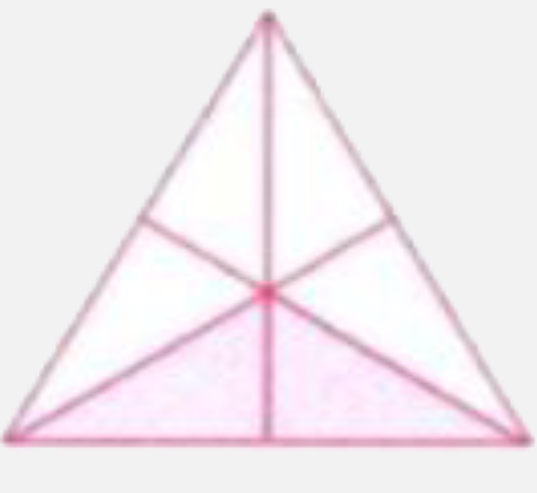 View Solution
View SolutionA uniform circular disc of mass M and radius R is pivoted at distance x above the centre of mass of the disc, such that the time period of the disc in the vertical plane is infinite. What is the distance between the pivoted point and centre of mass of the disc ?
View Solution

