The capacity of a parallel plate condenser without any dielectric is C. If the distance between the plates is doubled and the space between the plates is filled with a substance of dielectric constant 3, the capacity of the condenser becomes:
The correct Answer is:D
To solve the problem, we need to analyze how the capacitance of a parallel plate capacitor changes when the distance between the plates is doubled and a dielectric material is introduced.
1. Understand the formula for capacitance:
The capacitance C of a parallel plate capacitor without a dielectric is given by the formula:
C=ε0AD
where ε0 is the permittivity of free space, A is the area of the plates, and D is the distance between the plates.
2. Identify the initial conditions:
The initial capacitance is given as C. Therefore, we can express it as:
C=ε0AD
3. Change in distance:
According to the problem, the distance between the plates is doubled. Thus, the new distance D′ becomes:
D′=2D
4. Introduce the dielectric:
The space between the plates is filled with a dielectric material that has a dielectric constant K=3. The new permittivity ε′ of the capacitor becomes:
ε′=K⋅ε0=3ε0
5. Calculate the new capacitance:
The new capacitance C′ with the dielectric and the new distance can be expressed as:
C′=ε′AD′=3ε0A2D
6. Relate the new capacitance to the original capacitance:
We can substitute the original capacitance C into the equation:
C′=3ε0A2D=32⋅ε0AD=32C
7. Final result:
Thus, the new capacitance C′ of the capacitor is:
C′=32C
Conclusion:
The capacity of the condenser after doubling the distance and filling it with a dielectric of constant 3 becomes 32C.
---
The capacitance C of a parallel plate capacitor without a dielectric is given by the formula:
C=ε0AD
where ε0 is the permittivity of free space, A is the area of the plates, and D is the distance between the plates.
The initial capacitance is given as C. Therefore, we can express it as:
C=ε0AD
According to the problem, the distance between the plates is doubled. Thus, the new distance D′ becomes:
D′=2D
The space between the plates is filled with a dielectric material that has a dielectric constant K=3. The new permittivity ε′ of the capacitor becomes:
ε′=K⋅ε0=3ε0
The new capacitance C′ with the dielectric and the new distance can be expressed as:
C′=ε′AD′=3ε0A2D
We can substitute the original capacitance C into the equation:
C′=3ε0A2D=32⋅ε0AD=32C
Thus, the new capacitance C′ of the capacitor is:
C′=32C
The capacity of the condenser after doubling the distance and filling it with a dielectric of constant 3 becomes 32C.
Topper's Solved these Questions
Similar Questions
The capacity of a parallel plate condenser depends upon
View SolutionA parallel plate capacitor with air between the plates has a capacitance C. If the distance between the plates is doubled and the space between the plates is filled with a dielectric of dielectric constant 6, then the capacitance will become.
View Solution
Knowledge Check
A parallel plate capacitor with air between the plates has a capacitance of 10 pF. The capacitance, if the distance bgetween the plates is reduced by half and the space between tehm is filled with a substance of dielectric constant 4 is
A80pFB96pFC100pFD120pF
A dielectric slab is inserted between the plates of an isolated capacitor. The force between the plates will
View SolutionWhen a dielectric slab is gradually inserted between the plates of an isolated parallel-plate capacitor , the energy of the system decreases.What can you conclude about the force on the slab exerted by the electric field?
View SolutionConsider a disconnected plate capacitor of capacity 10μF with air filled in the gap between the plates. Now one-half of the space between the plates is filled with a dielectric of dielectric constant 4 as shown in .The capacity of the capacitor changes to
 .
.
View SolutionConsider a parallel plate capacitor of 10μF (micro-farad) with air filled in the gap between the plates. Now one half of the space between the plates is filled with a dielectric of dielectric constant 4, as shown in the figure. The capacity of the capacitor charges to
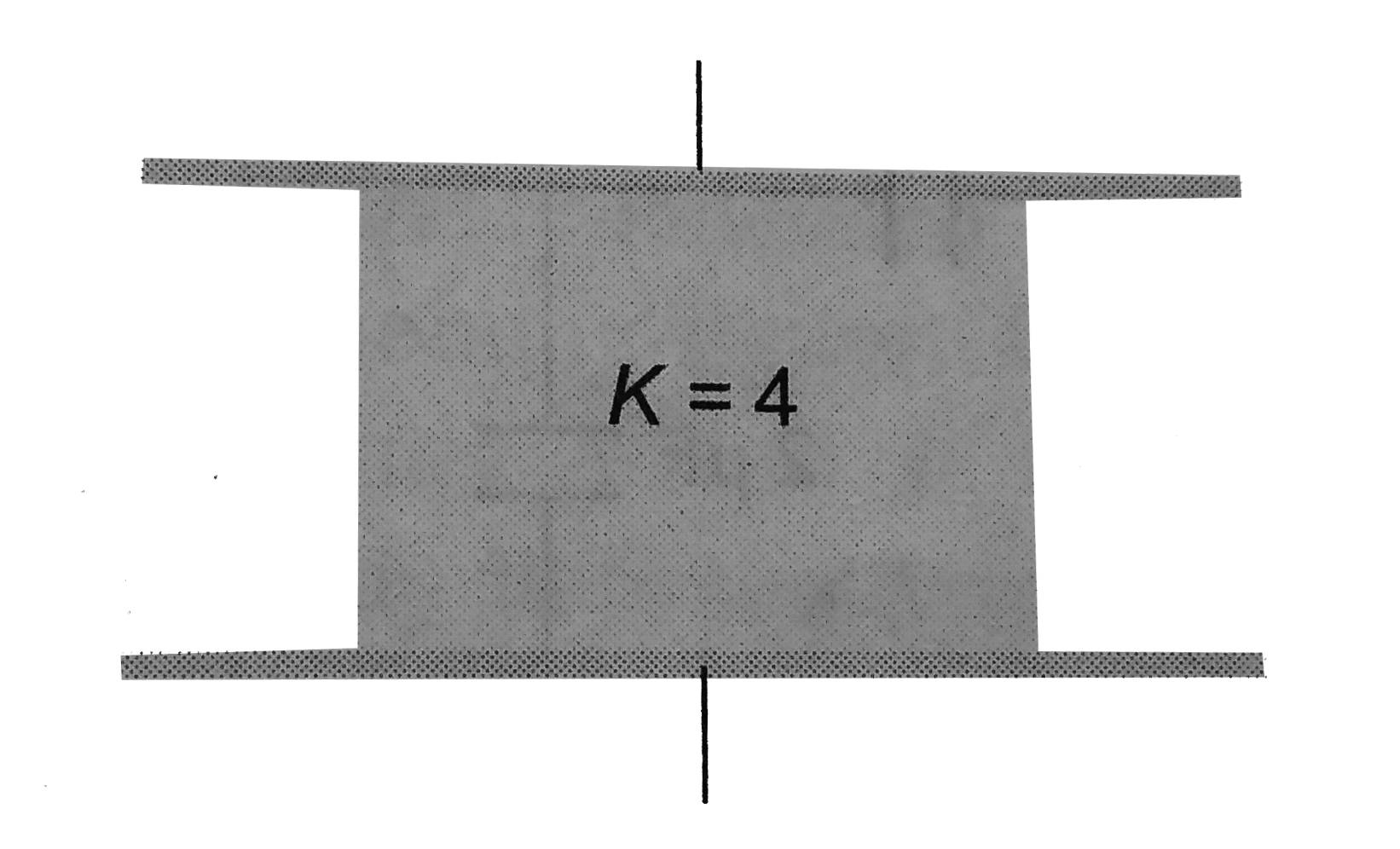
View SolutionA parallel plate capacitor with air between the plates has a capacitance of 8 pF.(1pF=10−12F) What will be the capacitance if the distance between the plates is reduced by half and the space between them is filled with a substance of dielectric constant 6 ?
View SolutionParallel Plate Capacitors with and without Dielectric
View SolutionA parallel plate condenser has a unifrom electric field E(V/m) in the space between the plates. If the distance between the plates is d(m) and area of each plate is A(m2) the energy (joule) stored in the condenser is
View Solution