The resistance of a coil is 5 ohm and a current of 0.2A is induced in it due to a varying magnetic field. The rate of change of magnetic flux in it will be-
0.5 W/b
0.05 Wb/s
1 W b/s
20 Wb/s
The correct Answer is:C
To solve the problem, we need to find the rate of change of magnetic flux (dΦ/dt) in the coil given its resistance (R) and the induced current (I).
1. Identify the given values:
- Resistance of the coil, R=5Ω
- Induced current, I=0.2A
2. Use Ohm's Law to find the electromotive force (emf):
The relationship between current, resistance, and emf is given by Ohm's Law:
emf=I×R
Substituting the known values:
emf=0.2A×5Ω=1V
3. Relate emf to the rate of change of magnetic flux:
According to Faraday's law of electromagnetic induction, the emf (ε) induced in a coil is also equal to the rate of change of magnetic flux through the coil:
emf=dΦdt
Therefore, we can write:
dΦdt=1Wb/s
4. Conclusion:
The rate of change of magnetic flux in the coil is:
dΦdt=1Wb/s
Final Answer:
The rate of change of magnetic flux in the coil is 1Wb/s.
---
- Resistance of the coil, R=5Ω
- Induced current, I=0.2A
The relationship between current, resistance, and emf is given by Ohm's Law:
emf=I×R
Substituting the known values:
emf=0.2A×5Ω=1V
According to Faraday's law of electromagnetic induction, the emf (ε) induced in a coil is also equal to the rate of change of magnetic flux through the coil:
emf=dΦdt
Therefore, we can write:
dΦdt=1Wb/s
The rate of change of magnetic flux in the coil is:
dΦdt=1Wb/s
The rate of change of magnetic flux in the coil is 1Wb/s.
Topper's Solved these Questions
Similar Questions
Knowledge Check
By a change of current from 5 A to 10 A in 0.1 s, the self induced emf is 10 V. The change in the energy of the magnetic field of a coil will be
A5JB6JC7.5JD9JBy a change of current from 5 A to 10 A in 0.1 s, the self induced emf is 10 V. The change in the energy of the magnetic field of a coil will be
A5JB6JC7.5JD9JCurrent flowing through a long solenoid is varied. Then, magnetic flux density of the magnetic field inside it varies :
Ainversely with IBinversely withI2Cdirectly with IDdirectly with I2
The magnetic field at the centre of the current carrying coil is
View SolutionMagnetic Field due to Circular Coil
View SolutionIn a coil of resistance 100Ω , a current is induced by changing the magnetic flux through it as shown in the figure. The magnitude of change in flux through the coil is
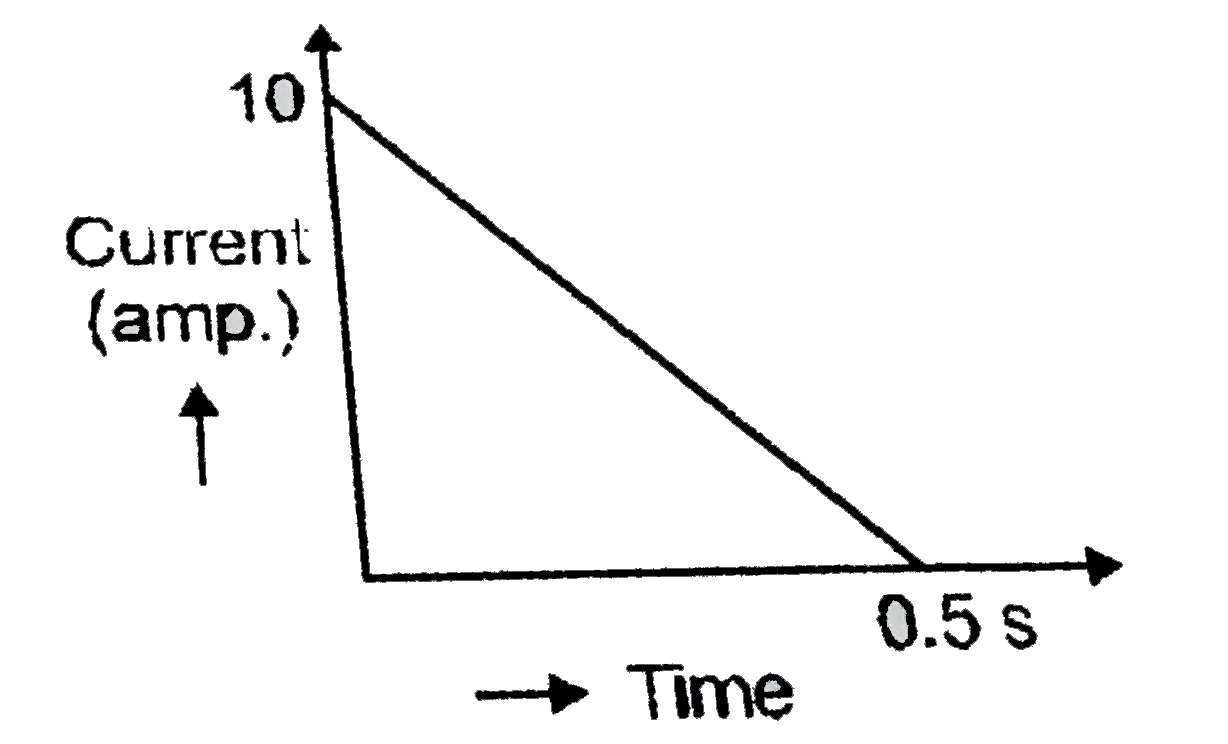
View SolutionA magnet is taken towards a conducting ring in such a way that a constant current of 10mA is induced in it. The total resistance of the ring is 0.5Ω. In 5s, the magnetic flux through the ring changes by
View SolutionA magnet is taken towards a conducting ring in such a way that a constant current of 10mA is induced in it. The total resistance of the ring is 0.5Ω. In 5s, the magnetic flux through the ring changes by
View Solution