If the unknown resistance calculated without using the end correction, is and with using the end corrections is then (assume same end correction at both ends)
when balanced point is in first half
when balanced point is in first half
when balanced point is in seconds half
always
The correct Answer is:B
To solve the problem, we need to analyze the relationship between the unknown resistances R1 and R2 calculated with and without end corrections, respectively.
1. Understanding the Problem:
- We have two resistances: R1 (calculated without end correction) and R2 (calculated with end correction).
- We are to assume that the same end correction is applied at both ends of the potentiometer wire.
2. Formulating the Expressions:
- The resistance R1 can be expressed in terms of the length L of the wire and the sensitivity S of the potentiometer:
R1=S⋅L100−L
- When considering the end correction, let’s denote the end correction as X. The effective length when the end correction is applied would be L+X. Thus, the expression for R2 becomes:
R2=S⋅L+X100−(L+X)
3. Analyzing the Two Cases:
- First Half: If L is in the first half of the potentiometer (e.g., L=30):
R1=S⋅30100−30=S⋅3070
R2=S⋅30+X100−(30+X)=S⋅30+X70−X
Here, R2 will be greater than R1 if X is positive.
- Second Half: If L is in the second half of the potentiometer (e.g., L=70):
R1=S⋅70100−70=S⋅7030
R2=S⋅70+X100−(70+X)=S⋅70+X30−X
Here, R1 will be greater than R2 if X is positive.
4. Conclusion:
- From the analysis, we can conclude:
- In the first half of the potentiometer, R2>R1.
- In the second half of the potentiometer, R1>R2.
Final Answer:
Thus, the relationship between R1 and R2 depends on the position of L along the potentiometer wire.
- We have two resistances: R1 (calculated without end correction) and R2 (calculated with end correction).
- We are to assume that the same end correction is applied at both ends of the potentiometer wire.
- The resistance R1 can be expressed in terms of the length L of the wire and the sensitivity S of the potentiometer:
R1=S⋅L100−L
- When considering the end correction, let’s denote the end correction as X. The effective length when the end correction is applied would be L+X. Thus, the expression for R2 becomes:
R2=S⋅L+X100−(L+X)
- First Half: If L is in the first half of the potentiometer (e.g., L=30):
R1=S⋅30100−30=S⋅3070
R2=S⋅30+X100−(30+X)=S⋅30+X70−X
Here, R2 will be greater than R1 if X is positive.
R1=S⋅70100−70=S⋅7030
R2=S⋅70+X100−(70+X)=S⋅70+X30−X
Here, R1 will be greater than R2 if X is positive.
- From the analysis, we can conclude:
- In the first half of the potentiometer, R2>R1.
- In the second half of the potentiometer, R1>R2.
Thus, the relationship between R1 and R2 depends on the position of L along the potentiometer wire.
Similar Questions
If the unknown Resistancee calculated without using the end correction is R1 and with using end corrections is R2 then (assume same end corection) .
View SolutionWhat is meant by the end correction ?
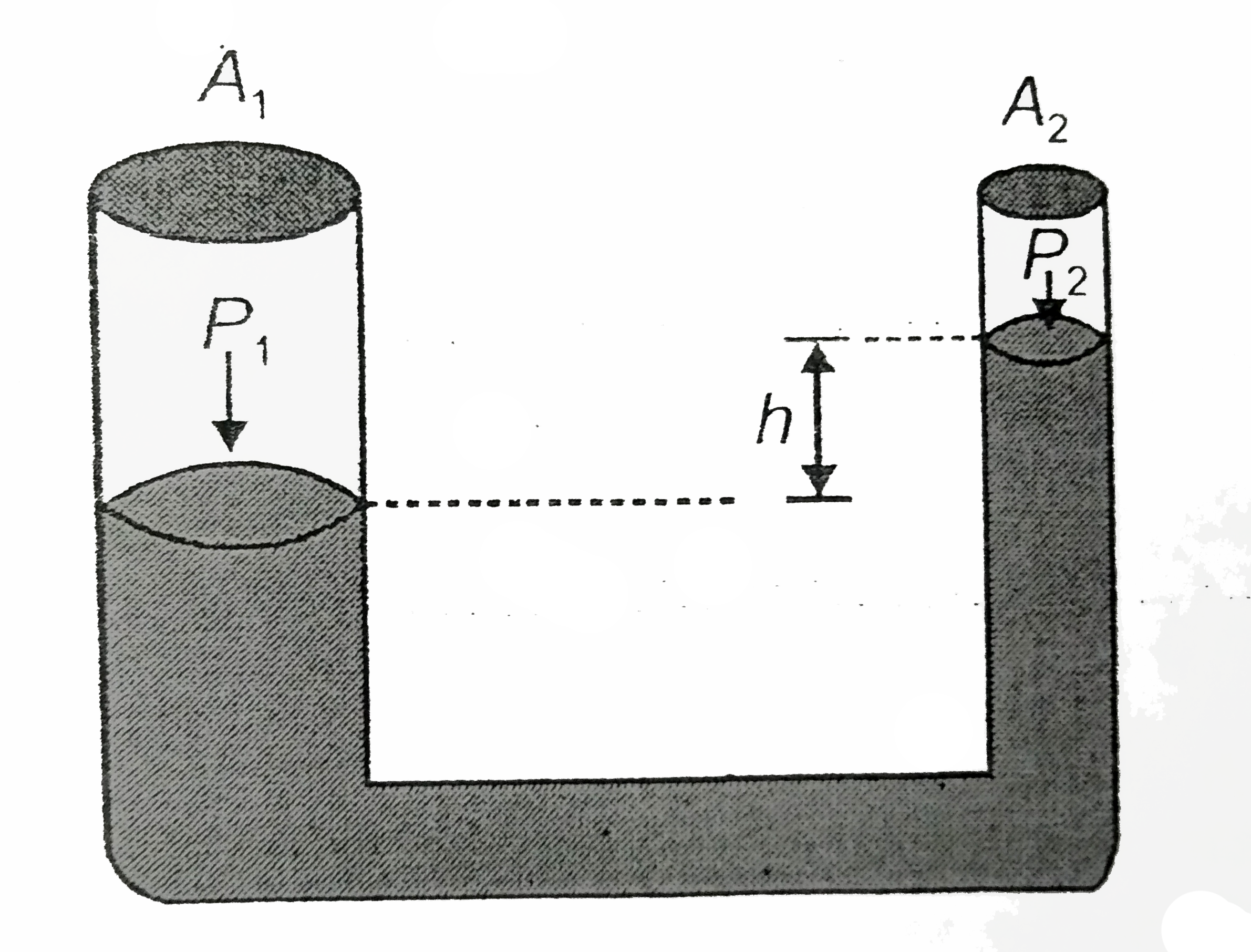
View SolutionIn the adjoing figure P1andP2 are the pressures in broad and narrow tubes with closed ends. Select the correct option
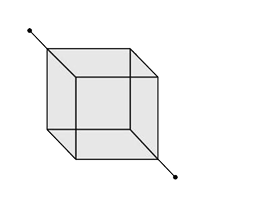
View SolutionTwelve wires of equal length and same cross-section are connected in the form of a cube. If the resistance of each of the wires is R, and the effective resistance between the two diagonal ends is Re, then the ratio RRe is equal to __.
View SolutionIf the end correction of an open pipe is 0.8 cm, then the inner radius of that pipe will be
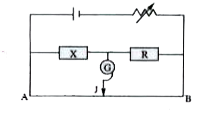
View SolutionConsider the meter bridge circuit without neglecting and corrections (α, β)
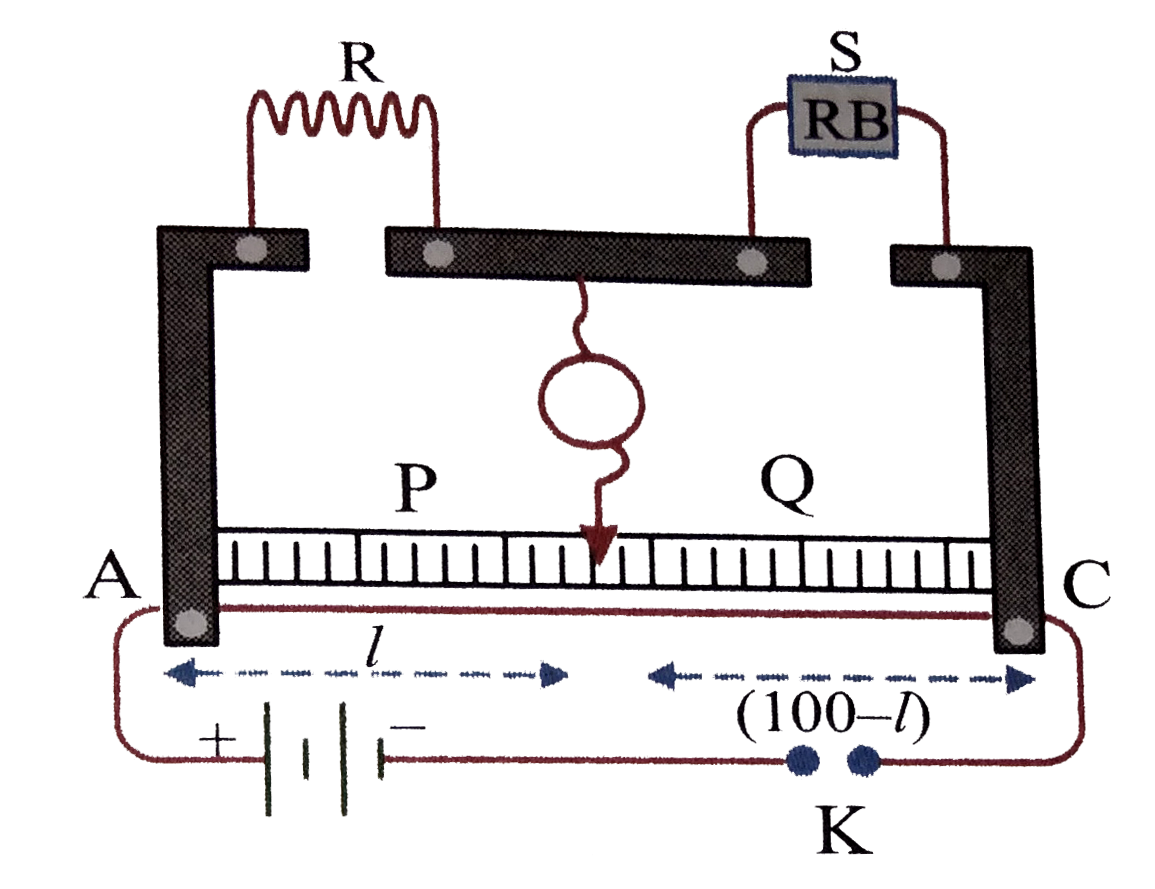
Now start taking obsevation. At the position of R, unknown resistance is used, and at position of S, 300Ω resistance is used. If the balanced length was found to be l=26cm, then estimate the unknown resistance.
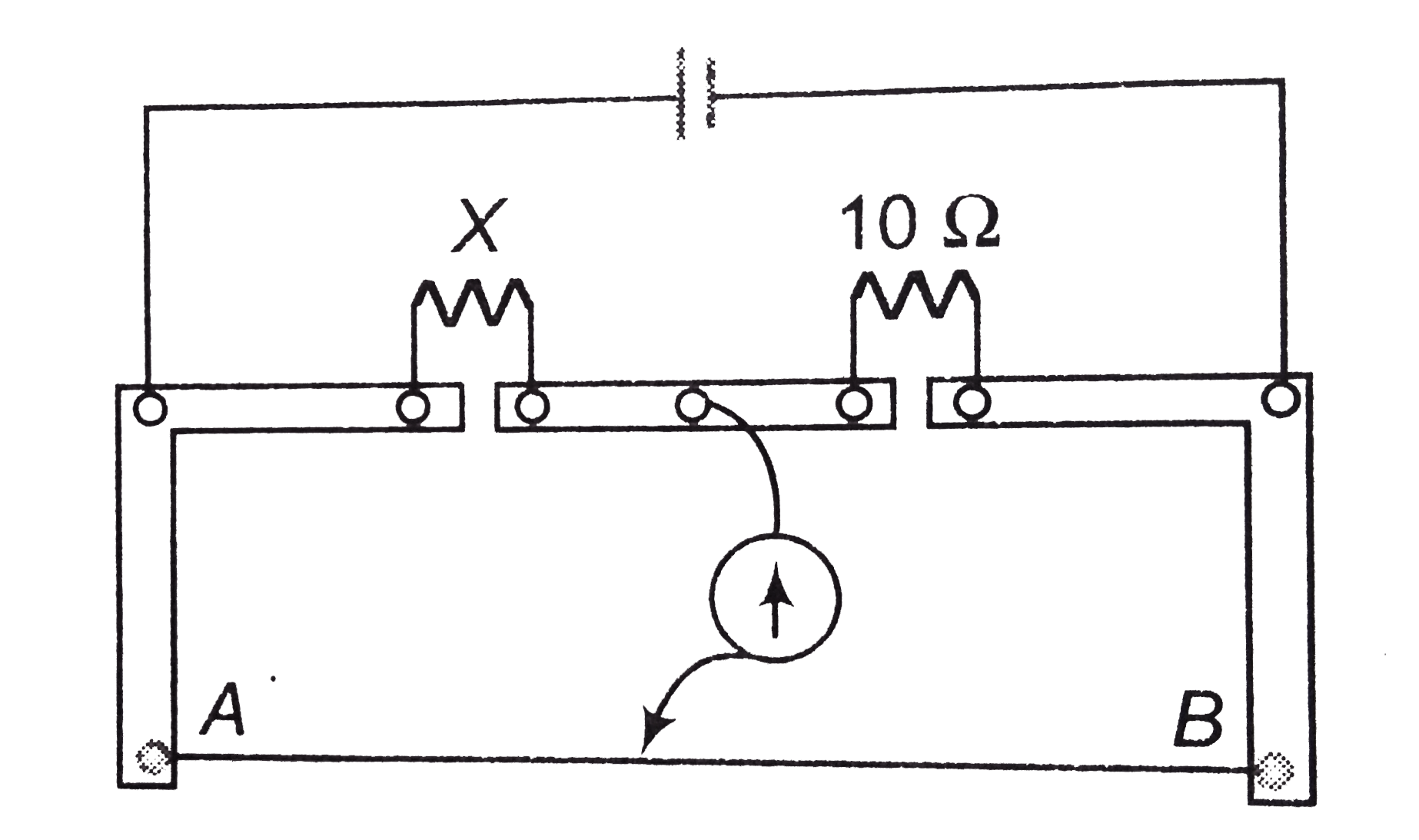
View SolutionA meter bridge is setup as shown in figure, to determine an unknown resistance X using a standard 10Ω resistor. The galvanometer shows null point when tapping key is at 52cm mark. The end correctiosnn are 1cm and 2cm respectively for the ends A and B. The determined value of X is
View SolutionFigure shows a metre bridge in which a known resistance R and an unknown resistance X connected to it. The length of the total wire AB is 100cm. Then the balancing length for which the percentage error in measuring the value of x is minimum (neglect end corrections)
View SolutionThe frequency of the fundamental note of a tube closed at one end is 200 Hz. What will be the frequency of the fundamental note of a similar tube of the same length but open at both ends ?
View SolutionA meter bridge is set- up as shown in figure, to determine an unknown resistance X using a standard 10Ω resistor. The galvanometer shows null point when tapping - key is at 52cm mark. The end -corrections are 1m and 2cm respectively for the ends A and B. The determined values of X is .
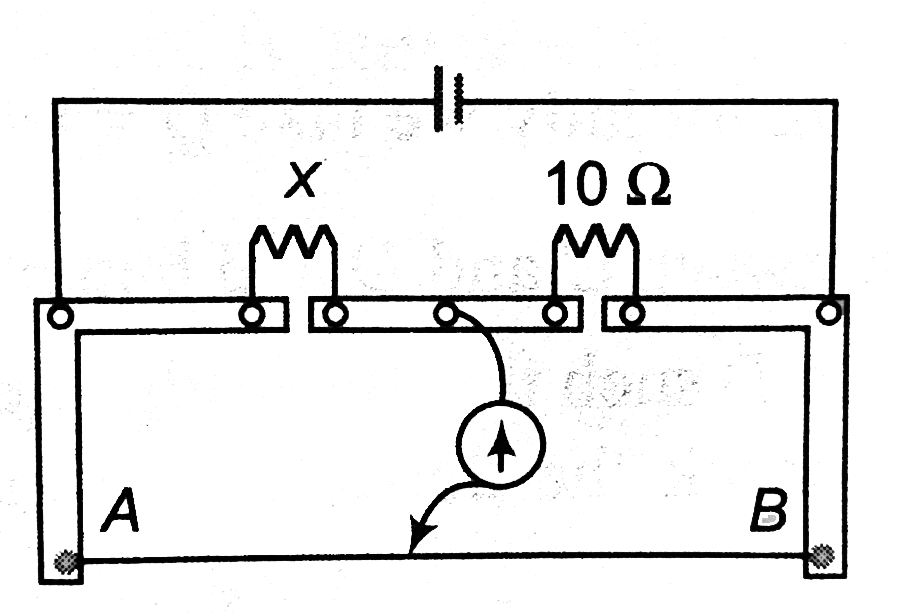
(a) 10.2Omeaga
(b) 10.6Ω
(c) 10.8Ω
(d) 11.1Ω.
View Solution
