For the wave y= 3.0 sin(36t + 0.018x + pi/4) plot the displacement (y) versus (t) graphs for x=0,. What is the shaps of the graph ? In which aspects does the oscillatory motion in travelling wave differ from one point to another amplitude, frequency or phase?
The correct Answer is:All the graphs are sinusoidal. They have same amplitude and frequency, but different initial phases.
To solve the problem, we will follow these steps:
Step 1: Identify the parameters of the wave equation
The given wave equation is:
y=3.0sin(36t+0.018x+π4)
Here, we can identify:
- Amplitude A=3.0
- Angular frequency ω=36rad/s
- Phase constant ϕ=π4
Step 2: Substitute x=0 into the wave equation
When x=0, the wave equation simplifies to:
y=3.0sin(36t+π4)
Step 3: Calculate the values of y at different time intervals
To plot the graph, we need to calculate the displacement y at various time intervals. We will use the period T to find these intervals.
Step 3.1: Calculate the period T
The relationship between angular frequency ω and the period T is given by:
ω=2πT
Thus,
T=2πω=2π36=π18seconds
Step 3.2: Choose time intervals
We will choose the following time intervals:
- t=0
- t=T8
- t=2T8
- t=3T8
- t=4T8
- t=5T8
- t=6T8
- t=7T8
- t=T
Calculating T/8:
T8=π/188=π144
Step 4: Calculate y for each time interval
Now we will calculate y for each time interval:
1. t=0:
y=3.0sin(0+π4)=3.0⋅1√2=3√2≈2.12
2. t=T8:
y=3.0sin(36⋅π144+π4)=3.0sin(π2)=3.0
3. t=2T8:
y=3.0sin(36⋅2π144+π4)=3.0sin(3π4)=3.0⋅1√2≈2.12
4. t=3T8:
y=3.0sin(36⋅3π144+π4)=3.0sin(π)=0
5. t=4T8:
y=3.0sin(36⋅4π144+π4)=3.0sin(5π4)=−3.0⋅1√2≈−2.12
6. t=5T8:
y=3.0sin(36⋅5π144+π4)=3.0sin(−π2)=−3.0
7. t=6T8:
y=3.0sin(36⋅6π144+π4)=3.0sin(−3π4)=−3.0⋅1√2≈−2.12
8. t=7T8:
y=3.0sin(36⋅7π144+π4)=3.0sin(−π)=0
9. t=T:
y=3.0sin(36⋅2π18+π4)=3.0sin(5π4)=−3.0⋅1√2≈−2.12
Step 5: Plot the graph
Now we can plot the values of y against t:
- At t=0, y≈2.12
- At t=T8, y=3.0
- At t=2T8, y≈2.12
- At t=3T8, y=0
- At t=4T8, y≈−2.12
- At t=5T8, y=−3.0
- At t=6T8, y≈−2.12
- At t=7T8, y=0
- At t=T, y≈−2.12
Final Shape of the Graph
The shape of the graph is sinusoidal, oscillating between the maximum amplitude of 3.0 and the minimum amplitude of −3.0.
Step 6: Analyze the differences in oscillatory motion
In a traveling wave, the oscillatory motion can differ from one point to another in terms of:
- Phase: Different points in space may have different phases of oscillation.
- Amplitude: In some cases, amplitude can vary with position, but in this case, it remains constant.
- Frequency: The frequency remains constant throughout the wave.
The given wave equation is:
y=3.0sin(36t+0.018x+π4)
- Amplitude A=3.0
- Angular frequency ω=36rad/s
- Phase constant ϕ=π4
When x=0, the wave equation simplifies to:
y=3.0sin(36t+π4)
To plot the graph, we need to calculate the displacement y at various time intervals. We will use the period T to find these intervals.
The relationship between angular frequency ω and the period T is given by:
ω=2πT
Thus,
T=2πω=2π36=π18seconds
We will choose the following time intervals:
- t=0
- t=T8
- t=2T8
- t=3T8
- t=4T8
- t=5T8
- t=6T8
- t=7T8
- t=T
T8=π/188=π144
Now we will calculate y for each time interval:
y=3.0sin(0+π4)=3.0⋅1√2=3√2≈2.12
y=3.0sin(36⋅π144+π4)=3.0sin(π2)=3.0
y=3.0sin(36⋅2π144+π4)=3.0sin(3π4)=3.0⋅1√2≈2.12
y=3.0sin(36⋅3π144+π4)=3.0sin(π)=0
y=3.0sin(36⋅4π144+π4)=3.0sin(5π4)=−3.0⋅1√2≈−2.12
y=3.0sin(36⋅5π144+π4)=3.0sin(−π2)=−3.0
y=3.0sin(36⋅6π144+π4)=3.0sin(−3π4)=−3.0⋅1√2≈−2.12
y=3.0sin(36⋅7π144+π4)=3.0sin(−π)=0
y=3.0sin(36⋅2π18+π4)=3.0sin(5π4)=−3.0⋅1√2≈−2.12
Now we can plot the values of y against t:
- At t=0, y≈2.12
- At t=T8, y=3.0
- At t=2T8, y≈2.12
- At t=3T8, y=0
- At t=4T8, y≈−2.12
- At t=5T8, y=−3.0
- At t=6T8, y≈−2.12
- At t=7T8, y=0
- At t=T, y≈−2.12
The shape of the graph is sinusoidal, oscillating between the maximum amplitude of 3.0 and the minimum amplitude of −3.0.
In a traveling wave, the oscillatory motion can differ from one point to another in terms of:
- Phase: Different points in space may have different phases of oscillation.
- Amplitude: In some cases, amplitude can vary with position, but in this case, it remains constant.
- Frequency: The frequency remains constant throughout the wave.
Topper's Solved these Questions
- SOUND WAVESBOOK - RESONANCE ENGLISHCHAPTER - SOUND WAVESEXERCISE - Exercise- 1 PART - I
34 Videos
- SOUND WAVESBOOK - RESONANCE ENGLISHCHAPTER - SOUND WAVESEXERCISE - Exercise- 1 PART - II
30 Videos
- SOUND WAVESBOOK - RESONANCE ENGLISHCHAPTER - SOUND WAVESEXERCISE - Solved Examples
24 Videos
- SIMPLE HARMONIC MOTIONBOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise
28 Videos
- STRING WAVESBOOK - RESONANCE ENGLISHCHAPTER - STRING WAVESEXERCISE - Exercise
32 Videos
Similar Questions
For the harmonic travelling wave y=2cos2pi(10t-0.0080x+3.5) where x and y ar in cm and t is in second. What is the phase diffference between the oscillatory motion at two points separated by a distance of
View SolutionA particle is moving in x−y plane with y=x2 and Vx=4−2t. The displacement versus time graph of the particle would be
View Solution
Knowledge Check
For the travelling harmonic wave
y(x, t) = 2 cos2π(10t - 0.008x + 0.35) where X and Y are in cm and t is in s. The phase difference between oscillatory motion of two points separated by distance of 0.5 m isA0.2πradB0.4πradC0.6πradD0.8πrad
The equation of a travelling sound wave is y=6.0sin(600t−1.8x) where y is measured in 10−5m, t in second and x in metre. (a) Find the ratio of the displacement amplitude of the particles to the wavelength of the wave. (b) Find the ratio of the velocity amplitude of the particles to the wave speed.
View SolutionThe graph shows a wave at t=0 travelling to the right with a velocity of 4m/s. The equation that best represents the wave is
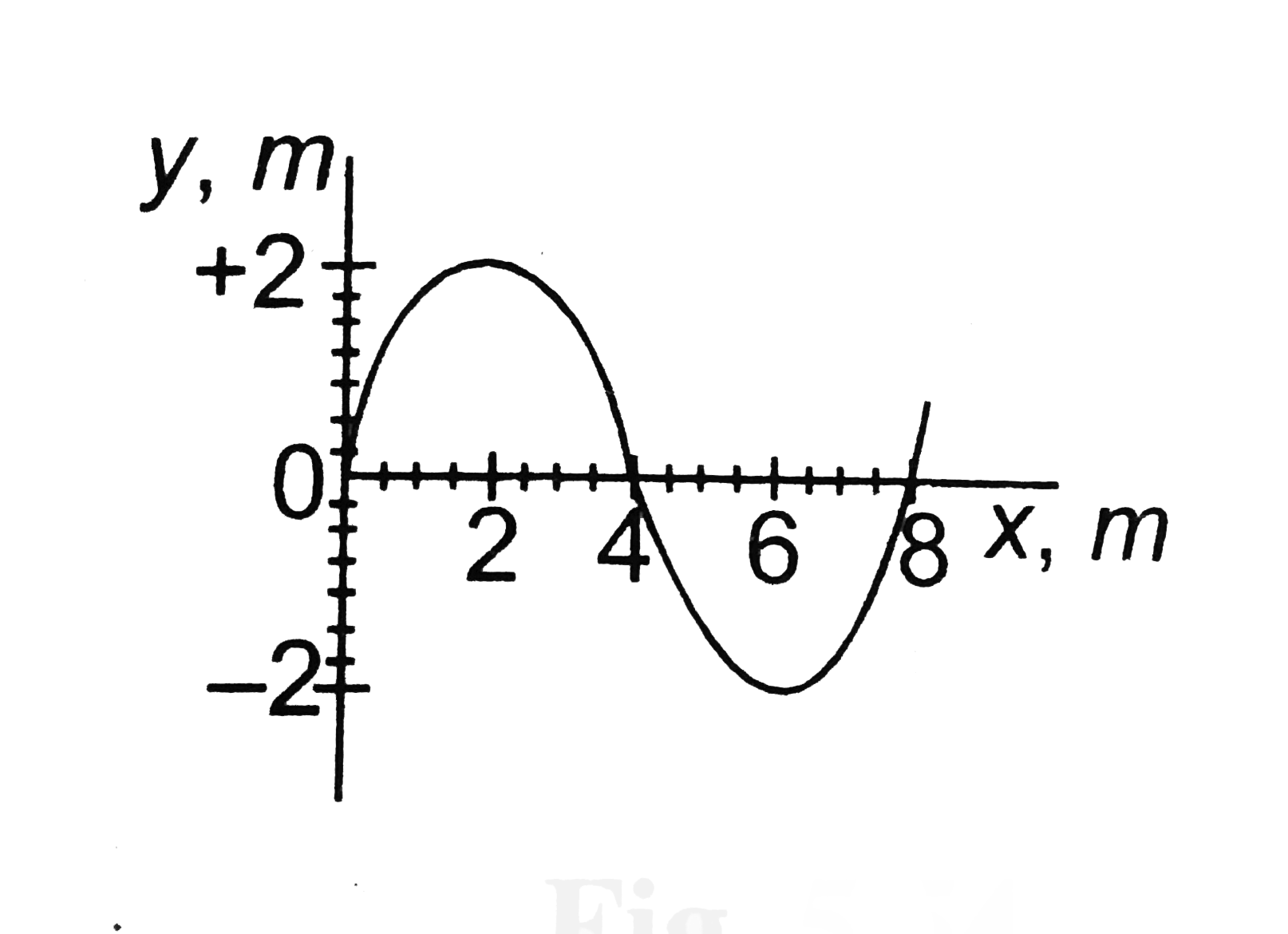
View SolutionA transverse harmonic wave on a string is described by y(x,t)=3.0sin(36t+0.018x+π/4) where x, y are in cm, t in second. The positive direction of x is from left to right . (i) Is this a travelling or stationary wave ? If travellying, what are the speed and direction of its propagation ? (ii) what are its amplitude and frequency ? (iii) what is the inital phase at the origing ? (iv) What is the least distance between two successive creests in the wave?
View SolutionThe displacement equation of a wave is y = 0.5 sin π(2+−0.01x) where x is in meter and t is in second. Find the frequency, amplitude, speeds and phase difference between two particles situated at a distance of 5 m from each other.
View SolutionFor the wave y=5sin30π[t−(x/240)], where xandy are in cm and t is in seconds, find the
(a) displacement when t=0 and x=2cm
(b) wavelength
( c ) velocity of the wave and
(d) frequency of the wave
View SolutionFor a travelling harmonic wave y=2.0cos(10t−0.0080x+0.35), where x and y are in centimetres and t in seconds. What is the phase difference between oscillatory motion of two points separated by a distance of (a) 4m (b) 0.5 m (c ) λ/2 (d) 3λ/4
View SolutionFor a travelling harmonic wave y=2.0cos(10t−0.0080x+0.35), where x and y are in centimetres and t in seconds. What is the phase difference between oscillatory motion of two points separated by a distance of (a) 4cm (b) 0.5 cm (c ) λ/2 (d) 3λ/4
View Solution
