A cylinder is filled with a liquid of refractive index μ. The radius of the cylinder is decreasing at a constant rate K. The volume of the liquid inside the container remains constant at V. The observer and the object O are in a state of rest at a distance L from each other. When radius of cylinder is r the apparent velocity of the object as seen by the observer is 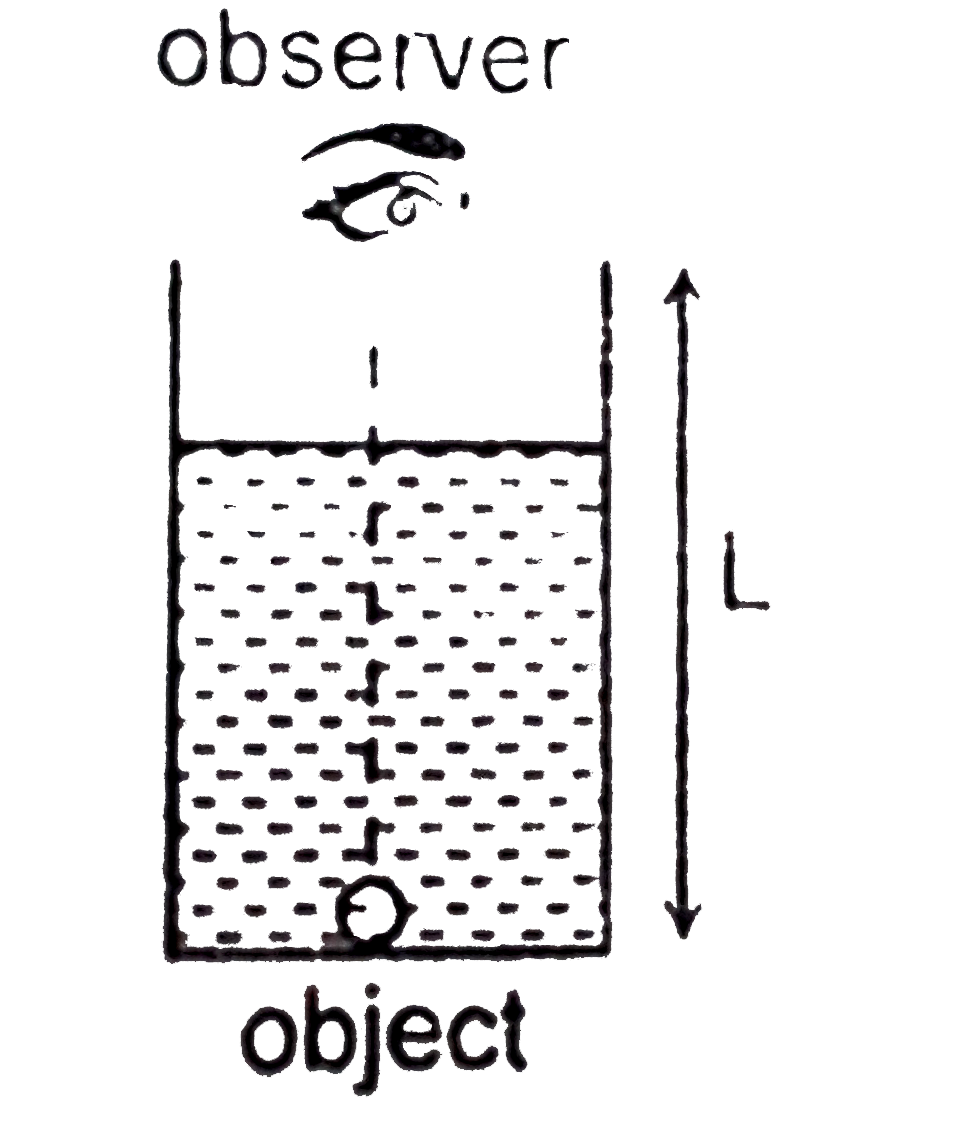
(1−μ)2KV(πμr3)
(1−μ)2KV(πμlr2)
(1−μ)2Kμ
(1−μ)K2μ
The correct Answer is:A
Xapp=L−H+Hμ 
dXappdt=dHdt(1−μμ)
πr2H=V
∴dHdt=−2Hrdrdt+2KHr
∴dXappdt=2KVπr3((1−μ)μ)
Topper's Solved these Questions
Similar Questions
The radius of a cylinder is r and heigth is h. Find its volume.
View SolutionIf the radius of a cylinder is doubled and height is halved, the volume will be doubled.
View SolutionA cubic container is filled with a liquid whose refractive index increases linearly from top to bottom. Which of the following represents the path of a ray of light inside the liquid ?
View SolutionA cylindrical space of radius R is filled with a uniform magnetic induction parallel to the axis of the cylinder. If B charges at a constant rate, the graph showin the variation of induced electric field with distance r from the axis of cylinder is
 View Solution
View SolutionA vessel is quarter filled with a liquid of refractive index μ. The remaining parts of the vessel is filled with an immiscible liquid of refractive index 3μ/2. The apparent depth of the vessel is 50% of the actual depth. The value of μ is
View SolutionFind the volume of the larges cylinder that can be inscribed in a sphere of radius r
View SolutionThe radius of a cylinder is increasing at the rate 2cm/sec. and its altitude is decreasing at the rate of 3cm/sec. Find the rate of change of volume when radius is 3 cm and altitude 5 cm.
View SolutionThe radius of a cylinder is increasing at the rate 2cm/sec. and its altitude is decreasing at the rate of 3cm/sec. Find the rate of change of volume when radius is 3 cm and altitude 5 cm.
View SolutionFind the volume of the largest cylinder that can be inscribed in a sphere of radius r
View SolutionThe radius and height of a cylinder are equal. If the radius of the sphere is equal to the height of the cylinder, then the ratio of the rates of increase of the volume of the sphere and the volume of the cylinder, is
View Solution

