A man is standing on a platform moving up and down as a S.H.M. will there be any change in his weight as recorded by a weighing machine on the platform ?
To solve the question of whether there will be any change in the weight of a man standing on a platform moving up and down in Simple Harmonic Motion (SHM) as recorded by a weighing machine, we can follow these steps:
1. Understanding the System:
- The man is standing on a platform that oscillates in SHM about a mean position. The platform moves up and down, which means it experiences acceleration at different points in its motion.
2. Identifying Forces:
- The weight of the man is given by mg, where m is the mass of the man and g is the acceleration due to gravity.
- The normal force N exerted by the weighing machine on the man will vary depending on the acceleration of the platform.
3. Acceleration in SHM:
- In SHM, the acceleration a of the platform at a displacement x from the mean position is given by:
a=−ω2x
- Here, ω is the angular frequency of the motion.
4. Applying Newton's Second Law:
- When the platform moves upward, the effective weight (normal force) recorded by the weighing machine can be expressed as:
N=mg+ma
- Substituting the expression for acceleration, we get:
N=mg+m(−ω2x)=mg−mω2x
- Conversely, when the platform moves downward, the normal force becomes:
N′=mg−ma=mg−m(−ω2x)=mg+mω2x
5. Conclusion:
- The normal force N (the weight recorded by the weighing machine) varies depending on the position of the platform (whether it is at the maximum displacement upward or downward).
- Therefore, as the platform oscillates, the reading on the weighing machine will change, indicating that the apparent weight of the man changes due to the acceleration of the platform.
Final Answer:
Yes, there will be a change in the weight recorded by the weighing machine on the platform as it moves up and down in SHM. The weight will vary depending on the acceleration of the platform at any given instant.
- The man is standing on a platform that oscillates in SHM about a mean position. The platform moves up and down, which means it experiences acceleration at different points in its motion.
- The weight of the man is given by mg, where m is the mass of the man and g is the acceleration due to gravity.
- The normal force N exerted by the weighing machine on the man will vary depending on the acceleration of the platform.
- In SHM, the acceleration a of the platform at a displacement x from the mean position is given by:
a=−ω2x
- Here, ω is the angular frequency of the motion.
- When the platform moves upward, the effective weight (normal force) recorded by the weighing machine can be expressed as:
N=mg+ma
- Substituting the expression for acceleration, we get:
N=mg+m(−ω2x)=mg−mω2x
- Conversely, when the platform moves downward, the normal force becomes:
N′=mg−ma=mg−m(−ω2x)=mg+mω2x
- The normal force N (the weight recorded by the weighing machine) varies depending on the position of the platform (whether it is at the maximum displacement upward or downward).
- Therefore, as the platform oscillates, the reading on the weighing machine will change, indicating that the apparent weight of the man changes due to the acceleration of the platform.
Yes, there will be a change in the weight recorded by the weighing machine on the platform as it moves up and down in SHM. The weight will vary depending on the acceleration of the platform at any given instant.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 1, PART - I
25 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 1, PART - II
36 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Solved Miscellaneous Problems
9 Videos
- SEMICONDUCTORSBOOK - RESONANCE ENGLISHCHAPTER - SEMICONDUCTORSEXERCISE - Exercise 3
88 Videos
- TEST PAPERSBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PHYSICS
784 Videos
Similar Questions
A man of mass m=2kg is standing on a platform of mass M=5kg, then at any instant.
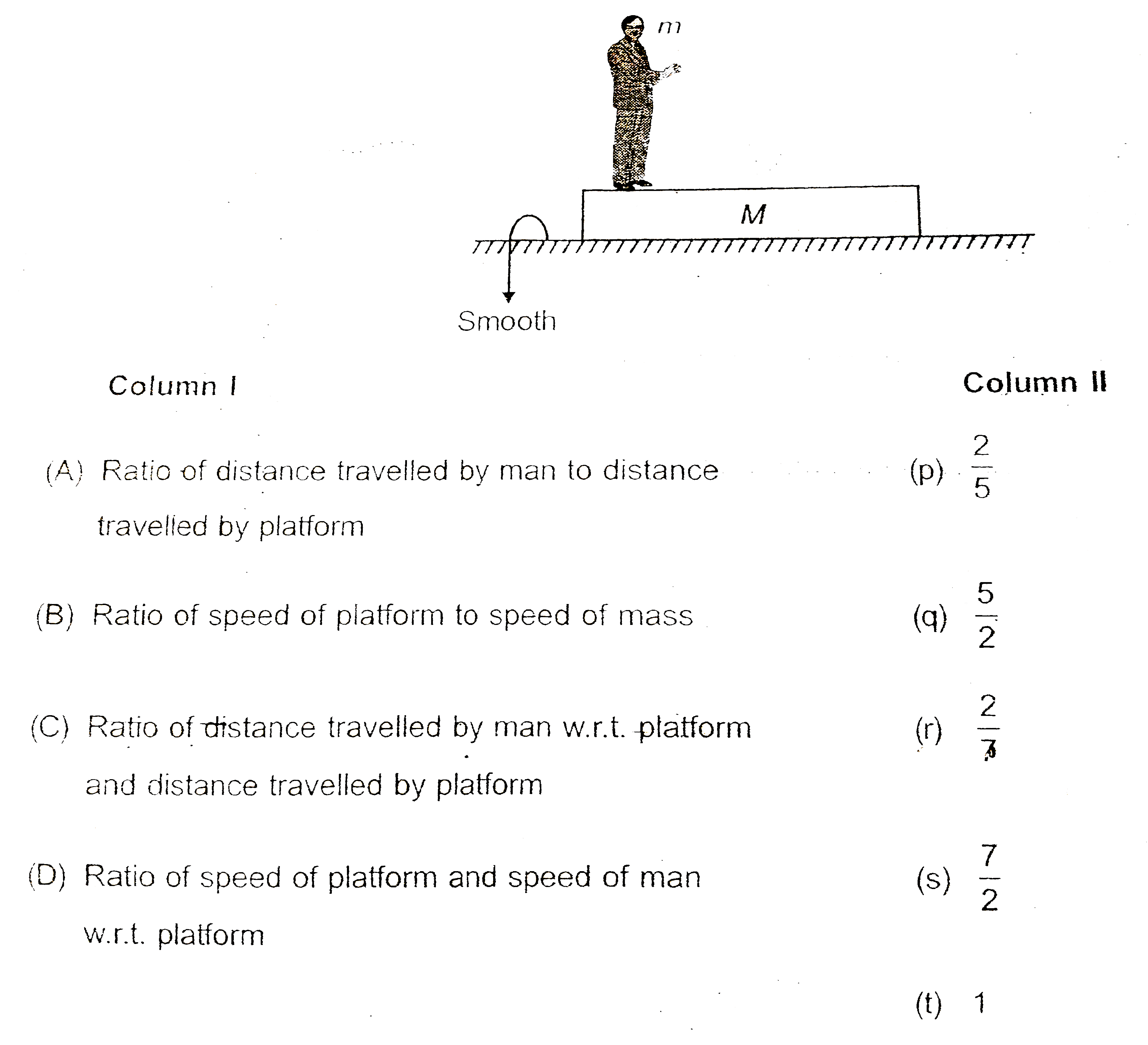
View SolutionA man of mass m is standing on a platform of mass M kept on smooth ice. If the man starts moving on the platform with a speed v relative to the platform with what velocity relative to the ice does the platform recoil?
View SolutionA person normally weighing 60kg stands on a platform which oscillates up and down harmonically at a frequency 2.0sec−1 and an amplitude 5.0cm . If a machine on the platform gives the person's weight against time deduce the maximum and minimum reading it will shown, Takeg=10m/sec2.
View SolutionA lift carries a person with a weighting machine in it, up and then down with the same constant acceleration. The weights recorded by the machine in the two cases are in the ratio 5:3. The acceleation of the lift is
View SolutionA person normally weighing 50 kg stands on a massless platform which oscillates up and down harmonically at a frequency of 2.0s−1 and an amplitude 5.0 cm. A weighing machine on the platform gives the persons wieght against time.
(a) Will there be any change in weight of the body, during the oscillation?
(b) If answer to part (a) is yes, what will be the maximum and minimum reading in the machine and at which position?
View SolutionA man is standing on a weighing machine placed in a lift. When stationary his weight is recorded as 40 kg . If the lift is accelerated upwards with an acceleration of 2m/s2 , then the weight recorded in the machine will be (g=10m/s2)
View SolutionA man of mass m is standing on a lift which moves down with an upward acceleration a. Find the pseudo force acting on the man as observed by himself. Find the pseudo force acting on the man if the lift falls freely.
View SolutionPassage XIII) Mr. Anoop having mass 50 kg is standing on a massless platform which oscillates up and down (doing SHM) of frequency 0.5 sec−1 and amplitude 0.4m. Platforms has a weighing machine fitted in it (Which is also massless). On which Anoop is standing. (Take (π2) = 10 and g = 10 m/s2. Assume that at t=0 (Platform+Anoop) is at their highest point of oscillation. [More than one option is correct]
Which of the following will be correct for reading of weighting machine
View SolutionA train in standing on a platform, a man inside a compartment of a train drops a stone. At the same instant train starts to move with constant acceleration. The path of the particle as seen by the person who drops the stone is:
View SolutionA man standing on a platform hears the sound of frequency 604 Hz coming from a frequency 550 Hz from a train whistle moving towards the platform. If the velocity of sound is 330 m/s, then what is the speed of train?
View Solution