A point object lies inside a transpatent solid sphere of radius 20cm and of refractive index n=2. When the object is viewed form air through the nearest surface it is seen at a distance 5cm form the surface. Find the apparent distance of object when its seen through the farthest curved surface
The correct Answer is:40cm
To solve the problem step by step, we will use the principles of refraction through spherical surfaces.
Step 1: Understand the Setup
We have a point object inside a transparent solid sphere with a radius of 20 cm and a refractive index n=2. The object is viewed from air through the nearest surface, where it appears at a distance of 5 cm from the surface.
Step 2: Identify the Variables
- Radius of the sphere, R=20cm
- Refractive index of the sphere, n2=2
- Refractive index of air, n1=1
- Image distance from the nearest surface, v=5cm
- Object distance from the nearest surface, u (to be determined)
Step 3: Use the Refraction Formula
The formula for refraction through a spherical surface is given by:
n1u+n2−n1R=n2v
Here, we need to find u when the object is viewed through the nearest surface.
Step 4: Substitute Known Values
Substituting the known values into the formula:
- n1=1
- n2=2
- v=5cm
- R=20cm
The equation becomes:
1u+2−120=25
Step 5: Simplify the Equation
This simplifies to:
1u+120=25
Step 6: Solve for 1u
Rearranging gives:
1u=25−120
To subtract these fractions, find a common denominator (which is 20):
25=820
So,
1u=820−120=720
Step 7: Calculate u
Taking the reciprocal gives:
u=207≈2.86cm
Step 8: Determine the Object Distance from the Farthest Surface
The total diameter of the sphere is 40cm. The distance from the nearest surface to the farthest surface is:
40cm−u=40cm−2.86cm≈37.14cm
Step 9: Use the Refraction Formula Again for the Farthest Surface
Now we will use the same refraction formula for the farthest surface, where the object distance u′=−37.14cm:
1u′+n2−n1R=n1v′
Substituting the values:
1−37.14+2−120=1v′
This simplifies to:
1−37.14+120=1v′
Step 10: Solve for v′
Finding a common denominator and solving gives:
1v′=−137.14+120
Calculating this will give the final apparent distance v′.
Final Calculation
Calculating v′ will yield:
v′≈80cm
Conclusion
The apparent distance of the object when viewed through the farthest surface is approximately 80cm.
---
We have a point object inside a transparent solid sphere with a radius of 20 cm and a refractive index n=2. The object is viewed from air through the nearest surface, where it appears at a distance of 5 cm from the surface.
- Radius of the sphere, R=20cm
- Refractive index of the sphere, n2=2
- Refractive index of air, n1=1
- Image distance from the nearest surface, v=5cm
- Object distance from the nearest surface, u (to be determined)
The formula for refraction through a spherical surface is given by:
Substituting the known values into the formula:
- n2=2
- v=5cm
- R=20cm
This simplifies to:
Rearranging gives:
Taking the reciprocal gives:
The total diameter of the sphere is 40cm. The distance from the nearest surface to the farthest surface is:
Now we will use the same refraction formula for the farthest surface, where the object distance u′=−37.14cm:
Finding a common denominator and solving gives:
Calculating v′ will yield:
The apparent distance of the object when viewed through the farthest surface is approximately 80cm.
Topper's Solved these Questions
- GEOMATRICAL OPTICS BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Exercise-2
62 Videos
- GEOMATRICAL OPTICS BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Exercise-3
80 Videos
- GEOMATRICAL OPTICS BOOK - RESONANCE ENGLISHCHAPTER - GEOMATRICAL OPTICS EXERCISE - Problem
33 Videos
- EXPERIMENTAL PHYSICSBOOK - RESONANCE ENGLISHCHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II
10 Videos
- GRAVITATIONBOOK - RESONANCE ENGLISHCHAPTER - GRAVITATIONEXERCISE - HIGH LEVEL PROBLEMS
16 Videos
Similar Questions
A point object is placed at the center of a glass sphere of radius 6cm and refractive index 1.5. The distance of virtual image from the surface is
View SolutionA point object is situated at a distance of 36 cm from the centre of the sphere of radius 12 cm and refractive index 1.5. Locate the position of the image due to refraction through sphere.
View SolutionA small air bubble is situated at a distance of 3 cm from the center of a glass sphere of radius 9 cm. When viewed from the nearest side, the bubble appears to be at a distance of 5 cm from the surface. Its apparent distance when viewed from the farthest side is n×5 cm where n is?
View SolutionThe figure ,shows a transparent sphere of radiusRand refractive indexμ.An object Ois placed at a distance x from the pole of the first surface so that a real image is formed at the pole of the exactly opposite surface.
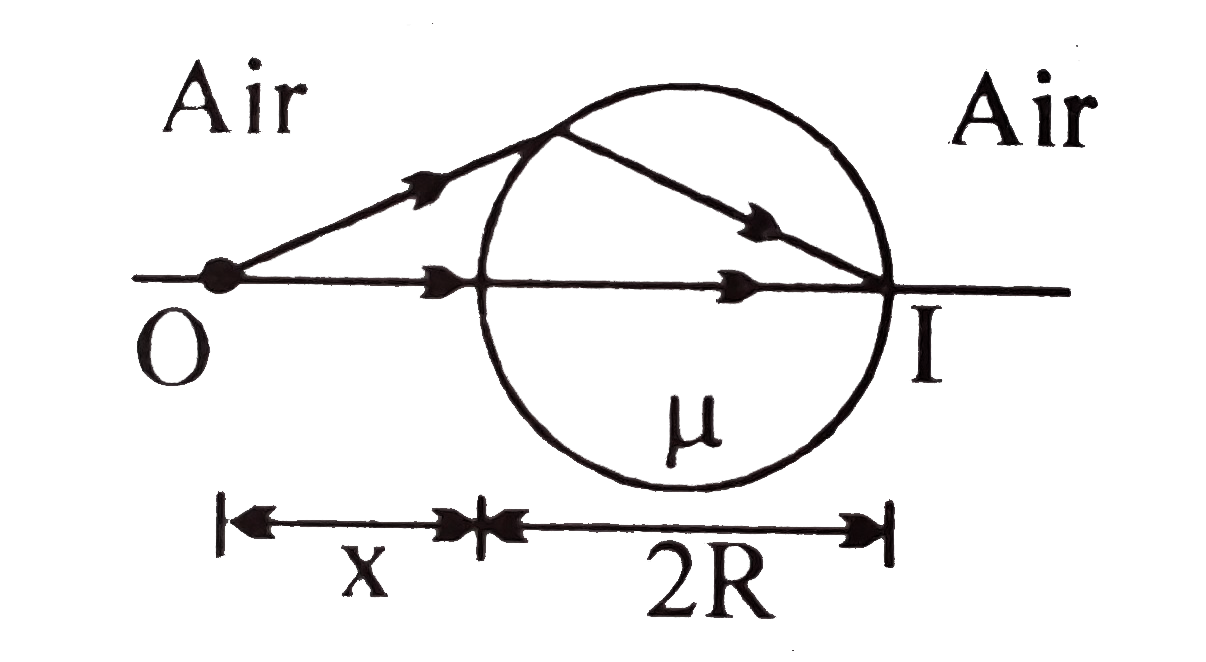 if an object is Placed at a distanceR from the pole of first surface ,then the real image is formed at a distance Rfrom thepole of the second surface.The refractive indexμ of the spher is given by
if an object is Placed at a distanceR from the pole of first surface ,then the real image is formed at a distance Rfrom thepole of the second surface.The refractive indexμ of the spher is given by
View SolutionA convex surface of radius of curvature 40 cm separate two media of refractive indices 43 and 1.50. An object is kept in the first medium at a distance of 20 cm from the surface. Calculate the position of the image.
View SolutionA concave spherical surface of radius of curvature 100 cm separates two media of refractive indices 1.50 and 43. An object is kept in the first medium at a distance of 30 cm from the surface. Calculate the position of the image.
View SolutionAn object lies 90cm in air avove water surface. It is viewed form water nearly normally. Find the apparent height fo the object.
View SolutionAn image is formed at a distance of 100 cm from the glass surface with refractive index 1.5, when a point object is placed in the air at a distance of 100 cm from the glass surface. The radius of curvature is of the surface is
View SolutionIn a spherical paper weight (R = 10 cm) made of glass of refractive index μ=32, an object is embedded at a distance 5 cm from its centre. What is the apparent position of the object when seen from the opposite side (see figure)?
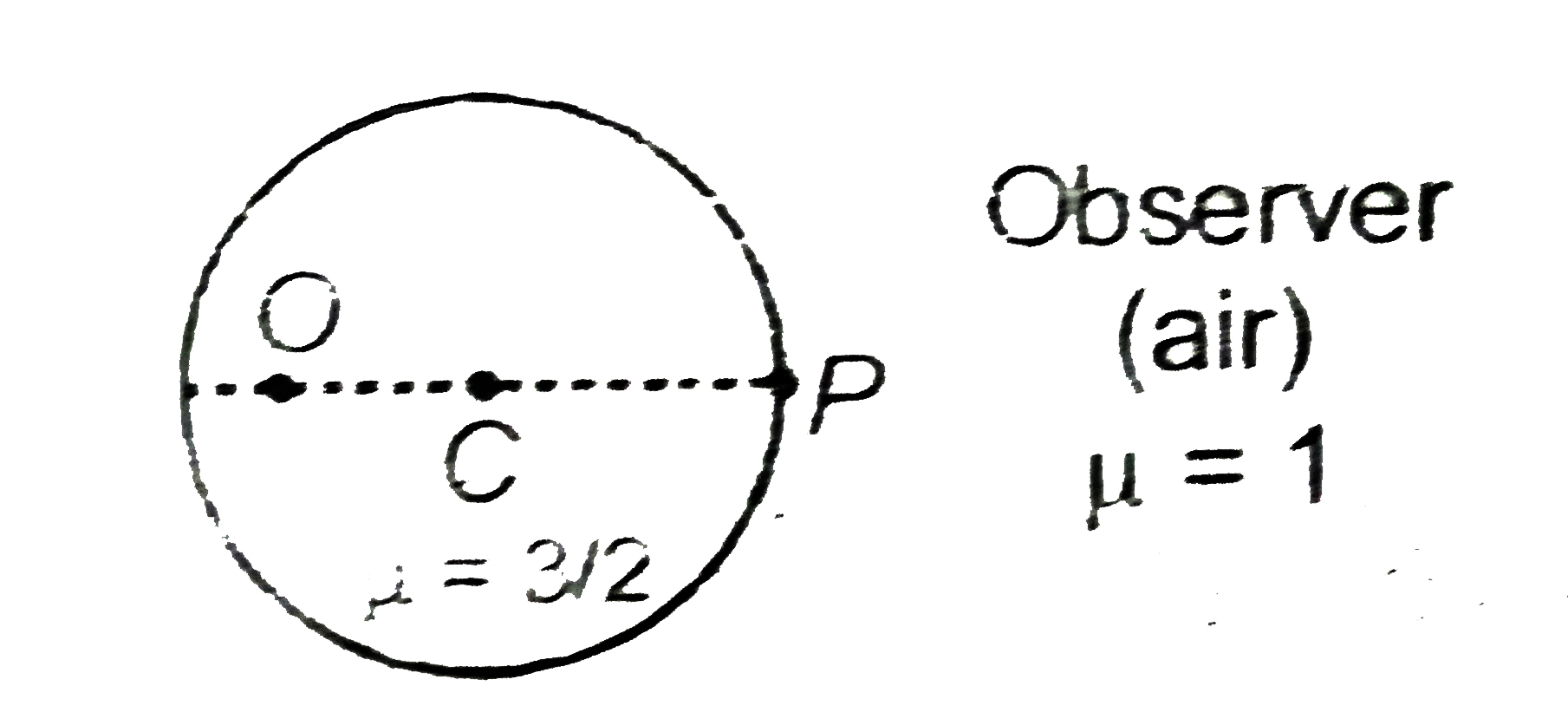
View SolutionAn object is placed at 24 cm distant from a surface of a lake. If water has refractive index of 4/3, then at what distance from the lake surface, a fish will get sight of an object.
View Solution
