An object is placed at 24 cm distant from a surface of a lake. If water has refractive index of 4/3, then at what distance from the lake surface, a fish will get sight of an object.
32 cm above the surface of water
18 cm over the surface of water
6 cm over the surface of water
6 cm below the surface of water
The correct Answer is:A
To solve the problem, we will use the formula that relates the apparent depth (d') and the real depth (d) of an object when viewed from a different medium, which is given by the refractive index (μ) of the medium:
μ=Apparent Depth (d')Real Depth (d)
1. Identify the Given Values:
- Real depth (d) = 24 cm
- Refractive index (μ) = 43
2. Write the Formula:
- The formula relating apparent depth and real depth is:
μ=d′d
Rearranging this gives:
d′=μ×d
3. Substitute the Known Values:
- Substitute the values of μ and d into the formula:
d′=43×24 cm
4. Calculate the Apparent Depth:
- Perform the multiplication:
d′=4×243=963=32 cm
5. Conclusion:
- The fish will see the object at an apparent depth of 32 cm from the surface of the lake.
Final Answer:
The distance from the lake surface at which the fish will see the object is 32 cm.
---
- Real depth (d) = 24 cm
- Refractive index (μ) = 43
- The formula relating apparent depth and real depth is:
μ=d′d
Rearranging this gives:
d′=μ×d
- Substitute the values of μ and d into the formula:
d′=43×24 cm
- Perform the multiplication:
d′=4×243=963=32 cm
- The fish will see the object at an apparent depth of 32 cm from the surface of the lake.
The distance from the lake surface at which the fish will see the object is 32 cm.
Topper's Solved these Questions
- RAY OPTICS AND WAVE OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS AND WAVE OPTICS EXERCISE - ENABLE
50 Videos
- RAY OPTICS AND WAVE OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS AND WAVE OPTICS EXERCISE - EFFICIENT
49 Videos
- RAY OPTICS BOOK - VMC MODULES ENGLISHCHAPTER - RAY OPTICS EXERCISE - IN-CHAPTER EXERCISE-J
10 Videos
- REVISION TEST-15 JEE - 2020BOOK - VMC MODULES ENGLISHCHAPTER - REVISION TEST-15 JEE - 2020EXERCISE - PHYSICS
25 Videos
Similar Questions
A point object is placed at the center of a glass sphere of radius 6cm and refractive index 1.5. The distance of virtual image from the surface is
View SolutionAn object is placed at a distance 24 cm in front of a convex lens of focal length 8 cm.
Calculate the distance of the image from the lens.
View Solution
Knowledge Check
A point source of light is placed at a depth of h below the surface of water of refractive index μ. A floating opaque disc is placed on the surface of water so that light from the source is not visible from the surface. The minimum diameter of the disc is
A2h(μ2−1)1/2B2h(μ2−1)1/2Ch2(μ2−1)1/2Dh(μ2−1)1/2
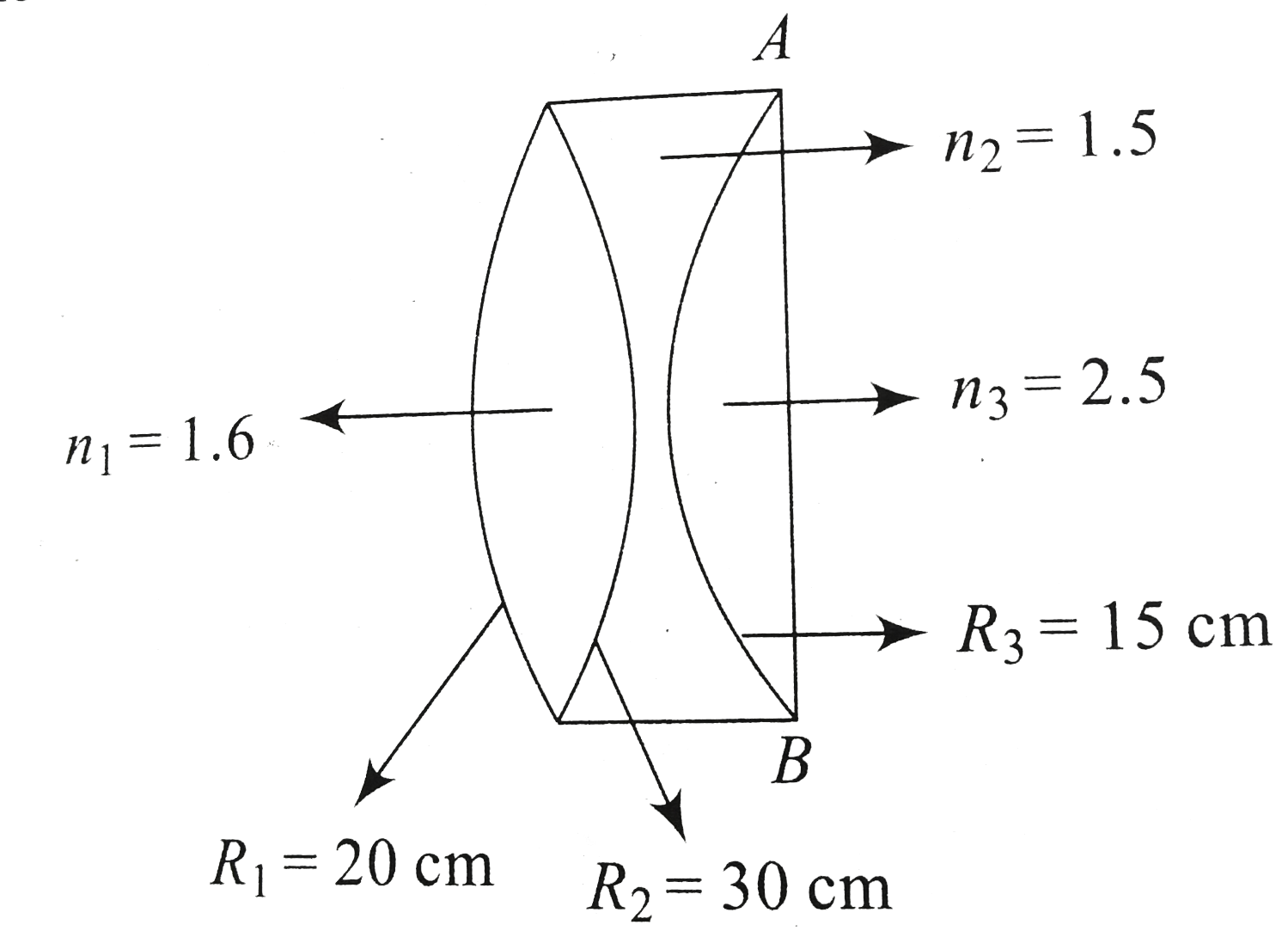
A lens is made of three thin different mediums. Radius of curvature and refractive index of each medium is shown iin Figure., Surface AB is straight. An object is placed at some distance from the lens by which a real image is formed on the screen placed at a distance of 10cm from the lens. Find the distance of the object from the lens.
View SolutionA point source of light is placed at a distance h below the surface of a large deep lake.
(a) Show that the fraction f of the light energy that escapes directly from the water surface is independent of h and is given by f=12−12n√n2−1 where n is the index of refraction of water.
(Note: Absorption within the water and reflection at the surface, except where it is total, have been neglected)
(b) Evaluate this ratio for n=4/3.
View SolutionThe figure ,shows a transparent sphere of radiusRand refractive indexμ.An object Ois placed at a distance x from the pole of the first surface so that a real image is formed at the pole of the exactly opposite surface.
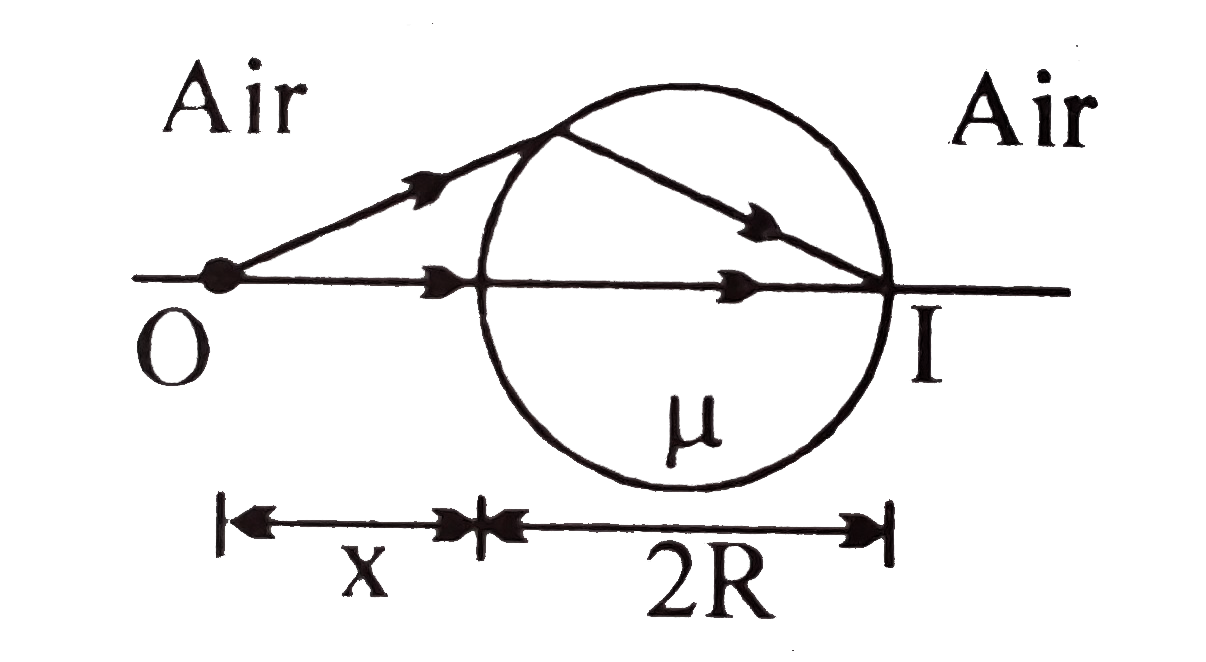 if an object is Placed at a distanceR from the pole of first surface ,then the real image is formed at a distance Rfrom thepole of the second surface.The refractive indexμ of the spher is given by
if an object is Placed at a distanceR from the pole of first surface ,then the real image is formed at a distance Rfrom thepole of the second surface.The refractive indexμ of the spher is given by
View SolutionAn object O is kept in air in front of a thin plano-convex lens of radius of curvature 10cm. Its refractive index at 3/2 and the medium towards right of the plane surface is water of refractive index 4/3. What should be distance x of the object so that the rays becomes parallel finally?

View SolutionAn object in placed 30cm in front of a concave lens that is made of a glass of refractive index 1.5 and has equal radii of curvature of its two surfaces, each 30 cm. The surface of the lens farther away from the object is silvered. Find the nature and position of the final image.
View SolutionA point source of light is placed at a distance hbelow the surface of a large deep lake.What is the percentage of light energy that escapes directly form the water surface if μof the water=4/3?(neglet partial reflection)
View SolutionA point source is placed at a depth h below the surface of water (refractive index =μ). The medium above the surface area of water is air from water.
View Solution
