A metallic metre stick translates in a direction making an angle of 60∘ with its length.The plane of motion is perpendicular to a uniform magnetic field of 0.1T that exists in the space.Find the emf induced between the ends of the rod if the speed of translation is 0.2m/s.
The correct Answer is:A, B, C
To solve the problem, we need to find the electromotive force (emf) induced between the ends of a metallic meter stick that is translating in a magnetic field. We will use the formula for induced emf in a moving conductor in a magnetic field.
1. Identify the given values:
- Magnetic field strength, B=0.1T
- Speed of translation, v=0.2m/s
- Length of the rod, L=1m (since it is a meter stick)
- Angle with the direction of motion, θ=60∘
2. Recall the formula for induced emf:
The induced emf (E) in a rod moving in a magnetic field is given by:
E=B⋅v⋅L⋅sin(θ)
3. Substitute the known values into the formula:
E=0.1T⋅0.2m/s⋅1m⋅sin(60∘)
4. Calculate sin(60∘):
sin(60∘)=√32
5. Substitute sin(60∘) into the equation:
E=0.1⋅0.2⋅1⋅√32
6. Perform the multiplication:
E=0.1⋅0.2⋅√32=0.02⋅√32
7. Calculate the final value:
E=0.02√32=0.01√3V
8. Approximate √3:
√3≈1.732
E≈0.01⋅1.732=0.01732V
Thus, the induced emf between the ends of the rod is approximately:
E≈0.01732V or 1.732×10−2V
Final Answer:
The induced emf is approximately 1.732×10−2V.
- Magnetic field strength, B=0.1T
- Speed of translation, v=0.2m/s
- Length of the rod, L=1m (since it is a meter stick)
- Angle with the direction of motion, θ=60∘
The induced emf (E) in a rod moving in a magnetic field is given by:
E=B⋅v⋅L⋅sin(θ)
E=0.1T⋅0.2m/s⋅1m⋅sin(60∘)
sin(60∘)=√32
E=0.1⋅0.2⋅1⋅√32
E=0.1⋅0.2⋅√32=0.02⋅√32
E=0.02√32=0.01√3V
√3≈1.732
E≈0.01⋅1.732=0.01732V
E≈0.01732V or 1.732×10−2V
The induced emf is approximately 1.732×10−2V.
Topper's Solved these Questions
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-1 PART 251videos
ELECTROMAGNETIC INDUCTION
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercis-2 PART 114videos
ELECTROMAGNETIC INDUCTION
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Problem12videos
ELECTROMAGNETIC INDUCTION
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTRODYNAMICSEXERCISE - Advanced level problems31videos
ELECTRODYNAMICS
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - HLP40videos
ELECTROSTATICS
Similar Questions
A wire of length 10cm translates in a direction making an angle of 60∘ with its length. The plane of motion is perpendicular ot a uniform magnetic field of 1.0 T that exists in the space. Find the emf induced between the ends of the rod if the speed of translation of 20 cm s^(-1).
View SolutionA wire of length 10cm translates in a direction making an angle of 60∘ with its length. The plane of motion is perpendicular ot a uniform magnetic field of 1.0 T that exists in the space. Find the emf induced between the ends of the rod if the speed of translation of 20cms−1.
View SolutionA conducting rod of unit length moves with a velocity of 5m/s in a direction perpendicular to its length and perpendicular to a uniform magnetic field of magnitude 0.4T. Find the emf induced between the ends of the stick.
View SolutionA metallic metre sitck moves with a velocity of 2 ms ^(-1) in a direction perpendicular to its length and perpendicular to a uniform magnetic field of magnitude 0.2 T. Find the emf indcued between the ends of the stick.
View SolutionA metallic wire PQ of length 1cm moves with a velocity of 2m/s in a direction perpendicular to its length and perpendicular to a uniform magnetic field of magnitude 0.2T.Find the emf induced between the ends of the wire.Which end will be positively charged.
 View Solution
View SolutionA metal rod of length 2 m is rotating with an angualr velocity of 100rads−1 in plane perpendicular to a uniform magnetic field of 0.3 T. The potential difference between the ends of the rod is
View SolutionA conductor of length 0.1m is moving with a velocity of 4m/s in a uniform magnetic field of 2T as shown in the figure. Find the emf induced ?
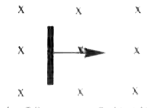 View Solution
View SolutionA conductor of length 0.1m is moving with a velocity of 4m/ s in a uniform magnetic field of 2T as shown in the figure. Find the emf induced ?
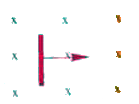 View Solution
View SolutionA conducting rod of length 2l is rotating with constant angular speed w about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The e.m.f. induced between two ends of the rod is
 View Solution
View SolutionA conducting rod of length 2l is rotating with constant angular speed w about its perpendicular bisector. A uniform magnetic field B exists parallel to the axis of rotation. The e.m.f. induced between two ends of the rod is
 View Solution
View Solution

