A glow-worm of mass 5.0g emits red light (650nm) with a power of 0.10w. Entirely in the backward direction. To what speed will it have accelerated sfter 10y if released into free space and assumed to live?
21ms−1
29ms−1
31.8ms−1
0.08ms−1
The correct Answer is:A
To solve the problem step by step, we will follow the calculations outlined in the video transcript while providing clear explanations for each step.
Step 1: Write down the given data
- Mass of the glow-worm, m=5.0g=5.0×10−3kg
- Wavelength of emitted light, λ=650nm=650×10−9m
- Power of emitted light, P=0.10W
- Time, t=10years=10×365×24×60×60s=10×3.156×107s
Step 2: Calculate the total time in seconds
t=10×3.156×107s=3.156×108s
Step 3: Relate power to energy and momentum
The power P can be related to the energy emitted per unit time. The energy of a single photon is given by:
E=hcλ
where h is Planck's constant (6.626×10−34J s) and c is the speed of light (3.0×108m/s).
Step 4: Calculate the number of photons emitted in time t
The total energy emitted in time t is:
Etotal=P⋅t
The number of photons n emitted is given by:
n=EtotalE=P⋅tE=P⋅t⋅λhc
Step 5: Substitute the values into the equation
Substituting the values we have:
n=(0.10W)⋅(3.156×108s)⋅(650×10−9m)(6.626×10−34J s)⋅(3.0×108m/s)
Step 6: Calculate the momentum
The momentum p of the emitted photons is given by:
p=n⋅hλ
Using the relation mv=p, we can express the speed v as:
mv=n⋅hλ
Thus,
v=n⋅hm⋅λ
Step 7: Substitute n back into the equation for v
Substituting n into the equation for v:
v=P⋅t⋅hm⋅c⋅λ
Step 8: Substitute all known values
Substituting the known values:
v=(0.10)⋅(3.156×108)⋅(6.626×10−34)(5.0×10−3)⋅(3.0×108)⋅(650×10−9)
Step 9: Calculate the speed v
Calculating the above expression gives:
v≈21m/s
Final Answer
The speed to which the glow-worm will have accelerated after 10 years is approximately 21m/s.
- Mass of the glow-worm, m=5.0g=5.0×10−3kg
- Wavelength of emitted light, λ=650nm=650×10−9m
- Power of emitted light, P=0.10W
- Time, t=10years=10×365×24×60×60s=10×3.156×107s
t=10×3.156×107s=3.156×108s
The power P can be related to the energy emitted per unit time. The energy of a single photon is given by:
E=hcλ
where h is Planck's constant (6.626×10−34J s) and c is the speed of light (3.0×108m/s).
The total energy emitted in time t is:
Etotal=P⋅t
The number of photons n emitted is given by:
n=EtotalE=P⋅tE=P⋅t⋅λhc
Substituting the values we have:
n=(0.10W)⋅(3.156×108s)⋅(650×10−9m)(6.626×10−34J s)⋅(3.0×108m/s)
The momentum p of the emitted photons is given by:
p=n⋅hλ
Using the relation mv=p, we can express the speed v as:
mv=n⋅hλ
Thus,
v=n⋅hm⋅λ
Substituting n into the equation for v:
v=P⋅t⋅hm⋅c⋅λ
Substituting the known values:
v=(0.10)⋅(3.156×108)⋅(6.626×10−34)(5.0×10−3)⋅(3.0×108)⋅(650×10−9)
Calculating the above expression gives:
v≈21m/s
The speed to which the glow-worm will have accelerated after 10 years is approximately 21m/s.
Topper's Solved these Questions
- BOOK - RESONANCE ENGLISHCHAPTER - NUCLEAR CHEMISTRYEXERCISE - PART-III5videos
NUCLEAR CHEMISTRY
- BOOK - RESONANCE ENGLISHCHAPTER - NUCLEAR CHEMISTRYEXERCISE - STAGE-II1videos
NUCLEAR CHEMISTRY
- BOOK - RESONANCE ENGLISHCHAPTER - NUCLEAR CHEMISTRYEXERCISE - ADVANCED LEVEL PROBLEMS30videos
NUCLEAR CHEMISTRY
- BOOK - RESONANCE ENGLISHCHAPTER - NITROGEN CONTAINING COMPOUNDSEXERCISE - ORGANIC CHEMISTRY(Nitrogen containing Compounds)30videos
NITROGEN CONTAINING COMPOUNDS
- BOOK - RESONANCE ENGLISHCHAPTER - P BLOCK ELEMENTSEXERCISE - PART -II23videos
P BLOCK ELEMENTS
Similar Questions
A torque of 10 Nm is applied to a flywheel of mass 5 kg and radius of gyration 0.6 m. What is the resultant angular acceleration ?
View SolutionA fireman of mass 60 kg slides down a pole. He is pressing the pole with a force of 600N. The coefficient of friction between the hands and the pole is 0.5, with what acceleration will the fireman slide down (g=10ms2)
View Solution
Knowledge Check
- Question 1 - Select One
A circular coil of 16 turns and radius 10cm carrying a current of 0.75A rests with its plane normal to an external field of magnitude 5⋅0×10−2T. The coil is free to turn about an axis in its plane perpendicular to the field direction. When the coil is turned slightly and released, it oscillates about its stable equilibrium with a frequency of 2⋅0s−1. What is the moment of inertia of the coil about its axis of rotation?
A1.13×10−1kg m2B1.13×10−2kg m2C1.113×10−3kg m2D1.13×10−4kg m2
A space craft flying in a straight course with a velocity of 75 km s−1 fires its rocket motors for 6.0 s. At the end of this time, its speed is 120 km s−1 in the same direction. Find : (i) the space craft's average acceleration while the motors were firing, (ii) the distance travelled by the space craft in the first 10 s after the rocket motors were started, the motors having been in action for only 6-0 s.
View SolutionA man of 50kg mass is standing in a gravity free space at a height of 10m above the floor. He throws a stone of 0.5kg mass downwards with a speed 2m/s. When the stone reaches the floor, the distance of the man above the floor will be
View SolutionA man of 50kg mass is standing in a gravity free space at a height of 10m above the floor. He throws a stone of 0.5kg mass downwards with a speed 2m/s. When the stone reaches the floor, the distance of the man above the floor will be
View SolutionA particle of mass 0.2 kg has an intial speed of 5ms−1 at the bottom of a rough inclined plane of inclination 30∘ and vertical height 0.5 m . What is the speed of the particle as it reaches the top of the inclined plan ? (Take μ=1/√3,g=10ms−2 ) .
View SolutionA circular coil of 16 turns and radius 10cm carrying a current of 0.75A rests with its plane normal to an external field of magnitude 5⋅0×10−2T. The coil is free to turn about an axis in its plane perpendicular to the field direction. When the coil is turned slightly and released, it oscillates about its stable equilibrium with a frequency of 2⋅0s−1. What is the moment of inertia of the coil about its axis of rotation?
View SolutionA block of mass m height 2h and width 2b rests on a flat car which moves horizontally with constant acceleration a as shown in figure. Determine
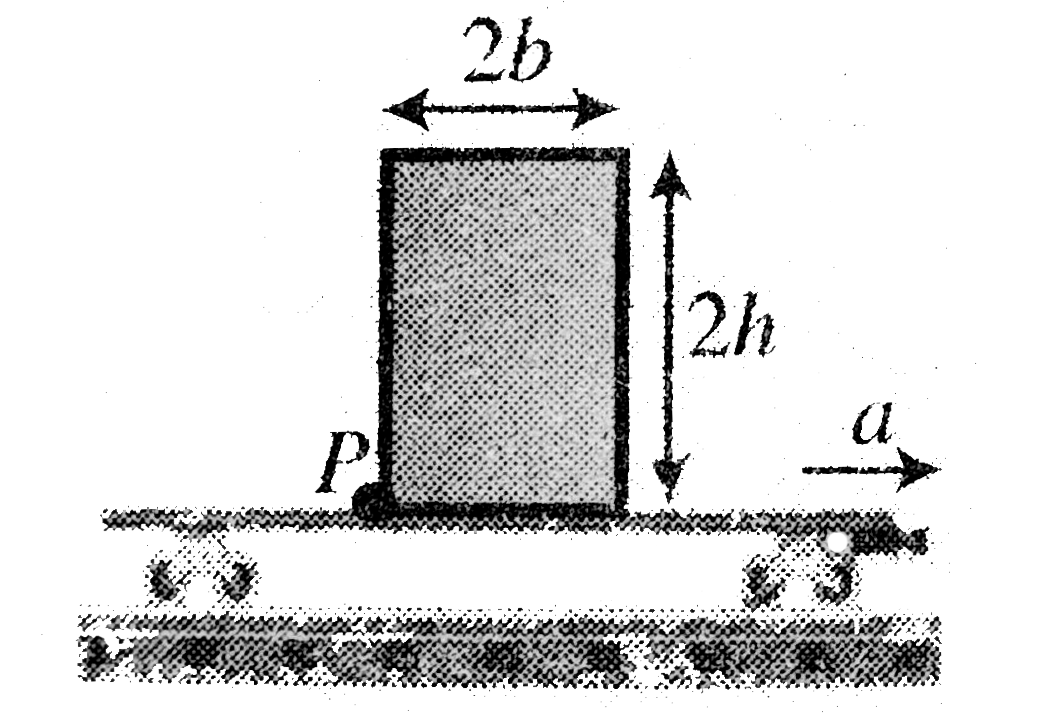
a. the value of the acceleration at which slipping of the block on the car starts, if the coefficient of friction is μ.
b. the value of the acceleration at which block topples about A, assuming sufficient friction to prevet slipping and
c. the shortest distance in which it can be stopped from a speed of 20ms−1 with constant deceleration so that the block is not disturbed. The following data are given b=0.6m,h=0.9m,μ=0.5 and g=10ms−2View SolutionAn aeroplane of mass 10,000 kg requires a speed of 20 ms−1 for take-off run of 100 m on the ground. The coefficient of kinetic friction between the wheels of the plane and ground is 0.3. Assume that the plane accelerates uniformly during the take off. Determine the minimum force required by the engine of the plane to take off (g=10ms−2).
View Solution

