Volume of HCP unit cell is:
24√2r3
8√2r3
16√2r3
24√3r3
The correct Answer is:A
To find the volume of a Hexagonal Close-Packed (HCP) unit cell, we can follow these steps:
Step 1: Understand the Structure of HCP
The HCP unit cell has a hexagonal base and a certain height. The volume of the unit cell can be calculated using the formula:
Volume=Area of base×Height
Step 2: Calculate the Area of the Hexagonal Base
The base of the HCP unit cell is a hexagon that can be divided into six equilateral triangles. The area A of one equilateral triangle with side length a is given by:
A=√34a2
Thus, the area of the hexagonal base, which consists of six such triangles, is:
Area of base=6×√34a2=3√32a2
Step 3: Determine the Height of the Unit Cell
For the HCP structure, the relationship between the height h of the unit cell and the side length a is given by:
ha=√83
From this, we can express the height h as:
h=√83a
Step 4: Substitute Area and Height into the Volume Formula
Now, substituting the area of the base and the height into the volume formula:
Volume=Area of base×Height=(3√32a2)×(√83a)
Step 5: Simplify the Expression
Now we simplify the expression:
Volume=3√32a2×√83a=√82a3√3
This can be further simplified as:
Volume=3⋅2√22a3=3√2a3
Step 6: Relate Side Length to Atomic Radius
In HCP, the side length a is related to the atomic radius r by the formula:
a=2r
Substituting this into the volume expression gives:
Volume=3√2(2r)3=3√2⋅8r3=24√2r3
Final Answer
Thus, the volume of the HCP unit cell is:
Volume=24√2r3
The HCP unit cell has a hexagonal base and a certain height. The volume of the unit cell can be calculated using the formula:
Volume=Area of base×Height
The base of the HCP unit cell is a hexagon that can be divided into six equilateral triangles. The area A of one equilateral triangle with side length a is given by:
A=√34a2
Thus, the area of the hexagonal base, which consists of six such triangles, is:
Area of base=6×√34a2=3√32a2
For the HCP structure, the relationship between the height h of the unit cell and the side length a is given by:
ha=√83
From this, we can express the height h as:
h=√83a
Now, substituting the area of the base and the height into the volume formula:
Volume=Area of base×Height=(3√32a2)×(√83a)
Now we simplify the expression:
Volume=3√32a2×√83a=√82a3√3
This can be further simplified as:
Volume=3⋅2√22a3=3√2a3
In HCP, the side length a is related to the atomic radius r by the formula:
a=2r
Substituting this into the volume expression gives:
Volume=3√2(2r)3=3√2⋅8r3=24√2r3
Thus, the volume of the HCP unit cell is:
Volume=24√2r3
Topper's Solved these Questions
- SOLID STATEBOOK - RESONANCE ENGLISHCHAPTER - SOLID STATEEXERCISE - Exercise -2 (Part-I)
19 Videos
- SOLID STATEBOOK - RESONANCE ENGLISHCHAPTER - SOLID STATEEXERCISE - Exercise -2 (Part-II Single correct double value integer type)
9 Videos
- SOLID STATEBOOK - RESONANCE ENGLISHCHAPTER - SOLID STATEEXERCISE - Exercise -1
20 Videos
- S BLOCK ELEMENTSBOOK - RESONANCE ENGLISHCHAPTER - S BLOCK ELEMENTSEXERCISE - ORGANIC CHEMISTRY(Biomolecules & Polymer)
4 Videos
- STRUCTURAL IDENTIFICATION & PRACTICAL ORGANIC CHEMISTRYBOOK - RESONANCE ENGLISHCHAPTER - STRUCTURAL IDENTIFICATION & PRACTICAL ORGANIC CHEMISTRYEXERCISE - Section -B
18 Videos
Similar Questions
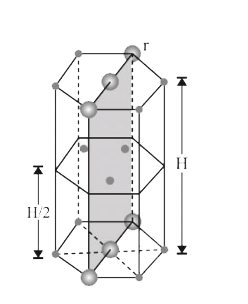
In hexagonal systems of crystals, a frequently encountered arrangement of atoms is described as a hexagonal prism. Here, the top and bottom of the cell are regular hexagons and three atoms are sandwiched in between them. A space-filling model of this structure, called hexagonal close-packed (HCP), is constituted of a sphere on a flat surface surrounded in the same plane by six identical spheres as closely as possible. Three spheres are then placed over the first layer so that they touch each other and represent the second layer. Each one of these three spheres touches three spheres of the bottom layer. Finally, the second layer is covered with a third layer that is identical to the bottom layer in relative position. Assume radius of every sphere to be ‘r’.
The volume of this HCP unit cell is
View SolutionThe height of a HCP unit cellis 5.715Å. What is the volume of the unit cell in Å3?
View SolutionA metal has bcc structure and the edge length of its unit cell is 3.04∘A. The volume of the unit cell in cm3 will be
View SolutionDensity of a unit cell is respresented as
ρ= Effective no. of atoms (s)×Mass of a unit cell Volume of a unit cell =Z.MNA.a3
where , Z = effective no . of atoms(s) or ion (s) per unit cell.
M= At . mass// formula
NA= Avogadro' s no . ⇒6.0323×1023
a= edge length of unit cell
Silver crystallizes in a fcc lattice and has a density of 10.6g/cm3. What is the length of a edge of the unit cell ?
View SolutionIce crystallises in hexagonal lattice having volume of unit cell is 132×10−24cm3.If density is 0.92g cm3at a given temperature, then number of water molecules per unit cell is
View SolutionIce crystallises in hexagonal lattice having volume of unit cell is 132×10−24cm3.If density is 0.92g cm3at a given temperature, then number of water molecules per unit cell is
View SolutionIce crystallises in hexagonal lattice having volume of unit cell is 132×10−24cm3.If density is 0.92g cm3at a given temperature, then number of water molecules per unit cell is
View SolutionIf calcium crystallizes in bcc arrangement and the radius of Ca atom is 96±, then the volume of unit cell of Ca is
View SolutionDensity of a unit cell is respresented as
ρ= Effective no. of atoms (s)×Mass of a unit cell Volume of a unit cell =Z.MNA.a3
where , Z =mass of effective no . of atoms(s) or ion (s).
M= At . mass// formula
NA= Avogadro' s no . ⇒6.0323×1023
a= edge length of unit cell
An element crystallizes in a structure having fcc unit cell of an edge 200 pm . Calculate the density , if 100 g of this element contains 12×1023 atoms :
View SolutionDensity of a unit cell is respresented as
ρ= Effective no. of atoms (s)×Mass of a unit cell Volume of a unit cell =Z.MNA.a3
where , Z = effective no . of atoms(s) or ion (s).
M= At . mass// formula
NA= Avogadro' s no . ⇒6.0323×1023
a= edge length of unit cell
The density of KBr is 2.75g/cm−3 . The length of the edge of the unit cell is 645 pm .To which type of cubic crystal , KBr belongs ?
View Solution