Let Z be the set of integers. If A = {x∈Z:2(x+2)(x2−5x+6)}=1 and B={x∈Z:−3<2x−1<9}, then the number of subsets of the set A × B is
A
218
B
212
C
215
D
210
Video Solution
Text Solution
The correct Answer is:D
NA
|
Topper's Solved these Questions
- RELATION, FUNCTION & ITFBOOK - RESONANCE ENGLISHCHAPTER - RELATION, FUNCTION & ITFEXERCISE - HLP_TYPE
38 Videos
- RELATION, FUNCTION & ITFBOOK - RESONANCE ENGLISHCHAPTER - RELATION, FUNCTION & ITFEXERCISE - SSP
55 Videos
- RELATION, FUNCTION & ITFBOOK - RESONANCE ENGLISHCHAPTER - RELATION, FUNCTION & ITFEXERCISE - JEE ADVANCED
12 Videos
- NUMBER THEORYBOOK - RESONANCE ENGLISHCHAPTER - NUMBER THEORYEXERCISE - Exercise -2 (PART - II)
4 Videos
- SEQUENCE & SERIES BOOK - RESONANCE ENGLISHCHAPTER - SEQUENCE & SERIES EXERCISE - EXERCISE -2 (PART-II : PREVIOUSLY ASKED QUESTION OF RMO)
3 Videos
Similar Questions
Knowledge Check
If −5<2x−3≤x+2,x∈R, then the solution set of x on the number line is
A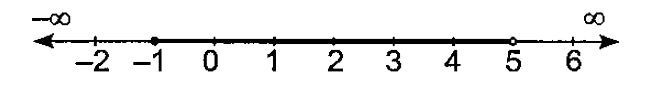 B
B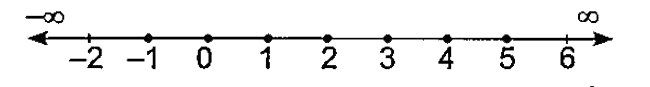 C
C D
D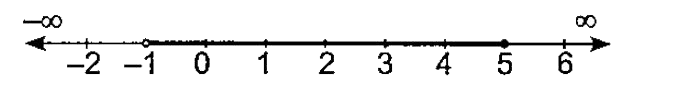
If 2x−3<x+1<4x+7,x∈R then the solution set of x on the number line is:
A B
B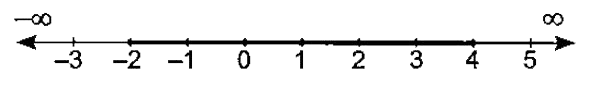 C
C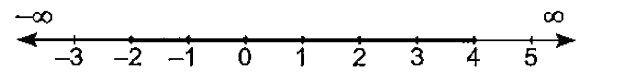 D
D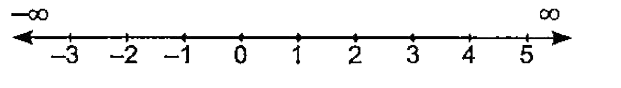
If 2x−5≤5x+4<29 and x is an integer, then the solution set of x is
A{−2,−1,0,1,2,3,4,5}B{−2,−1,0,1,2,3,4}C{−3,−2,−1,0,1,2,3,4,5}D{−3,−2,−1,0,1,2,3,4}
Let Z denoted the set of integers, then
{x∈Z|x−3∣<4}∩{x∈Z|x−4∣<5}=
View SolutionLet A={x:xεz and 2(x+2)(x2−5x+6)=1},B={x:xεz and −3<2x−1<9}. Find number of subsets of cartesian product A×B (A) 218 (B) 210 (C) 215 (D) 220
View SolutionIf A={x:x∈Nandx<614} and B={x:x∈Nandx2≤5) the number of subsets of set A×(A∩B) which contains exactly 3 elements is:
View SolutionWrite the following sets in the roster form :
(i){x:x2−5x+6=0,x∈N} (ii){x:2x−5<4,x∈Z}
View SolutionThe number of intergal values of x if 5x−1<(x+1)2<7x−3 is
View Solution