If then find the number of subsets of .
The correct Answer is:
To solve the problem, we need to find the set A defined by the condition −3<x<3 where x is an integer (i.e., x∈Z). Then, we will determine the number of subsets of this set A.
1. Identify the Set A:
- The condition −3<x<3 implies that x can take integer values between -3 and 3, but not including -3 and 3.
- Therefore, the integer values that satisfy this condition are:
−2,−1,0,1,2
- Thus, we can write:
A={−2,−1,0,1,2}
2. Count the Elements in Set A:
- The elements of set A are:
−2,−1,0,1,2
- There are a total of 5 elements in set A.
- Therefore, we have:
|A|=5
3. Calculate the Number of Subsets:
- The number of subsets of a set is given by the formula 2|A|, where |A| is the number of elements in the set.
- Since we found that |A|=5, we can calculate the number of subsets:
Number of subsets=2|A|=25=32
4. Conclusion:
- The number of subsets of the set A is:
32
- The condition −3<x<3 implies that x can take integer values between -3 and 3, but not including -3 and 3.
- Therefore, the integer values that satisfy this condition are:
−2,−1,0,1,2
- Thus, we can write:
A={−2,−1,0,1,2}
- The elements of set A are:
−2,−1,0,1,2
- There are a total of 5 elements in set A.
- Therefore, we have:
|A|=5
- The number of subsets of a set is given by the formula 2|A|, where |A| is the number of elements in the set.
- Since we found that |A|=5, we can calculate the number of subsets:
Number of subsets=2|A|=25=32
- The number of subsets of the set A is:
32
Topper's Solved these Questions
Similar Questions
Knowledge Check
If 2x−3<x+1<4x+7,x∈R then the solution set of x on the number line is:
A B
B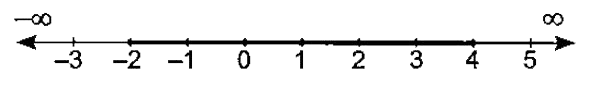 C
C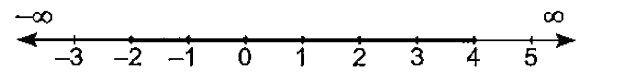 D
D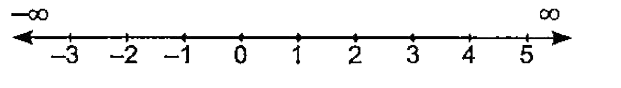
1x3,1x2,1x,x,x2,x3
If −1<x<0, what is the median of the six numbers in the list above ?A1xBx2Cx2(x+1)2Dx(x2+1)2
Let S={1,2,3,..............n} and A={(a,b)|1≤a,b≤n}=S×S. A subset B of A is such that (x,x)∈B for every x∈S. Then find the number of subsets B.
View Solution3x−2<2x+1
View SolutionSolve −3+x<2,x∈N.
View SolutionIn f(x)=[(cosx2,,x<0),(sinx3−∣∣x3−1∣∣,,x≥0) then find the number of points where g(x)=f(|x|) is non-differentiable.
View SolutionIn f(x)=[(cosx2,,x<0),(sinx3−∣∣x3−1∣∣,,x≥0) then find the number of points where g(x)=f(|x|) is non-differentiable.
View SolutionIf x<4 and x,y∈{1,2,3,..,10}, then find the number of ordered pairs (x,y).
View Solution