(i) Two charged particles having vharge 4.0×10−6C and mass 24×10−3Kg each are joining by an insulating string of length 1 m and the system is kept on a smooth horizontal table. Find the tension in the string.
(ii) If suddenly string is cut then what is the acceleration of each particle ?
(iii) Are they having equal acceleration ?
The correct Answer is:(i) Kq1q2r2=0.144N
(ii) Kq1q2mr2=6m/s2
(iii) No (Magnitude is same but direction is different)
Let's solve the question step by step.
Given Data:
- Charge of each particle, q=4.0×10−6C
- Mass of each particle, m=24.0×10−3kg
- Distance between the particles (length of the string), r=1.0m
- Coulomb's constant, k=9.0×109N m2/C2
(i) Finding the tension in the string:
The tension in the string is equal to the electrostatic force of repulsion between the two charged particles. The formula for the electrostatic force F is given by Coulomb's law:
F=kq1q2r2
Since both charges are the same, we can write:
F=kq2r2
Substituting the values:
F=9.0×109⋅(4.0×10−6)2(1.0)2
Calculating (4.0×10−6)2:
(4.0×10−6)2=16.0×10−12C2
Now substituting back into the equation for F:
F=9.0×109⋅16.0×10−121
Calculating F:
F=9.0×109⋅16.0×10−12=144.0×10−3N=0.144N
Thus, the tension in the string is:
Tension=0.144N
(ii) Finding the acceleration of each particle if the string is cut:
When the string is cut, each particle will experience the same force F and will have the same mass m. The acceleration a of each particle can be calculated using Newton's second law:
a=Fm
Substituting the values:
a=0.144N24.0×10−3kg
Calculating a:
a=0.1440.024=6.0m/s2
Thus, the acceleration of each particle is:
Acceleration=6.0m/s2
(iii) Are they having equal acceleration?
Since both particles have the same mass and experience the same force when the string is cut, they will have equal acceleration.
Thus, the answer is:
Yes, they have equal acceleration.
Summary of Answers:
(i) Tension in the string = 0.144N
(ii) Acceleration of each particle = 6.0m/s2
(iii) Yes, they have equal acceleration.
- Charge of each particle, q=4.0×10−6C
- Mass of each particle, m=24.0×10−3kg
- Distance between the particles (length of the string), r=1.0m
- Coulomb's constant, k=9.0×109N m2/C2
The tension in the string is equal to the electrostatic force of repulsion between the two charged particles. The formula for the electrostatic force F is given by Coulomb's law:
When the string is cut, each particle will experience the same force F and will have the same mass m. The acceleration a of each particle can be calculated using Newton's second law:
Since both particles have the same mass and experience the same force when the string is cut, they will have equal acceleration.
(i) Tension in the string = 0.144N
(ii) Acceleration of each particle = 6.0m/s2
(iii) Yes, they have equal acceleration.
Topper's Solved these Questions
- ELECTROSTATICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise-1 Section (B)
12 Videos
- ELECTROSTATICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Exercise-1 Section (C)
14 Videos
- ELECTROSTATICSBOOK - RESONANCE ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Problems
22 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - A.l.P
19 Videos
- EXPERIMENTAL PHYSICSBOOK - RESONANCE ENGLISHCHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II
10 Videos
Similar Questions
Two charged particles having charge 2.0×10−8C each are joined by an insulating string of length 1 m and the system is kept on a smooth horizontal table. Find the tension in the string.
View SolutionTwo charged particles having charge 2.0×10−8C. Each are joined by an isolating string of length 1m and the system is kept on a smooth horizontal task. The tension in the string.
View SolutionTwo charged particles, having equal charges of 2.0×10−5C each, are brought from infinity to within a separation of 10 cm. Find the increase in the electric potential energy during the process.
View SolutionA block of mass 1kg is tied to a string of length 1m the other end of which is fixed The block is moved on a smooth horizontal table with constant speed 10ms−1. Find the tension in the string
View SolutionA uniform rod of mass m and length L is kept on a horizontal table with L4 length on the table as shown in figure. As the string attached to the end B is cut, the angular acceleration of the rod about point C will be
_PHY_E05_242_Q01.png)
View SolutionTwo identical particles, each having a charge of 2.0×10−4C and mass of 10g, are kept at a separation of 10cm and then released. What would be the speeds of the particles when the separation becomes large?
View SolutionA particle of mass m is attached to a string of length L and given velocity √10gL in the horizontal direction at the lowest point. Find tension in the string when the particle is at (i) lowest position (ii) highest position,
View SolutionA body of mass 50 kg, suspended by two strings of lengths 30 cm and 40 cm fastend to two points in the same horizontal line 50 cm apart, is in equilibrium. Find the tension (in Newtons) in each string.
View SolutionTwo bodies of masses 1 kg and 2 kg are connected by a very light string passed over a clamped light smooth pulley. If the system is released from rest, find the acceleration of the two masses and the tension in the string
View SolutionA uniform string of length 10m and mass 20 kg lies on a smooth frictionless inclined plane. A force of 200N is applied as shown in the figure.
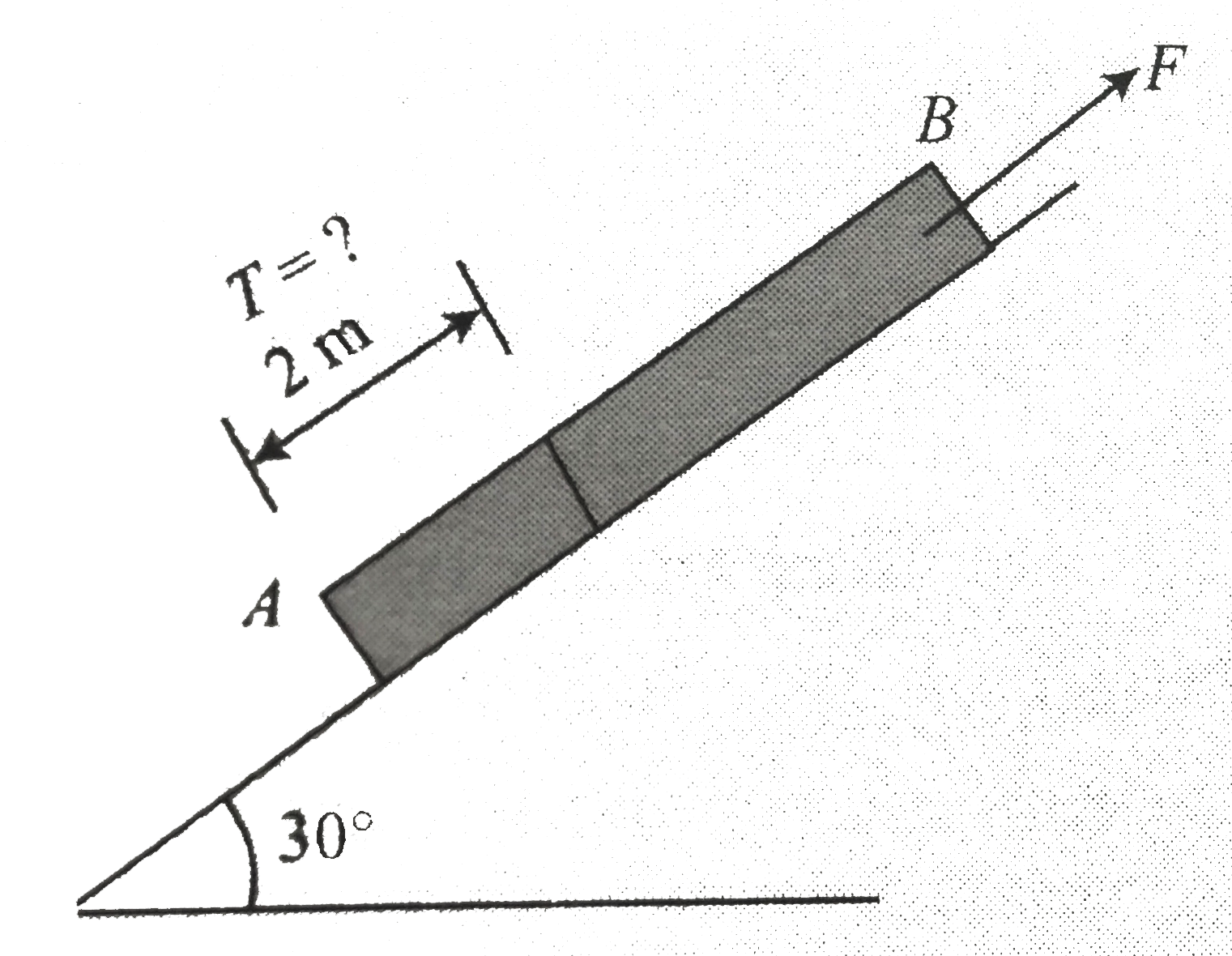
(a) Find the acceleration of the string.
(b) Find the tension in the string at 2m from end A.
View Solution
