Which of the following exert highest pressure at 300 K and 4 L volume (assume ideal behaviour) ?
28 g CO+ 40 g D2
88 g CO2 + 280 g N2
256 g O2 + 400 g SO3
24 g He + 128 g CH4
To determine which of the given options exerts the highest pressure at 300 K and 4 L volume, we can use the ideal gas law, which states:
P×V=n×R×T
Where:
- P = Pressure
- V = Volume
- n = Number of moles
- R = Ideal gas constant
- T = Temperature
Since R, V, and T are constants in this scenario, we can conclude that pressure P is directly proportional to the number of moles n:
P∝n
This means that the gas with the highest number of moles will exert the highest pressure. Now, let’s calculate the number of moles for each option.
Step 1: Calculate the number of moles for each option.
Option A: 28 g of Carbon Monoxide (CO) + 40 g of Deuterium (D)
1. Moles of CO:
Molecular mass of CO=12(C)+16(O)=28g/mol
nCO=28g28g/mol=1mol
2. Moles of D:
Molecular mass of D=2g/mol
nD=40g2g/mol=20mol
Total moles in Option A:
nA=1+20=21mol
Option B: 88 g of Carbon Dioxide (CO2) + 280 g of Nitrogen (N2)
1. Moles of CO2:
Molecular mass of CO2=12(C)+2×16(O)=44g/mol
nCO2=88g44g/mol=2mol
2. Moles of N2:
Molecular mass of N2=2×14(N)=28g/mol
nN2=280g28g/mol=10mol
Total moles in Option B:
nB=2+10=12mol
Option C: 256 g of Oxygen (O2) + 400 g of Sulfur Dioxide (SO2)
1. Moles of O2:
Molecular mass of O2=2×16(O)=32g/mol
nO2=256g32g/mol=8mol
2. Moles of SO2:
Molecular mass of SO2=32(S)+2×16(O)=64g/mol
nSO2=400g64g/mol=6.25mol
Total moles in Option C:
nC=8+6.25=14.25mol
Option D: 24 g of Helium (He) + 128 g of Methane (CH4)
1. Moles of He:
Molecular mass of He=4g/mol
nHe=24g4g/mol=6mol
2. Moles of CH4:
Molecular mass of CH4=12(C)+4(H)=16g/mol
nCH4=128g16g/mol=8mol
Total moles in Option D:
nD=6+8=14mol
Step 2: Compare the total moles from each option.
- Option A: 21mol
- Option B: 12mol
- Option C: 14.25mol
- Option D: 14mol
Conclusion
The option with the highest number of moles is Option A with 21 moles. Therefore, it will exert the highest pressure.
Final Answer:
Option A: 28 g Carbon Monoxide + 40 g Deuterium
- P = Pressure
- V = Volume
- n = Number of moles
- R = Ideal gas constant
- T = Temperature
Molecular mass of CO=12(C)+16(O)=28g/mol
nCO=28g28g/mol=1mol
Molecular mass of D=2g/mol
nD=40g2g/mol=20mol
nA=1+20=21mol
Molecular mass of CO2=12(C)+2×16(O)=44g/mol
nCO2=88g44g/mol=2mol
Molecular mass of N2=2×14(N)=28g/mol
nN2=280g28g/mol=10mol
nB=2+10=12mol
Molecular mass of O2=2×16(O)=32g/mol
nO2=256g32g/mol=8mol
Molecular mass of SO2=32(S)+2×16(O)=64g/mol
nSO2=400g64g/mol=6.25mol
nC=8+6.25=14.25mol
Molecular mass of He=4g/mol
nHe=24g4g/mol=6mol
Molecular mass of CH4=12(C)+4(H)=16g/mol
nCH4=128g16g/mol=8mol
nD=6+8=14mol
- Option B: 12mol
- Option C: 14.25mol
- Option D: 14mol
Option A: 28 g Carbon Monoxide + 40 g Deuterium
Topper's Solved these Questions
Similar Questions
Knowledge Check
Which of the following graphs represent the behaviour of an ideal gas ?
A1.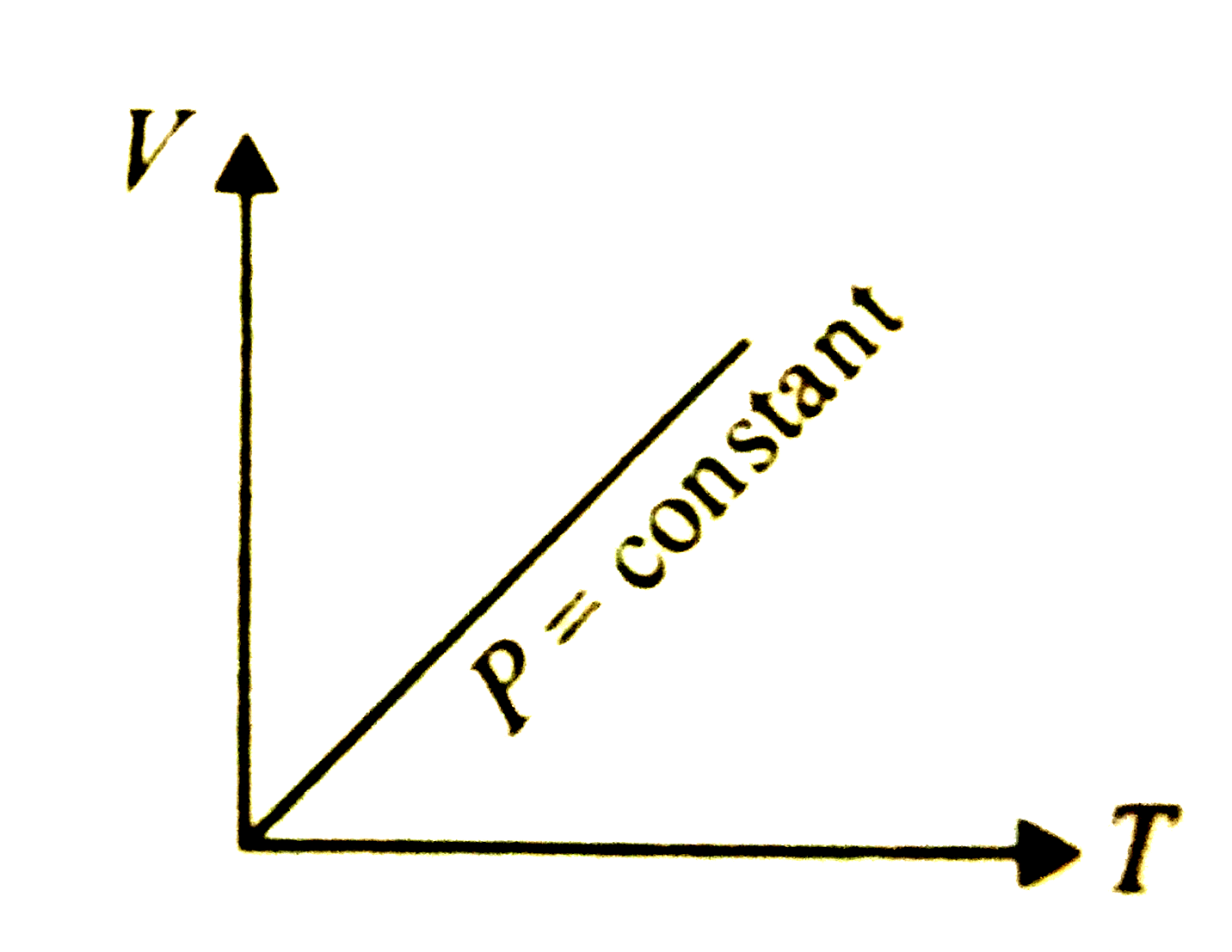 B2.
B2.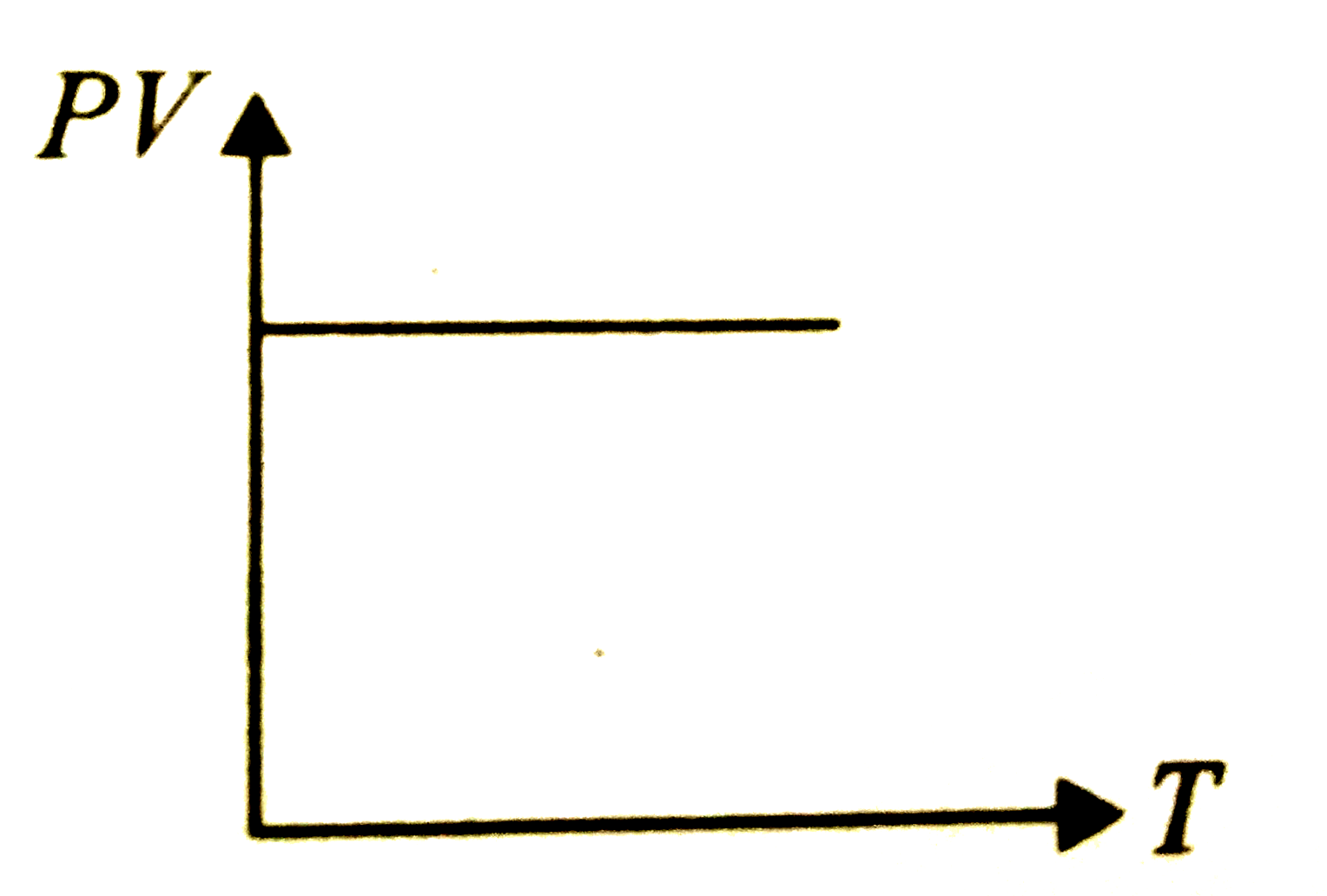 C3.
C3.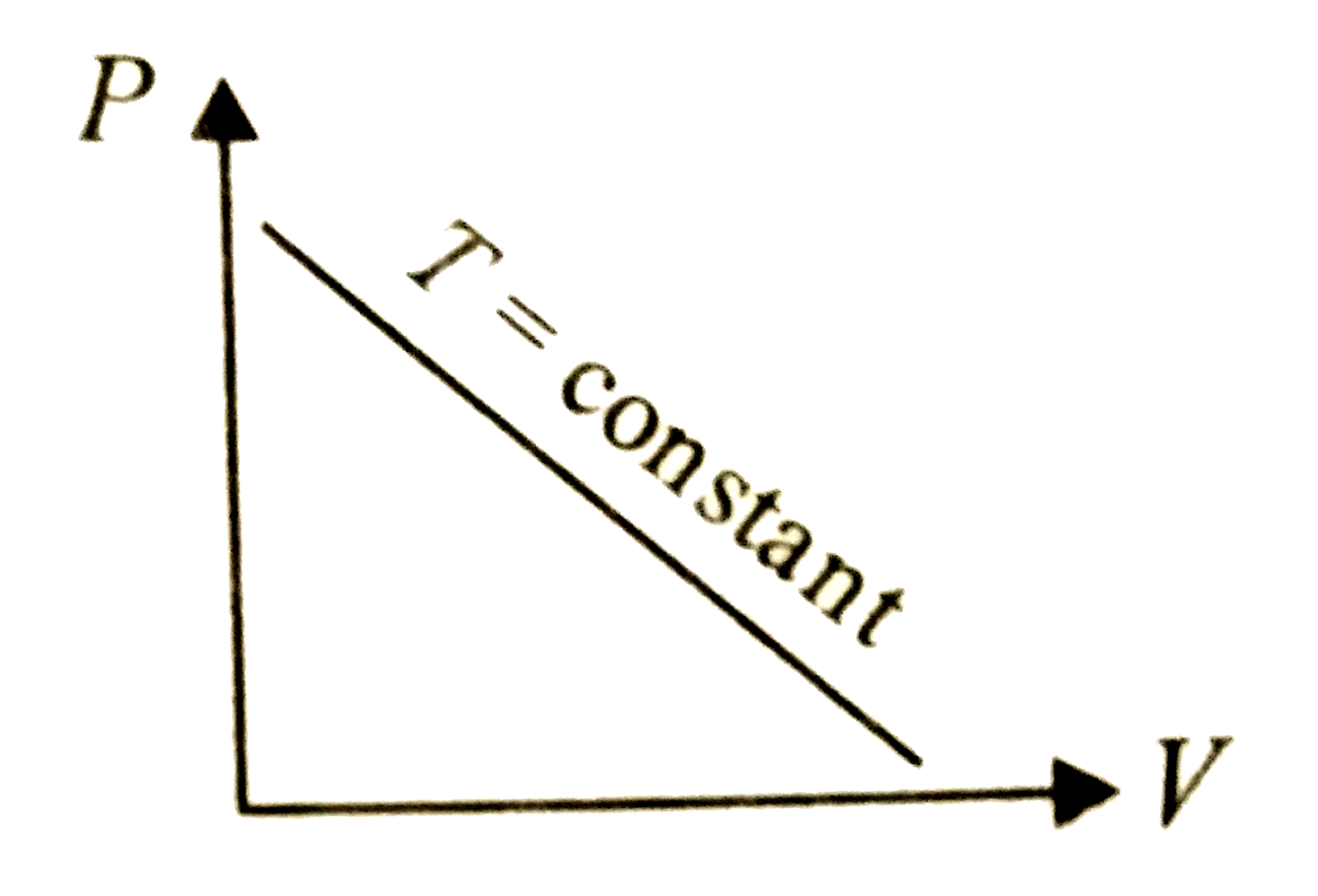 D4.
D4.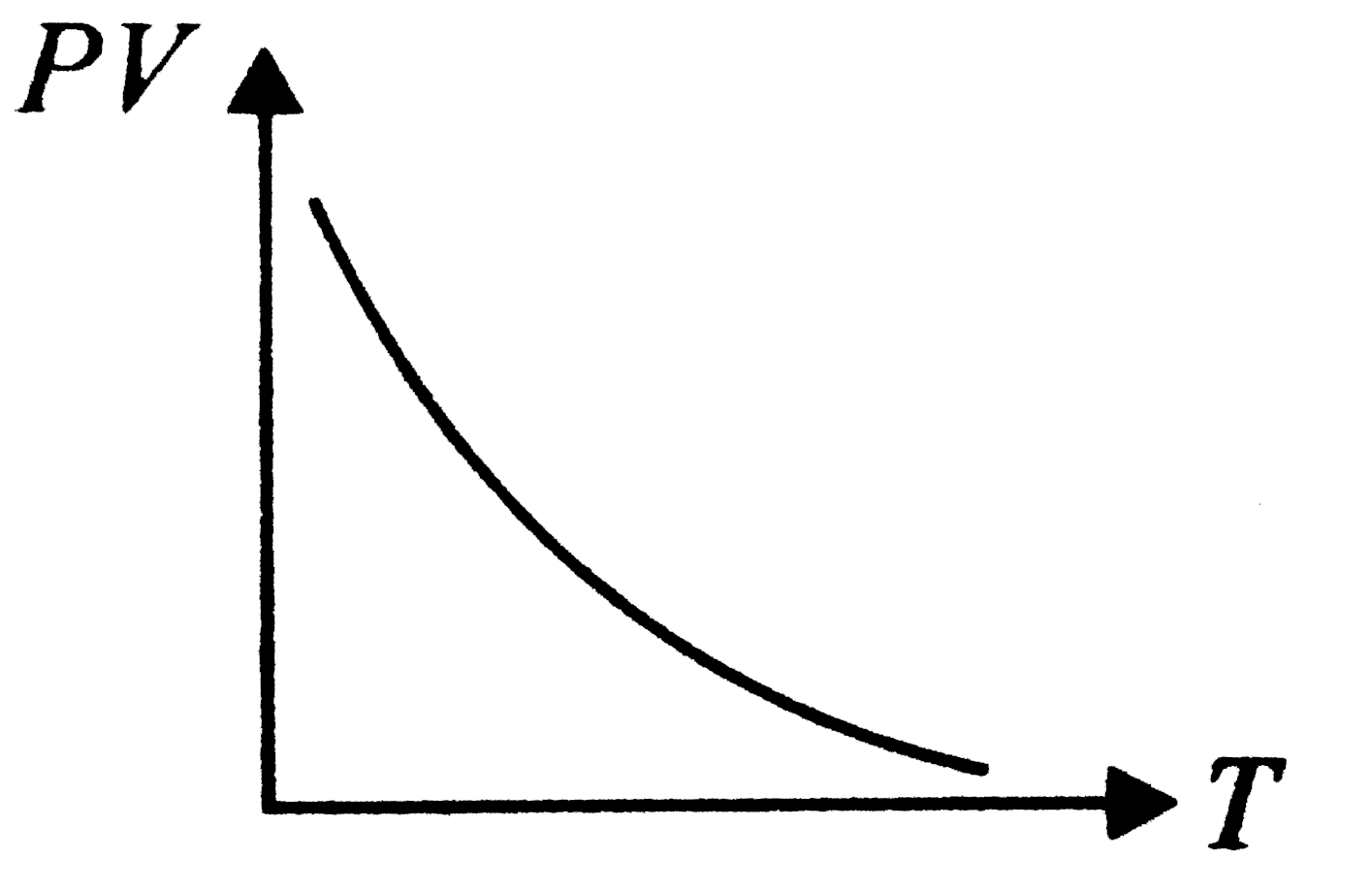
Under which of the following sets of conditions is a real gas expected to deviate from ideal behaviour?
(I) High pressure, small volume
(II) High temperature , low pressure
(III) Low temperature, high pressure
View SolutionWhich of the following graphs is consistent with ideal gas behaviour?
View SolutionWhich of the following diagrams (figure) depicts ideal gas behaviour?
View SolutionWhich of the following gases always show positive deviation from ideal behaviour with increase in pressure ?
View SolutionWhich one of the following solutions will have highest osmotic pressure? (Assume that all the salts are equally dissociated)?
View SolutionWhich of the following graphs is inconsistent with ideal gas behaviour ? (Assume n = constant)
View SolutionGases tend to behave non-ideally at low temperatures and high pressures. The deviation from ideal behaviour can be explained by considering two types of corrections. They are volume correction and pressure correction.
Select incorrect statement(s) :
View Solution
