ABCDEF is a regular hexagon with centre a the origin such that (A) 2 (B) 4 (C) 6 (D) 3
4
2
The correct Answer is:B
To solve the problem, we need to find the value of λ in the equation:
→AB+→ED+→FC=λ→ED
Given that ABCDEF is a regular hexagon centered at the origin, we can start by analyzing the vectors involved.
Step 1: Understand the properties of the regular hexagon
In a regular hexagon, all sides are equal, and the angles between adjacent sides are 120∘. The vectors representing the sides can be expressed in terms of a common length x.
Step 2: Define the vectors
Let:
- →AB=→ED=→FO=→OC=x
Step 3: Find →FC
The vector →FC can be expressed as the sum of →FO and →OC:
→FC=→FO+→OC=x+x=2x
Step 4: Substitute the vectors into the equation
Now we can substitute the values of the vectors into the original equation:
→AB+→ED+→FC=x+x+2x=4x
Step 5: Set up the equation with λ
We know that:
λ→ED=λx
Thus, we can equate the two sides:
4x=λx
Step 6: Solve for λ
Assuming x≠0, we can divide both sides by x:
4=λ
So, the value of λ is:
λ=4
Conclusion
The answer is λ=4, which corresponds to option (B).
---
In a regular hexagon, all sides are equal, and the angles between adjacent sides are 120∘. The vectors representing the sides can be expressed in terms of a common length x.
Let:
- →AB=→ED=→FO=→OC=x
The vector →FC can be expressed as the sum of →FO and →OC:
→FC=→FO+→OC=x+x=2x
Now we can substitute the values of the vectors into the original equation:
→AB+→ED+→FC=x+x+2x=4x
We know that:
λ→ED=λx
Thus, we can equate the two sides:
4x=λx
Assuming x≠0, we can divide both sides by x:
4=λ
So, the value of λ is:
λ=4
The answer is λ=4, which corresponds to option (B).
Topper's Solved these Questions
- VECTORS BOOK - VMC MODULES ENGLISHCHAPTER - VECTORS EXERCISE - LEVEL -2
47 Videos
- VECTORS BOOK - VMC MODULES ENGLISHCHAPTER - VECTORS EXERCISE - Numerical ValueType for JEE Main
15 Videos
- VECTORS BOOK - VMC MODULES ENGLISHCHAPTER - VECTORS EXERCISE - JEE ADVANCED (ARCHIVE) (TRUE/ FALSE)
30 Videos
- TRIGONOMETRIC IDENTITIES AND EQUATIONS BOOK - VMC MODULES ENGLISHCHAPTER - TRIGONOMETRIC IDENTITIES AND EQUATIONS EXERCISE - JEE Advanced (Archive)
11 Videos
Similar Questions
Knowledge Check
If ABCD is a rhombus whose diagonals intersect at E, then −−→EA+−−→EB+−−→EC+−−→ED equals
A→0B−−→ADC2−−→BCD2−−→AD
If ABCDEF is a regualr hexagon, then −−→AC+−−→AD+−−→EA+−−→FA=
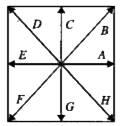
View SolutionA number of vectors are as shown in .They can be combined in various ways , like →A+→C=→B . What is the value of (a) →E+→C (b) →A+→F (c ) →A+→D (d) →E+→A (e ) →A−→B .
View SolutionIf ABCDEF is a regular hexagon, prove that −−→AC+−−→AD+−−→EA+−−→FA=3−−→AB
View SolutionIf ABCDEF is a regular hexagon, prove that AD+EB+FC=4AB.
View SolutionIn a regular hexagon ABCDEF, −−→AE
View SolutionConsider the regular hexagon ABCDEF with centre at O (origin).
Q. AD+EB+FC is equal to
View SolutionIf ABCDEF is a regular hexagon then −−→AD+−−→EB+−−→FC equals :
View Solution
