A particle moves along x-axis such that its position veries with time as x=50t−5t2. Select the correct alternative (s).
There must be a fourth force acting on the particle
There may be a fourth force acting on the particle
There must be only given three forces acting on the particle
There may be only three given forces acting on the particle
The correct Answer is:bd
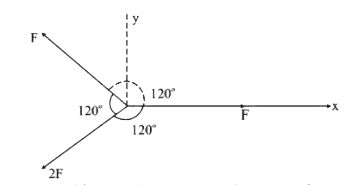
We find that resultant is along ‘2F’ (240∘ with +ve x-axis). If the particle is moving along 60∘ with +ve x-axis there are two possibilities. (i) There is a fourth force (ii) The particle was given an initial velocity in this direction
Topper's Solved these Questions
- INTRODUCTION TO VECTORS & FORCES BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - JEE Main ( ARCHIVE ) ( LEVEL-1)
12 Videos
- INTRODUCTION TO VECTORS & FORCES BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - JEE Advanced ( ARCHIVE LEVEL-2)
12 Videos
- INTRODUCTION TO VECTORS & FORCES BOOK - VMC MODULES ENGLISHCHAPTER - INTRODUCTION TO VECTORS & FORCES EXERCISE - level 1
75 Videos
- GRAVITATIONBOOK - VMC MODULES ENGLISHCHAPTER - GRAVITATIONEXERCISE - JEE Advance (Archive) TRUE/FALSE
1 Videos
- JEE MAIN - 5BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN - 5EXERCISE - PART I : PHYSICS (SECTION - 2)
5 Videos
Similar Questions
An object moves along x-axis such that its position varies with time according to the relation x=50t−5t2
Here, x is in meters and time is in seconds. Find the displacement and distance travelled for the time interval t = 0 to t = 6 s.
View SolutionA particle moves along the x-axis in such a way that its x-co-ordinate varies with time as x=2–5t+6t2.
The initial velocity and acceleration of particle will respectively be-
View SolutionA particle starts moving along the x-axis from t=0, its position varying with time as x=2t3−3t2+1.
a. At what time instants is its velocity zero?
b. What is the velocity when it passes through the origin?
View SolutionA particle moves along X-axis in such a way that its coordinate X varies with time t according to the equation x=(2−5t+6t2)m. The initial velocity of the particle is
View SolutionA particle moves along the X-axis as x=u(t−2s)+a(t−2s)2.
View SolutionA particle moves along x - axis in such a way that its x - co - ordinate varies with time according to the equation x=4−2t+t2. The speed of the particle will vary with time as
View SolutionA body moves along x-axis such that its displacement varies with time as given, x=7+3t+5t2. The average velocity during 3rd second is
View SolutionA particle moves along the X-axis as x=u(t−2s)=at(t−2)2.
View SolutionA particle moves along a straight line its velocity dipends on time as v=4t−t2. Then for first 5s:
View SolutionA particle is moving along the x-axis whose position is given by x=4−9t+t33. Mark the correct statement(s) in relation to its motion.
View Solution
