An ideal gas is initially at temperature T and volume V. ITS volume is increased by due to an increase in temperature , pressure remaining constant. The quantity varies with temperature as
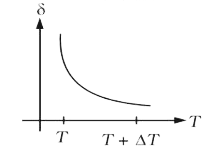
The correct Answer is:C
For an ideal gas:
For p = constant
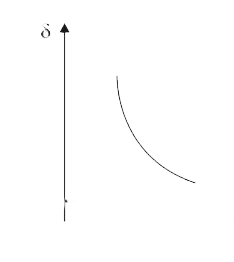
Therefore, is inversely proportional to temperature T. i.e.,
When T increases, decreases and vice-versa.
Hence, graph will be a rectangular hyperbola as shown in the above figure.
Topper's Solved these Questions
- GASEOUS STATE & THERMODYNAMICSBook:VMC MODULES ENGLISHChapter:GASEOUS STATE & THERMODYNAMICSExercise:JEE MAIN (ARCHIVE )
Explore 81 Videos
- ENERGY & MOMENTUMBook:VMC MODULES ENGLISHChapter:ENERGY & MOMENTUMExercise:JEE ADVANCE (ARCHIVE) - TRUE/FALSE TYPE
Explore 1 Video
- GRAVITATIONBook:VMC MODULES ENGLISHChapter:GRAVITATIONExercise:JEE Advance (Archive) TRUE/FALSE
Explore 1 Video
Similar Questions
Knowledge Check
If the pressure and the volume of certain quantity of ideal gas are halved, then its temperature
Ais doubledBbecomes one-fourthCremains constantDbecome four timesAs the temperature increases, average kinetic energy of molecules increases. What would be the effect of increase of temperature on pressure provided the volume is constant ?
AIncreasesBDecreasesCRemains sameDBecomes half
By increasing temperature of a gas by 6∘C its pressure increases by 0.4%, at constant volume. Then initial temperature of gas is
View SolutionThe pressure p and volume V of an ideal gas both increase in a process.
View SolutionAt constant temperature if the pressure of an ideal gas is increased by 10% then its volume must decrease by
View SolutionAt constant temperature if the pressure of an ideal gas is increased by 10% then its volume must decrease by
View SolutionAs the temperature increases, average kinetic energy of molecules increases. What would be the effect of increase of temperature on pressure provided the volume is constant ?
View SolutionWhen volume of system is increased two times and temperature is decreased half of its initial temperature, then pressure becomes ………….
View Solution
