Average velocity of a particle moving in a straight line, with constant acceleration a and initial velocity u in first t seconds is.
u+12at
u+at
u+at2
u2
The correct Answer is:A
To find the average velocity of a particle moving in a straight line with constant acceleration, we can follow these steps:
Step 1: Understand the parameters
We have:
- Initial velocity (u)
- Constant acceleration (a)
- Time duration (t)
Step 2: Use the formula for average velocity
The average velocity (V_avg) of an object moving with constant acceleration can be calculated using the formula:
Vavg=Vf+Vi2
where Vf is the final velocity and Vi is the initial velocity.
Step 3: Calculate the final velocity
The final velocity (V_f) after time t can be calculated using the equation of motion:
Vf=u+at
Step 4: Substitute the values into the average velocity formula
Now, substitute Vf and Vi into the average velocity formula:
Vavg=(u+at)+u2
Step 5: Simplify the expression
Combine the terms in the numerator:
Vavg=2u+at2
Step 6: Final expression for average velocity
Thus, the average velocity of the particle in the first t seconds is:
Vavg=u+at2
Conclusion
The average velocity of the particle moving in a straight line with constant acceleration a and initial velocity u in the first t seconds is:
Vavg=u+at2
---
We have:
- Initial velocity (u)
- Constant acceleration (a)
- Time duration (t)
The average velocity (V_avg) of an object moving with constant acceleration can be calculated using the formula:
Vavg=Vf+Vi2
where Vf is the final velocity and Vi is the initial velocity.
The final velocity (V_f) after time t can be calculated using the equation of motion:
Vf=u+at
Now, substitute Vf and Vi into the average velocity formula:
Vavg=(u+at)+u2
Combine the terms in the numerator:
Vavg=2u+at2
Thus, the average velocity of the particle in the first t seconds is:
Vavg=u+at2
The average velocity of the particle moving in a straight line with constant acceleration a and initial velocity u in the first t seconds is:
Vavg=u+at2
Topper's Solved these Questions
- KINEMATICS OF A PARTICLE BOOK - VMC MODULES ENGLISHCHAPTER - KINEMATICS OF A PARTICLE EXERCISE - JEE MAIN (archive)
19 Videos
- KINEMATICS OF A PARTICLE BOOK - VMC MODULES ENGLISHCHAPTER - KINEMATICS OF A PARTICLE EXERCISE - JEE Advanced (archive)
14 Videos
- KINEMATICS OF A PARTICLE BOOK - VMC MODULES ENGLISHCHAPTER - KINEMATICS OF A PARTICLE EXERCISE - LEVEL 1
75 Videos
- JEE MAIN REVISON TEST-23BOOK - VMC MODULES ENGLISHCHAPTER - JEE MAIN REVISON TEST-23EXERCISE - PHYSICS (SECTION 2)
1 Videos
- LAWS OF MOTION BOOK - VMC MODULES ENGLISHCHAPTER - LAWS OF MOTION EXERCISE - IMPECCABLE
53 Videos
Similar Questions
If average velocity of particle moving on a straight line is zero in a time interval, then
View SolutionIdentify the correct graph represeriting the motion of a particle along a straight line With constant acceleration with zero initial velocity.
View SolutionStatement-I : A particle moves in a straight line with constant acceleration. The average velocity of this particle cannot be zero in any time interval.
Statement-II : For a particle moving in straight line with constant acceleration, the average velocity in a time interval is u+v2, where u and v are initial and final velocity of the particle of the given time interval.
View SolutionThe velocity-time graph of a particle moving along a straight line is shown is . The rate of acceleration and deceleration is constant and it is equal to 5ms−2. If the a average velocity during the motion is 20ms−1, Then
 .
.
The value of t is.
View SolutionAssertion: When a particle moves with constant velocity its average velocity, its insttantaneous velocity and its speed are all equal in magnitude.
Reason: If the average velocity of a particle moving on a straight line is zero in a time interval, then it is possible that the instantaneous velocity is never zero in the internal.
View SolutionThe velocity-time graph of a particle moving along a straight line is shown is Fig. The rate of acceleration and deceleration is constant and it is equal to 5ms−2. If the a average velocity during the motion is 20ms−1, Then
 .
.
The maximum velocity of the particle is .
View SolutionThe velocity-time graph of a particle moving along a straight line is shown is Fig. The rate of acceleration and deceleration is constant and it is equal to 5ms−2. If the a average velocity during the motion is 20ms−1, Then
 .
.
The maximum velocity of the particle is .
View SolutionA particle moves in straight line. Acceleration of particle changes with velocity as showns in graph:
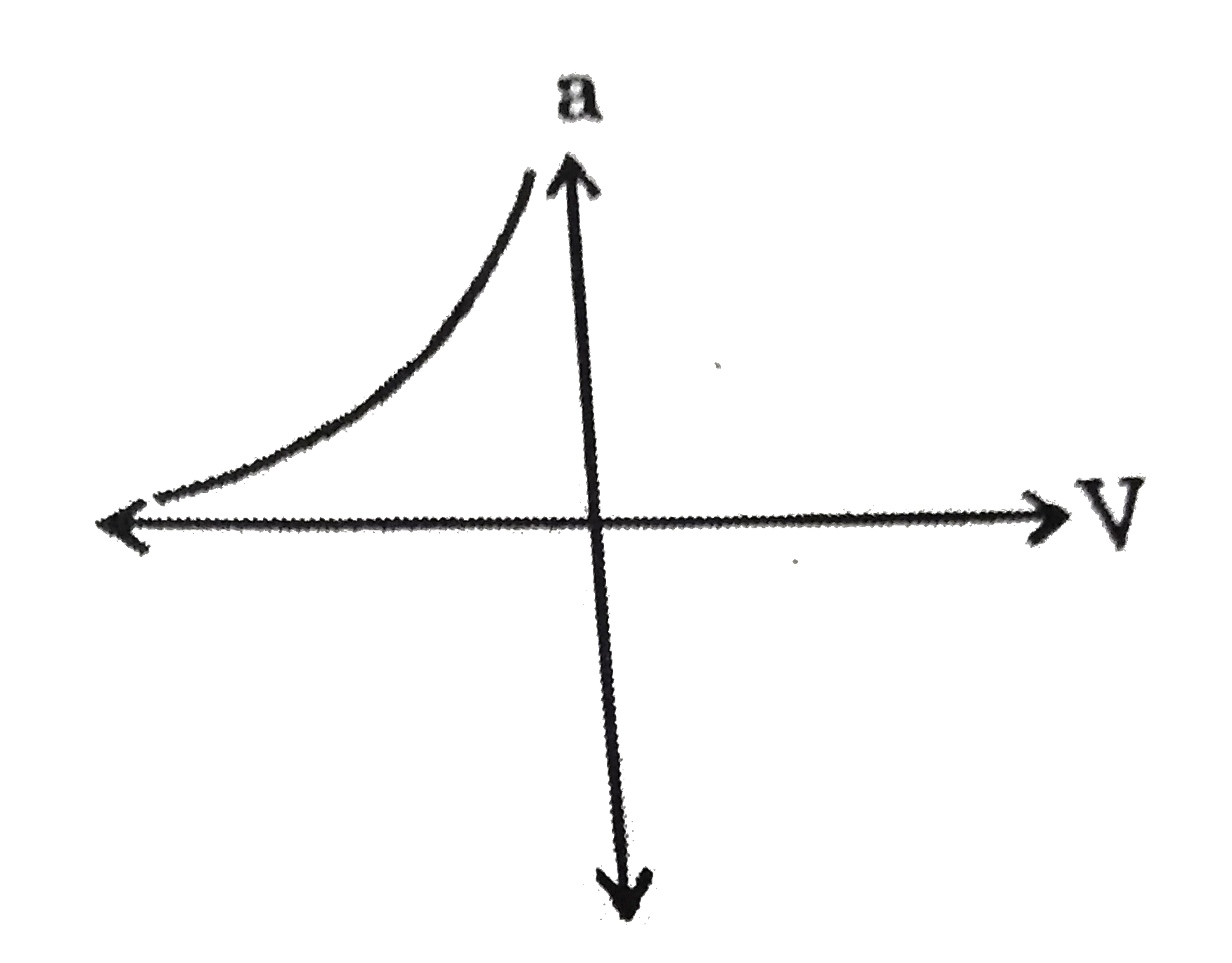
View SolutionA particle is moving along a straight line with constant acceleration. At the end of tenth second its velocity becomes 20m/s and tenth second it travels a distance od 10m. Then the acceleration of the particle will be.
View SolutionThe velocity of a particle moving with constant acceleration at an instant t0 is 10m/s After 5 seconds of that instant the velocity of the particle is 20m/s. The velocity at 3 second before t0 is:-
View Solution
