Derive final velocities V1andV2 after perfectly elastic collision.
To derive the final velocities V1 and V2 after a perfectly elastic collision, we can follow these steps:
Step 1: Define the System
Consider two objects with masses m1 and m2. Let their initial velocities be u1 and u2 respectively. After the collision, their final velocities will be V1 and V2.
Step 2: Apply Conservation of Momentum
Since no external forces are acting on the system, the total linear momentum before the collision is equal to the total linear momentum after the collision. This gives us the equation:
m1u1+m2u2=m1V1+m2V2(1)
Step 3: Apply Conservation of Kinetic Energy
In a perfectly elastic collision, kinetic energy is also conserved. Therefore, we can write:
12m1u21+12m2u22=12m1V21+12m2V22
By simplifying, we get:
m1u21+m2u22=m1V21+m2V22(2)
Step 4: Relate the Velocities
For perfectly elastic collisions, the relative velocity of separation is equal to the relative velocity of approach. This can be expressed as:
V2−V1=−(u2−u1(3)
Step 5: Solve the Equations
Now we have three equations (1), (2), and (3). We can solve these equations to find V1 and V2.
1. From equation (3), we can express V2 in terms of V1:
V2=V1+(u1−u2)(4)
2. Substitute equation (4) into equation (1):
m1u1+m2u2=m1V1+m2(V1+(u1−u2))
This simplifies to:
m1u1+m2u2=(m1+m2)V1+m2(u1−u2)
Rearranging gives:
(m1+m2)V1=m1u1+m2u2−m2(u1−u2)
(m1+m2)V1=m1u1+m2u2−m2u1+m2u2
(m1+m2)V1=(m1−m2)u1+2m2u2
Thus,
V1=(m1−m2)u1+2m2u2m1+m2(5)
3. Now substitute V1 back into equation (4) to find V2:
V2=V1+(u1−u2)
Substituting V1:
V2=(m1−m2)u1+2m2u2m1+m2+(u1−u2)
This simplifies to:
V2=(m1−m2)u1+2m2u2+(u1−u2)(m1+m2)m1+m2
After simplifying, we get:
V2=(m2−m1)u2+2m1u1m1+m2(6)
Final Results
Thus, the final velocities after a perfectly elastic collision are:
V1=(m1−m2)u1+2m2u2m1+m2
V2=(m2−m1)u2+2m1u1m1+m2
Consider two objects with masses m1 and m2. Let their initial velocities be u1 and u2 respectively. After the collision, their final velocities will be V1 and V2.
Since no external forces are acting on the system, the total linear momentum before the collision is equal to the total linear momentum after the collision. This gives us the equation:
In a perfectly elastic collision, kinetic energy is also conserved. Therefore, we can write:
For perfectly elastic collisions, the relative velocity of separation is equal to the relative velocity of approach. This can be expressed as:
Now we have three equations (1), (2), and (3). We can solve these equations to find V1 and V2.
Thus, the final velocities after a perfectly elastic collision are:
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - Level - 187videos
ENERGY & MOMENTUM
- BOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - Level - 1 PARAGRAPH QUESTIONS3videos
ENERGY & MOMENTUM
- BOOK - VMC MODULES ENGLISHCHAPTER - ENERGY & MOMENTUMEXERCISE - LEVEL - 0 -SHORT ANSWER TYPE22videos
ENERGY & MOMENTUM
- BOOK - VMC MODULES ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - JEE Advanced (Archive)89videos
ELECTROSTATICS
- BOOK - VMC MODULES ENGLISHCHAPTER - GASEOUS STATE & THERMODYNAMICSEXERCISE - JEE ADVANCED (ARCHIVE )111videos
GASEOUS STATE & THERMODYNAMICS
Similar Questions
Knowledge Check
- Question 1 - Select One
A sphere P of mass m and velocity vi undergoes an oblique and perfectly elastic collision with an identical sphere Q initially at rest. The angle θ between the velocities of the spheres after the collision shall be
A0B45∘C90∘D180∘ - Question 2 - Select One
Consider the following statements A and B. Identify the correct choice in the given answers
A. In a one dimensional perfectly elastic collision between two moving bodies of equal masses the bodies merely exchange their velocities after collision.
B. If a lighter body at rest suffers perfectly elastic collision with a very heavy body moving with a certain velocity, then after collision both travel with same velocity.AA and B are correctBBoth A and B are wrongCA is correct, B is wrongDA is wrong, B is correct
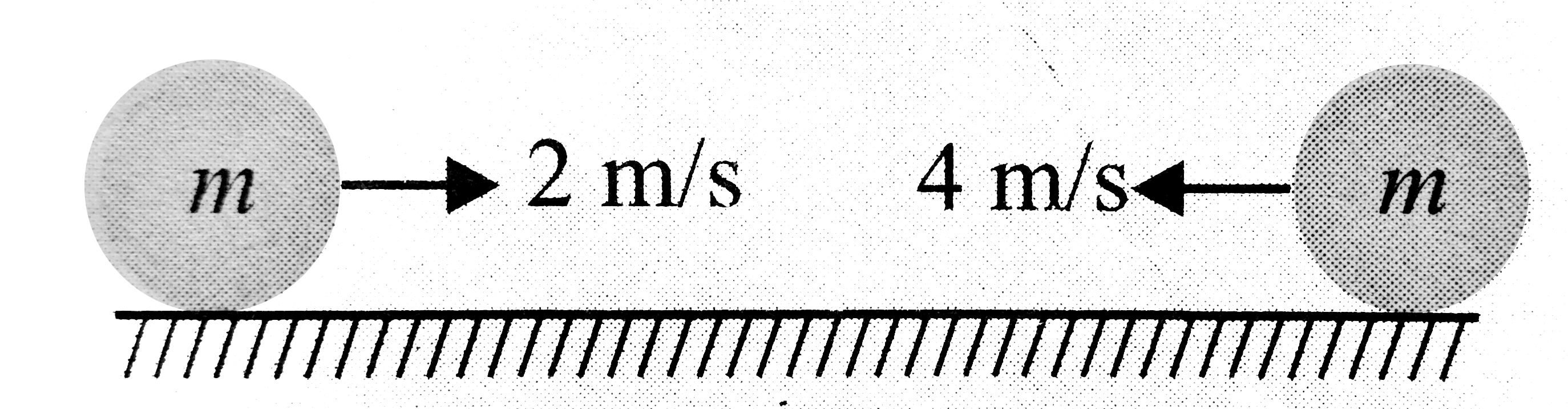
Two identical balls are approaching towards each other on a straight line with velocity 2m/s and 4m/s, respectiely. Find the final velocities after elastic collision between them.
 View Solution
View SolutionTwo perfectly elastic particles A and B of equal masses travelling along a line joining them with velocities 15m/s and 10m/s respectively collide. Their velocities after the elastic collision will be (in m/s) respectively
View SolutionTwo perfectly elastic particles A and B of equal masses travelling along a line joining them with velocities 15m/s and 10m/s respectively collide. Their velocities after the elastic collision will be (in m/s) respectively
View SolutionTwo particles of mass mA and mB and their velocities are VA and VB respectively collides. After collision they interchanges their velocities, then ratio of mAmB is
View SolutionTwo balls of masses m and 2m moving in opposite directions collide head on elastically with velocities v and 2v. Find their velocities after collision.
View SolutionTwo balls of masses m and 2m moving in opposite directions collide head on elastically with velocities v and 2v. Find their velocities after collision.
View Solution

