The coefficient of restitution e for a perfectly elastic collision is
1
∞
Zero
−1
The correct Answer is:C
To determine the coefficient of restitution (e) for a perfectly elastic collision, we can follow these steps:
1. Understanding the Coefficient of Restitution (e):
The coefficient of restitution is defined as the ratio of the relative speed of separation to the relative speed of approach of two colliding bodies. It is given by the formula:
e=Relative speed after collisionRelative speed before collision
2. Analyzing a Perfectly Elastic Collision:
In a perfectly elastic collision, both momentum and kinetic energy are conserved. This means that the objects bounce off each other without any loss of kinetic energy.
3. Relative Speeds in Elastic Collision:
- Before the collision, let the velocities of two colliding bodies be u1 and u2.
- The relative speed before collision is given by:
Relative speed before collision=u1−u2
- After the collision, let their velocities be v1 and v2.
- The relative speed after collision is given by:
Relative speed after collision=v2−v1
4. Applying the Definition:
For a perfectly elastic collision, the relative speed after the collision is equal to the negative of the relative speed before the collision:
v2−v1=−(u1−u2)
This implies:
v2−v1=u2−u1
5. Substituting into the Coefficient of Restitution Formula:
Now substituting the relative speeds into the coefficient of restitution formula:
e=v2−v1u1−u2=−(u1−u2)u1−u2=1
6. Conclusion:
Thus, for a perfectly elastic collision, the coefficient of restitution e is equal to 1.
Final Answer:
The coefficient of restitution e for a perfectly elastic collision is 1.
---
The coefficient of restitution is defined as the ratio of the relative speed of separation to the relative speed of approach of two colliding bodies. It is given by the formula:
e=Relative speed after collisionRelative speed before collision
In a perfectly elastic collision, both momentum and kinetic energy are conserved. This means that the objects bounce off each other without any loss of kinetic energy.
- Before the collision, let the velocities of two colliding bodies be u1 and u2.
- The relative speed before collision is given by:
Relative speed before collision=u1−u2
- After the collision, let their velocities be v1 and v2.
- The relative speed after collision is given by:
Relative speed after collision=v2−v1
For a perfectly elastic collision, the relative speed after the collision is equal to the negative of the relative speed before the collision:
v2−v1=−(u1−u2)
This implies:
v2−v1=u2−u1
Now substituting the relative speeds into the coefficient of restitution formula:
e=v2−v1u1−u2=−(u1−u2)u1−u2=1
Thus, for a perfectly elastic collision, the coefficient of restitution e is equal to 1.
The coefficient of restitution e for a perfectly elastic collision is 1.
Topper's Solved these Questions
- WORK ENERGY AND POWER BOOK - VMC MODULES ENGLISHCHAPTER - WORK ENERGY AND POWER EXERCISE - ENABLE
50 Videos
- WORK ENERGY AND POWER BOOK - VMC MODULES ENGLISHCHAPTER - WORK ENERGY AND POWER EXERCISE - EFFICIENT
50 Videos
- WAVE MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - WAVE MOTIONEXERCISE - JEE ADVANCED ARCHIVE LEVEL 2 (TRUE FALSE TYPE)
4 Videos
Similar Questions
Knowledge Check
An elastic collision conserves
Akinetic energy but not momentumBmomentum but not kinetic energyCneither momentum nor kinetic energyDboth kinetic energy and momentum
In an elastic collision
View SolutionStatement-1 : The coefficient of restitution is less than one for all collision studied under Newton's laws of restituation.
and
Statement-2 : For a perfeclty elastic collision, coefficient of restitution is equal to one .
View SolutionA ball of mass 1kg is dropped from a height of 3.2m on smooth inclined plane. The coefficient of restitution for the collision is e=1/2. The ball's velocity become horizontal after the collision.

View SolutionTwo balls of masses m and 2m and momenta 4p and 2p (in the directions shown) collide as shown in figure. During collsion, the value of linear impulse between them is J. In terms of J and p find coefficient of restitution 'e'. Under what condition collision is elastic. Also find the condition of perfectly inelastic collision.

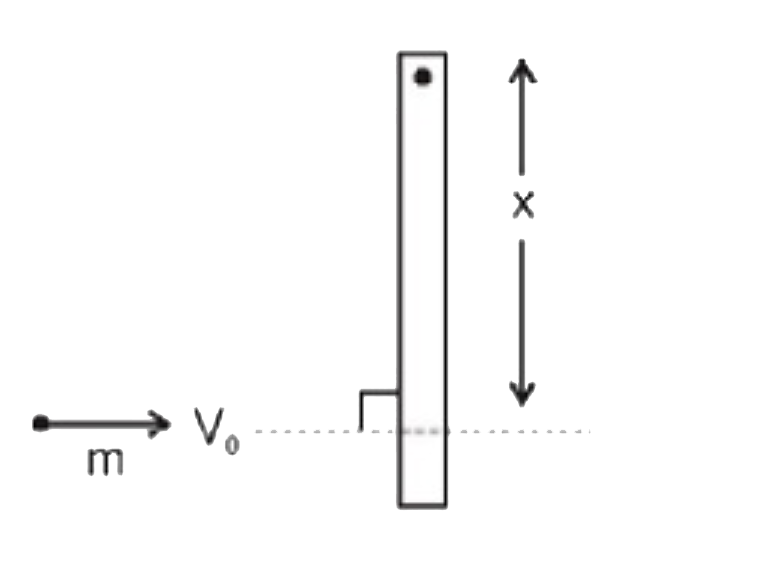
View SolutionA uniform rod of mass m and length L is hinged at one end and free to rotate in the horizontal plane. All the surface are smooth. A particle of same mass m collides with the rod with a speed V0. The coefficient of restitution for the collision is e=12. If hinge reaction during the collision is zero then the value of x is :
View SolutionA 1.0 kg ball drops vertically into a floor from a height of 25 cm . It rebounds to a height of 4 cm. The coefficient of restitution for the collision is
View SolutionA ball is dropped from a height h on a floor. The coefficient of restitution for the collision between the ball and the floor is e. The total distance covered by the ball before it comes to the rest.
View Solution
