Two capillary tubes of the same length but different radii r1 and r2 are fitted in parallel to the bottom of a vessel. The pressure head is ρ. What should be the radius of a single tube that can replace the can replace the two tubes so that the rate of flow is same as before
(17)1/4r
17r
8.5r
√17r
The correct Answer is:A
Radius R of the single tube is given by
πpR48η1=πpr48η1+πp(2r)48η1 or R4=r4+16r4 or R=(17)1/4r which is choice (A).
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - JEE MAIN (LEVEL - 1 )31videos
LIQUIDS
- BOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - JEE ADVANCED (LEVEL -2)55videos
LIQUIDS
- BOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - LEVEL -190videos
LIQUIDS
- BOOK - VMC MODULES ENGLISHCHAPTER - LAWS OF MOTION EXERCISE - IMPECCABLE53videos
LAWS OF MOTION
- BOOK - VMC MODULES ENGLISHCHAPTER - MAGNETIC EFFECTS OF CURRENT EXERCISE - JEE Advanced (Archive)78videos
MAGNETIC EFFECTS OF CURRENT
Similar Questions
Two capillary tubes of lengths in the ratio 2:1 and radii in the ratio 1:2 are connected in series. Assume the flow of the liquid through the tube is steady. Then, the ratio of pressure difference across the tubes is
View Solution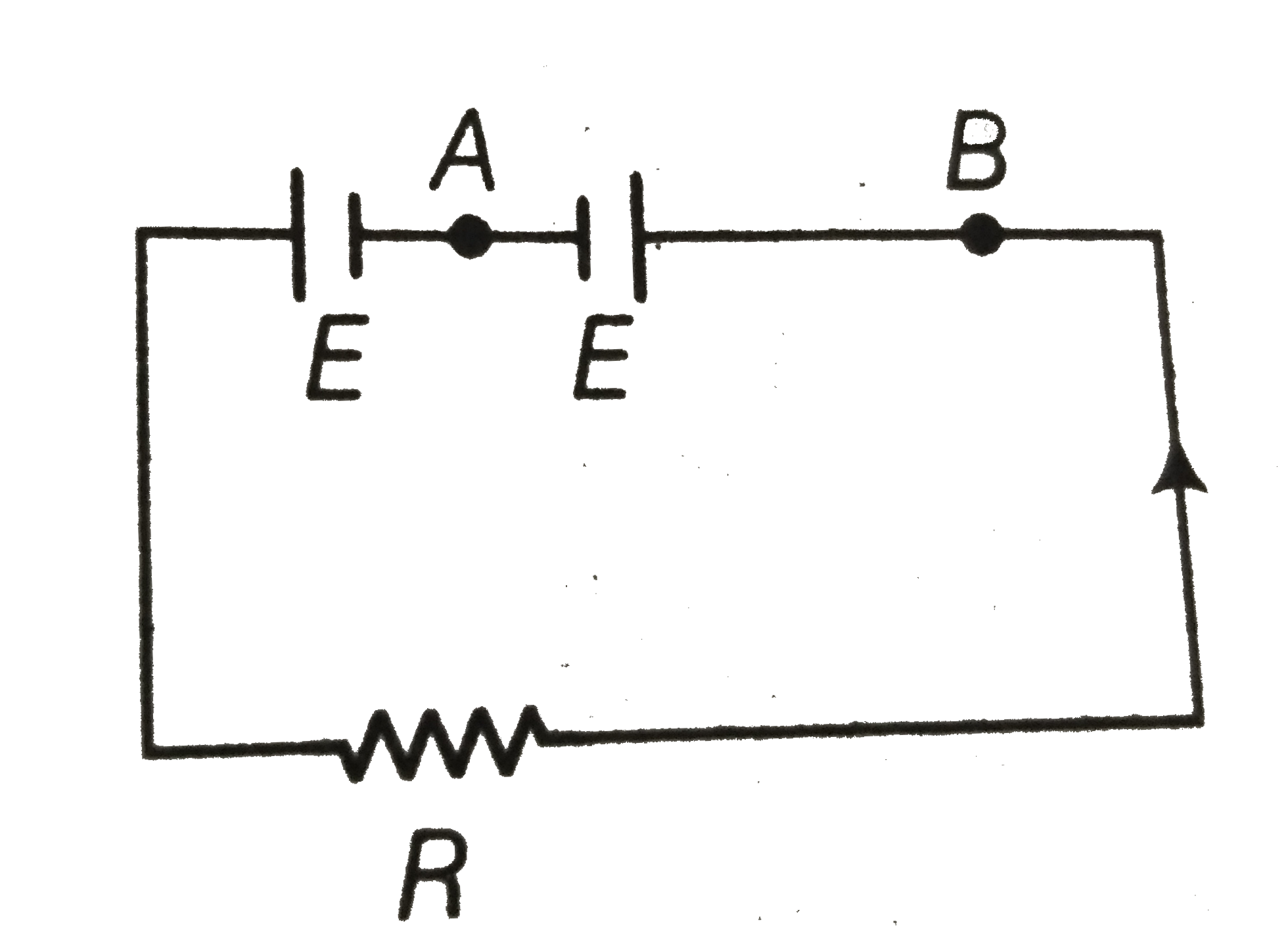
Two cells of same emf E but internal resistance r1andr2 are connected in series to an external resistor R(figure). What should be the value of R so that the potential difference across the terminals of the first cell becomes zero?View Solution
Knowledge Check
- Question 1 - Select One
Two capillary tubes of radii 0.2 cm and 0.4 cm are dipped in the same liquid.The ratio of heights through which liquid will rise in the tube is
A1:2B2:1C1:4D4:1 - Question 2 - Select One
Two capillaries of same length and radii in the ratio 1:2 are connected in series. A liquid flows through them in streamlined condition. If the pressure across the two extreme ends of the combination is 1 m of water, the pressure difference across first capillary is
A9.4 mB4.9 mC0.49 mD0.94 m
A capillary tube is made of glass of refractive index n'. The outer radius of the tube is R. The tube is filled with a liquid of refractive index n<n'. What should be the minimum internal radius of the tube so that any ray that hits the tube would enter the liquid.
View SolutionTwo capillary tubes of lengths in the ratio 2:1 and radii in the ratio 1:2 are connected in series. Assume the flow of the liquid through the tube is steady. Then, the ratio of pressure difference across the tubes is
View Solution
A wire of length 2L, is made by joining two wires A and B of same length but different radii r and 2r and made of the same material. It is vibrating at a frequency such that the joint of the two wires forms a node. If the number of antionodes in wire A is P and that in B is q then the ratio p:q is:View SolutionWe have two (narrow) capillary tubes T1 and T2 . Their lengths are l1 and l2 and radii of cross-section are r1 and r2 respectively. The rate of flow of water under a pressure difference P through tube T is 8cm3/sec. If l1=2l2 and r1=r2 what will be the rate of flow when the two tubes are connected in series and pressure difference across the combinatin is same as before (=P)
View SolutionTwo solid spheres of radii R1 and R2 are made of the same material and have similar surfaces. These are raised to the same temperature and then allowed to cool under identical conditions. The ratio of their initial rates of loss of heat are
View SolutionUnder isothermal condition two soap bubbles of radii r1 and r2 coalesce to form a single bubble of radius r. The external pressure is p0. Find the surface tension of the soap in terms of the given parameters.
View Solution

