A parallel plate capacitor is made of two dielectric blocks in series. One of the blocks has ghickness d1 and dielectric constant K1 and the other has thickness d2 and dielectric constant K2 as shown in figure. This arrangement can be thought as a dielectric slab of thickness d(=d1+d2) and effective dielectric constant K. The is

K1d1+K2d2K1+K2
K1K2(d1+d2)(K1d2+K2d1)
2K1K2K1K2
K1d1+K2d2K1+K3
The correct Answer is:C
1C=1C1+1C2orC=C1C2C1+C2=k1ε0Ad1K2ε0Ad2k1ε0Ad1+K2ε0Ad2=K1K2ε0AK1d2+K2d1.
C=Kε0Ad1+d2⇒K=K1K2(d1+d2)K1d2+K2d1.
Topper's Solved these Questions
- CAPACITORSBOOK - VMC MODULES ENGLISHCHAPTER - CAPACITORSEXERCISE - LEVEL 2
40 Videos
- CAPACITORSBOOK - VMC MODULES ENGLISHCHAPTER - CAPACITORSEXERCISE - JEE Main (Archive) LEVEL-1
36 Videos
- CAPACITORSBOOK - VMC MODULES ENGLISHCHAPTER - CAPACITORSEXERCISE - LEVEL 0 (LONG ANSWER TYPE)
3 Videos
- BASIC MATHEMATICS & VECTORSBOOK - VMC MODULES ENGLISHCHAPTER - BASIC MATHEMATICS & VECTORSEXERCISE - Impeccable
50 Videos
- CURRENT ELECTRICITYBOOK - VMC MODULES ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - IN-CHAPTER EXERCISE-F
10 Videos
Similar Questions
A parallel plate capacitor is made of two dielectric blocks in series. One of the blocks has thickness d1 and dielectric constant K1 and the other has thickness d2 and dielectric constant K2 as shown in figure. This arrangement can be through as a dielectric slab of thickness d(=d1+d2) and effective dielectric constant K. The K is.
 .
.
View SolutionA parallel plate capacitor is made of two dielectric blocks in series. One of the blocks has thickness d1 and dielectric constant K1 and the other has thickness d2 and dielectric constant K2 as shown in figure. This arrangement can be through as a dielectric slab of thickness d(=d1+d2) and effective dielectric constant K. The K is.
 .
.
View SolutionA parallel plate capacitor is made of two dielectric blocks in series. One of the blocks has thickness d1 and dielectric constant K1 and the other has thickness d2 and dielectric constant K2 as shown in figure. This arrangement can be through as a dielectric slab of thickness d(=d1+d2) and effective dielectric constant K. The K is.
 .
.
View SolutionBetween the plates of a parallel plate condenser, a plate of thickness t1 and dielectric constant k1 is placed. In the rest of the space, there is another plate of thickness t2 and dielectric constant k2. The potential difference across the condenser will be
View SolutionBetween the plates of a parallel plate condenser, a plate of thickness t1 and dielectric constant k1 is placed. In the rest of the space, there is another plate of thickness t2 and dielectric constant k2. The potential difference across the condenser will be
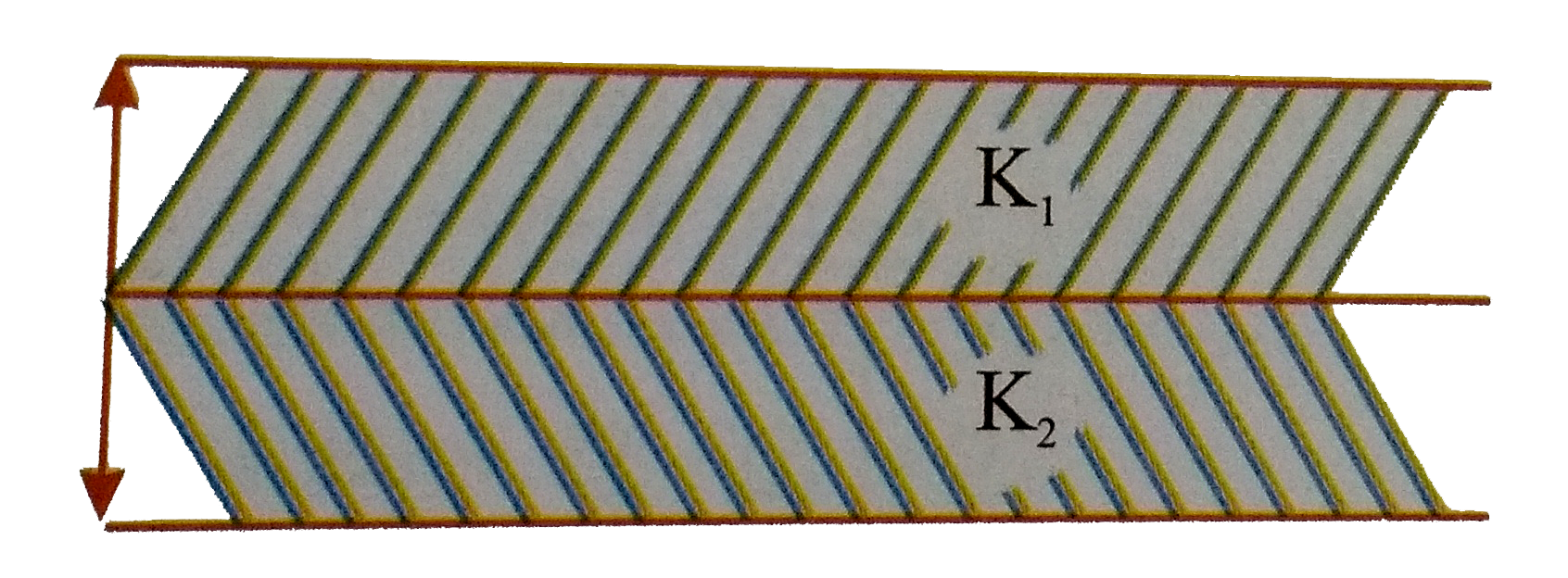
View SolutionA parallel plate capacitor with square plates is filed with four dielectrics of dielectric constants K1,K2,K3,K4, arranged as shown in the figure. The effective dielectric constant K will be :
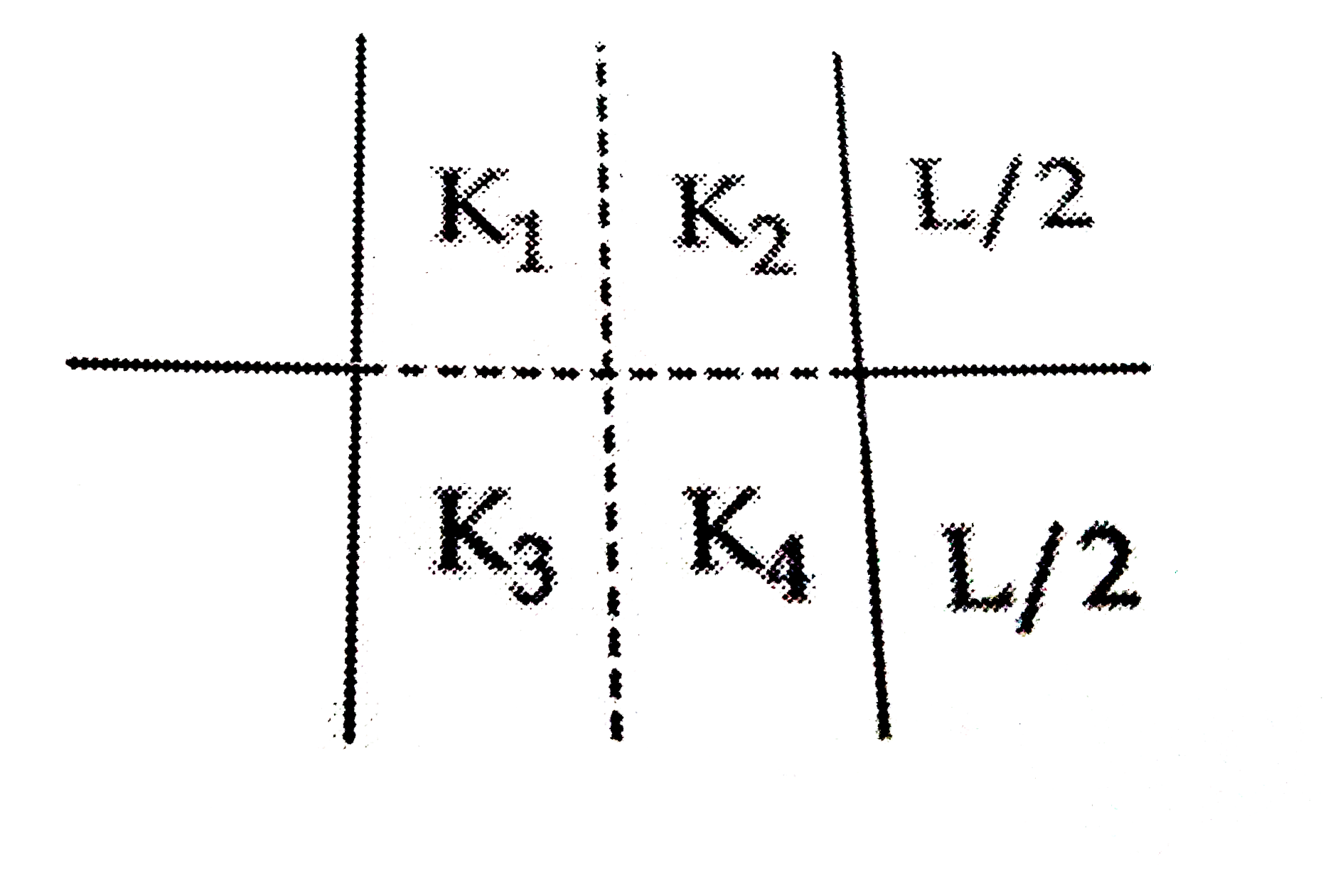
View SolutionA parallel plate capacitor of plate area A and separation d is filled with two materials each of thickness d2 and dielectric constants ∈1 and ∈2 respectively. The equivalent capacitance will be

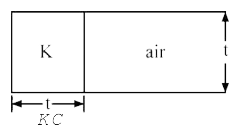
View SolutionA parallel plate capacitor with air as the dielectric has capacitance C. A slab of dielectric constant K and having the same thickness as the separation between plates is introduced so as to fill one-fourth of the capacitor as shown in the figure. The new capacitance will be :
View SolutionA parallel plate capacitor has plate area A and plate separation d. The space betwwen the plates is filled up to a thickness x (ltd) with a dielectric constant K. Calculate the capacitance of the system.

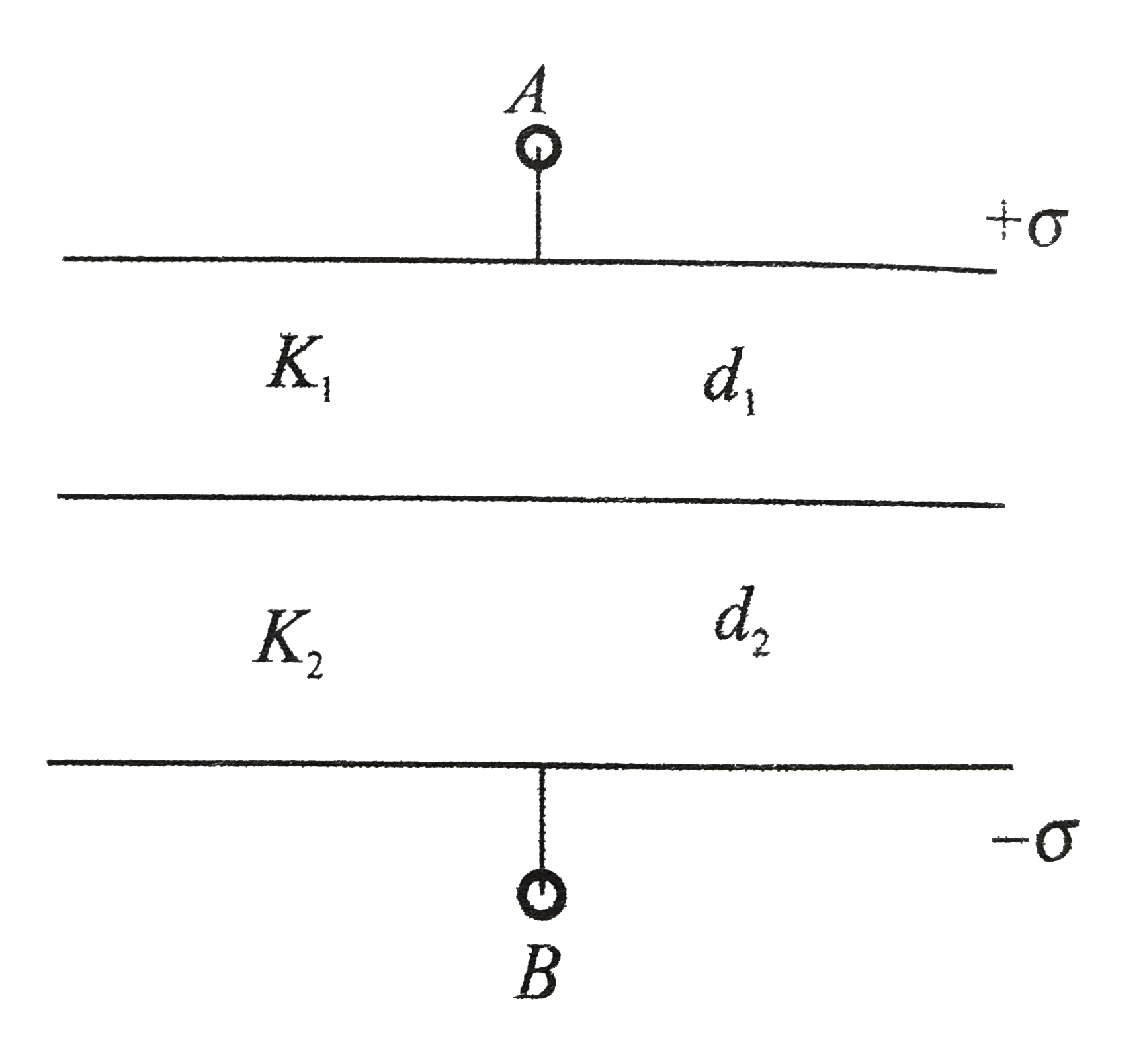
View SolutionFind the capacitance between A and B if two dielectric alabs (each of area A) of dielectric constants K1 and K2 and thicknesses d1 and d2 are inserted between the plates of a parallel plate capacitor of plate area A.
 .
.
View Solution
