The separation between the plates of a parallel-plate capacitor of capacitance 20μF is 2mm. The capacitor is initially uncharged. If a dielectric slab of surface area same as the capacitor, thickness 1mm, and dielectric constant 2 is introduced, and then the new capacitance is?
The correct Answer is:15
To find the new capacitance of the parallel-plate capacitor after introducing a dielectric slab, we can follow these steps:
Step 1: Understand the Initial Setup
The initial capacitance C0 of the capacitor is given as 20μF with a plate separation D=2 mm.
Step 2: Calculate the Area of the Plates
Using the formula for capacitance:
C=ε0AD
where ε0 is the permittivity of free space, A is the area of the plates, and D is the separation between the plates. We can rearrange this to find the area A:
A=C0⋅D/ε0
However, we do not need to calculate A explicitly since we will use it in the next steps.
Step 3: Introduce the Dielectric Slab
A dielectric slab of thickness t=1 mm and dielectric constant k=2 is introduced. The remaining distance between the plates after inserting the dielectric is:
D′=D−t=2 mm−1 mm=1 mm
Step 4: Calculate the New Capacitance
The new capacitance C′ can be calculated considering the two regions: one with the dielectric and one without. The capacitance of the section with the dielectric is given by:
C1=kε0At=2ε0A1 mm
And the capacitance of the section without the dielectric is:
C2=ε0AD′=ε0A1 mm
Since these two capacitances are in series, the total capacitance C′ can be found using the formula for capacitors in series:
1C′=1C1+1C2
Substituting the values:
1C′=12ε0A1 mm+1ε0A1 mm
1C′=1 mm2ε0A+1 mmε0A
1C′=1+22ε0A=32ε0A
Thus,
C′=2ε0A3
Step 5: Relate New Capacitance to Initial Capacitance
Since we know that C0=ε0A2 mm, we can express ε0A in terms of C0:
ε0A=2 mm⋅C0
Substituting this into the equation for C′:
C′=2⋅(2 mm⋅C0)3
C′=4 mm3⋅C0
Step 6: Calculate C′
Now substituting C0=20μF:
C′=4 mm3⋅20μF=80μF3≈26.67μF
Final Answer
The new capacitance C′ is approximately 26.67μF.
---
The initial capacitance C0 of the capacitor is given as 20μF with a plate separation D=2 mm.
Using the formula for capacitance:
C=ε0AD
where ε0 is the permittivity of free space, A is the area of the plates, and D is the separation between the plates. We can rearrange this to find the area A:
A=C0⋅D/ε0
However, we do not need to calculate A explicitly since we will use it in the next steps.
A dielectric slab of thickness t=1 mm and dielectric constant k=2 is introduced. The remaining distance between the plates after inserting the dielectric is:
D′=D−t=2 mm−1 mm=1 mm
The new capacitance C′ can be calculated considering the two regions: one with the dielectric and one without. The capacitance of the section with the dielectric is given by:
C1=kε0At=2ε0A1 mm
And the capacitance of the section without the dielectric is:
C2=ε0AD′=ε0A1 mm
1C′=1C1+1C2
1C′=12ε0A1 mm+1ε0A1 mm
1C′=1 mm2ε0A+1 mmε0A
1C′=1+22ε0A=32ε0A
Thus,
C′=2ε0A3
Since we know that C0=ε0A2 mm, we can express ε0A in terms of C0:
ε0A=2 mm⋅C0
C′=2⋅(2 mm⋅C0)3
C′=4 mm3⋅C0
Now substituting C0=20μF:
C′=4 mm3⋅20μF=80μF3≈26.67μF
The new capacitance C′ is approximately 26.67μF.
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - CAPACITORSEXERCISE - LEVEL 240videos
CAPACITORS
- BOOK - VMC MODULES ENGLISHCHAPTER - CAPACITORSEXERCISE - JEE Main (Archive) LEVEL-136videos
CAPACITORS
- BOOK - VMC MODULES ENGLISHCHAPTER - CAPACITORSEXERCISE - LEVEL 0 (LONG ANSWER TYPE)3videos
CAPACITORS
- BOOK - VMC MODULES ENGLISHCHAPTER - BASIC MATHEMATICS & VECTORSEXERCISE - Impeccable50videos
BASIC MATHEMATICS & VECTORS
- BOOK - VMC MODULES ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - IN-CHAPTER EXERCISE-F10videos
CURRENT ELECTRICITY
Similar Questions
The separation between the plates of a capacitor of capacitance 2μF is 4mm. Initially there is air between the paltes. If two dielectric slabs of area of cross-section same as the capacitor, thickness 2mm each, and dielectric constant 3 and 5 respectively are introduced, the capacitance becomes (in μF)
View SolutionA parallel plate capacitor of capacitance C (without dielectric) is filled by dielectric slabs as shown in figure. Then the new capacitance of the capacitor is
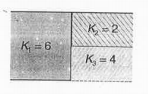 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A parallel plate air capacitor has a capacitance C. When it is half filled with a dielectric of dielectric constant 5, the percentage increase in the capacitance will be
A400%B66.6%C33.3%D200%
If a thin metal foil of same area is placed between the two plates of a parallel plate capacitor of capacitance C, then new capacitance will be
View SolutionIf the separation between the plates of a capacitor is 5 mm , then area of the plate of a 3 F parallel plate capacitor is
View SolutionThe space between the plates of a parallel plate capacitor of capacitance C is filled with three dielectric slabs of identical size as shown in figure. If the dielectric constants of the three slabs are K1,K2andK3 find the new capacitance.
 View Solution
View SolutionA dielectric slab is placed between the plates of a parallel plate capacitor. Its capacitance
A. become zero
B. remains the same
C. decrease
D. increaseView SolutionA parallel plate capacitor, with plate area A and plate separation d, is filled with a dielectric slabe as shown. What is the capacitance of the arrangement ?
View SolutionThe electrostatic energy stored in a parallel-plate capacitor of capacitance 2μF is 10mJ. If the separation between the plates of the capacitor is 1mm, the elctric field inside the capacitor is 10XN/C. The value of X is_____.
View SolutionA parallel-plate of capacitor of capacitance 5μF is connected in a battery of emf 6V. The separation between the plate is 2mm.(a) Find the charge on the positive plate.(b) find the eletric field between the plate.(c) A dielectric slab of thickness 1 mm and dielectric constant 5 is inserted into the gap to occupy the lower half of it.Find the capacitance of the new combination .(d)How much charge has flow n through the battery after the slab is inserted?
View Solution

