Two particles , each of mass m and charge q, are attached to the two ends of a light rigid rod of length 2R . The rod is rotated at constant angular speed about a perpendicular axis passing through its centre. The ratio of the magnitudes of the magnetic moment of the system and its angular momentum about the centre of the rod is
q/2m
q/m
2q/m
q/πm
The correct Answer is:A
To solve the problem, we need to find the ratio of the magnetic moment of the system to its angular momentum about the center of the rod. Let's break down the solution step by step.
Step 1: Understanding the System
We have two particles, each with mass m and charge q, attached to the ends of a rigid rod of length 2R. The rod is rotating with a constant angular speed ω about an axis perpendicular to the rod and passing through its center.
Step 2: Calculate the Current
The current I generated by the rotating charges can be calculated using the formula:
I=q⋅f
where f is the frequency of rotation. The frequency can be expressed in terms of angular speed ω as:
f=ω2π
Thus, the current becomes:
I=q⋅ω2π
Step 3: Calculate the Area of Rotation
Each charge moves in a circular path with radius R. The area A of the circular path is given by:
A=πR2
Step 4: Calculate the Magnetic Moment
The magnetic moment μ of the system can be calculated using the formula:
μ=I⋅A
Substituting the values of I and A:
μ=(q⋅ω2π)⋅(πR2)
This simplifies to:
μ=qωR22
Step 5: Calculate the Angular Momentum
The angular momentum L of the system can be calculated using the moment of inertia I and angular speed ω:
L=I⋅ω
For our system, the moment of inertia I for two point masses at a distance R from the center is:
I=mR2+mR2=2mR2
Thus, the angular momentum becomes:
L=(2mR2)⋅ω
Step 6: Calculate the Ratio of Magnetic Moment to Angular Momentum
Now, we find the ratio of the magnetic moment μ to the angular momentum L:
Ratio=μL=qωR222mR2ω
The R2 and ω terms cancel out:
Ratio=q4m
Final Answer
Thus, the ratio of the magnitudes of the magnetic moment of the system to its angular momentum about the center of the rod is:
q4m
We have two particles, each with mass m and charge q, attached to the ends of a rigid rod of length 2R. The rod is rotating with a constant angular speed ω about an axis perpendicular to the rod and passing through its center.
The current I generated by the rotating charges can be calculated using the formula:
I=q⋅f
where f is the frequency of rotation. The frequency can be expressed in terms of angular speed ω as:
f=ω2π
Thus, the current becomes:
I=q⋅ω2π
Each charge moves in a circular path with radius R. The area A of the circular path is given by:
A=πR2
The magnetic moment μ of the system can be calculated using the formula:
μ=I⋅A
Substituting the values of I and A:
μ=(q⋅ω2π)⋅(πR2)
This simplifies to:
μ=qωR22
The angular momentum L of the system can be calculated using the moment of inertia I and angular speed ω:
L=I⋅ω
For our system, the moment of inertia I for two point masses at a distance R from the center is:
I=mR2+mR2=2mR2
Thus, the angular momentum becomes:
L=(2mR2)⋅ω
Now, we find the ratio of the magnetic moment μ to the angular momentum L:
Ratio=μL=qωR222mR2ω
The R2 and ω terms cancel out:
Ratio=q4m
Thus, the ratio of the magnitudes of the magnetic moment of the system to its angular momentum about the center of the rod is:
q4m
Topper's Solved these Questions
- MAGNETIC EFFECTS OF CURRENT BOOK - VMC MODULES ENGLISHCHAPTER - MAGNETIC EFFECTS OF CURRENT EXERCISE - JEE Main (Archive)
75 Videos
- LIQUIDSBOOK - VMC MODULES ENGLISHCHAPTER - LIQUIDSEXERCISE - JEE ADVANCED (LEVEL -2)
55 Videos
- MOCK TEST 1BOOK - VMC MODULES ENGLISHCHAPTER - MOCK TEST 1EXERCISE - PART I : PHYSICS (SECTION-2)
10 Videos
Similar Questions
Two balls of masses m and 2m are attached to the ends of a light rod of length L. The rod rotates with an angular speed ω about an axis passing through the center of mass of system and perpendicular to the plane. Find the angular momentum of the system about the axis of rotation.
View SolutionA charge q is uniformly distributed on a non-conducting disc of radius R. It is rotated with an angular speed co about an axis passing through the centre of mass of the disc and perpendicular to its plane. Find the magnetic moment of the disc.
View Solution
Knowledge Check
Two masses each of mass M are attached to the end of a rigid massless rod of length L. The moment of interia of the system about an axis passing centre of mass and perpendicular to its length is.
AML2/4BML2/2CML2D2ML2Two thin discs each of mass M and radius r metre are attached to form a rigid body as shown in figure. The rotational inertia of this body about an axis perpendicular to the plane of disc B and passing through its centre is
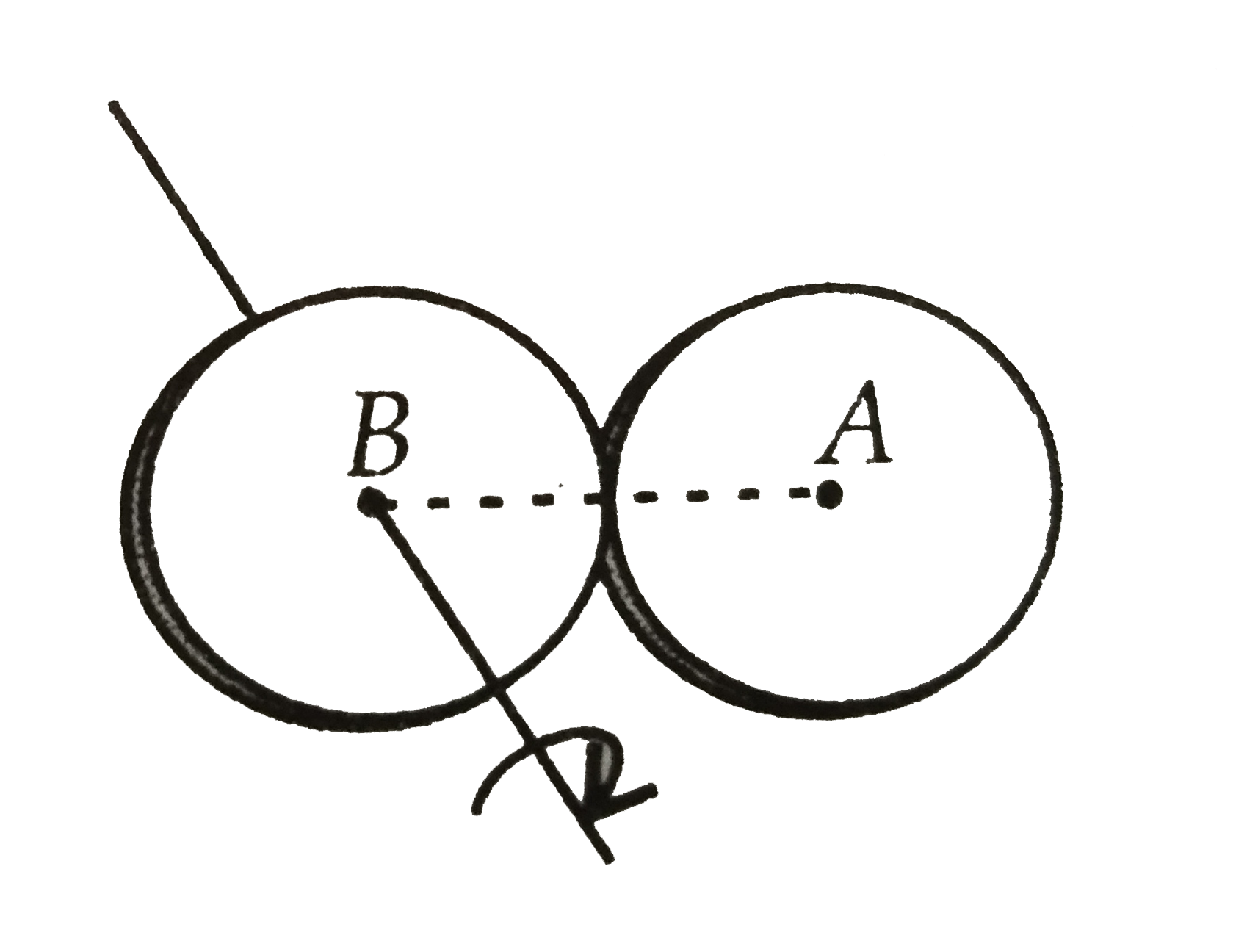 A2Mr2B3Mr2C4Mr2D5Mr2
A2Mr2B3Mr2C4Mr2D5Mr2
The radius of gyration of a uniform rod of length L about an axis passing through its centre of mass is
View SolutionTwo point masses m and 4m are connected by a light rigid rod of length l at the opposite ends. Moment of inertia of the system about an axis perpendicular to the length and passing through the centre of mass is
View SolutionTwo particles of masses m1andm2 are joined by a light rigid rod of length r. The system rotates at an angular speed ω about an axis through the centre of mass of the system and perpendicular to the rod. Show that the angular momentum of the system is L=μr2ω where μ is the reduced mass of the system defined as μ=m1m2m1+m2
View SolutionTwo small balls A and B each of mass m, are attached tightly to the ends of a light rod of length d. The structure rotates about the perpendicular bisector of the rod at an angular speed ω. Calculate the angular momentum of the individual balls and of the system about the axis of rotation.
View SolutionA disc of mass m, radius r and carrying charge q, is rotating with angular speed ω about an axis passing through its centre and perpendicular to its plane. Calculate its magnetic moment
View SolutionA disc of mass m, radius r and carrying charge q, is rotating with angular speed ω about an axis passing through its centre and perpendicular to its plane. Calculate its magnetic moment
View Solution
