The base BC of a triangle ABC is bisected at the point (a, b) and equation to the sides AB and AC are respectively ax+by=1 and bx+ay=1 Equation of the median through A is:
ax−by=ab
(2b−1)(ax+by)=ab
(2ab−1)(ax+by−1)=(a2+b2−1)(bx+ay−1)
bx−ay=1
The correct Answer is:C
To find the equation of the median through point A in triangle ABC, we start with the given information:
1. The base BC of triangle ABC is bisected at the point (a, b).
2. The equations of the sides AB and AC are given as:
- L1:ax+by=1
- L2:bx+ay=1
Step 1: Identify the equations of the lines
The equations of the lines are:
- L1:ax+by−1=0
- L2:bx+ay−1=0
Step 2: Find the point of intersection (A)
The point A is the intersection of lines L1 and L2. To find the coordinates of point A, we can solve these two equations simultaneously.
From L1:
ax+by=1(1)
From L2:
bx+ay=1(2)
Step 3: Solve the equations
We can multiply equation (1) by b and equation (2) by a:
b(ax+by)=b⇒abx+b2y=b(3)
a(bx+ay)=a⇒abx+a2y=a(4)
Now, subtract equation (4) from equation (3):
(b2y−a2y)=b−a
(b2−a2)y=b−a
y=b−ab2−a2=b−a(b−a)(b+a)=1b+a(if b≠a)
Substituting y back into equation (1) to find x:
ax+b(1b+a)=1
ax+bb+a=1
ax=1−bb+a
ax=(b+a)−bb+a=ab+a
x=1b+a(if a≠0)
Thus, the coordinates of point A are:
A(1b+a,1b+a)
Step 4: Equation of the median AD
The median AD will pass through point A and the midpoint D of BC, which is given as (a, b).
The slope of line AD can be calculated as:
slope=b−1b+aa−1b+a=b(b+a)−1a(b+a)−1
Using the point-slope form of the line equation:
y−y1=m(x−x1)
where (x1,y1)=A(1b+a,1b+a) and m is the slope calculated above.
The equation of the median AD becomes:
y−1b+a=b(b+a)−1a(b+a)−1(x−1b+a)
Final Equation
After simplifying, we will arrive at the final equation of the median AD.
2. The equations of the sides AB and AC are given as:
- L1:ax+by=1
- L2:bx+ay=1
The equations of the lines are:
- L1:ax+by−1=0
- L2:bx+ay−1=0
The point A is the intersection of lines L1 and L2. To find the coordinates of point A, we can solve these two equations simultaneously.
ax+by=1(1)
From L2:
bx+ay=1(2)
We can multiply equation (1) by b and equation (2) by a:
b(ax+by)=b⇒abx+b2y=b(3)
a(bx+ay)=a⇒abx+a2y=a(4)
(b2y−a2y)=b−a
(b2−a2)y=b−a
y=b−ab2−a2=b−a(b−a)(b+a)=1b+a(if b≠a)
ax+b(1b+a)=1
ax+bb+a=1
ax=1−bb+a
ax=(b+a)−bb+a=ab+a
x=1b+a(if a≠0)
A(1b+a,1b+a)
The median AD will pass through point A and the midpoint D of BC, which is given as (a, b).
slope=b−1b+aa−1b+a=b(b+a)−1a(b+a)−1
y−y1=m(x−x1)
where (x1,y1)=A(1b+a,1b+a) and m is the slope calculated above.
y−1b+a=b(b+a)−1a(b+a)−1(x−1b+a)
After simplifying, we will arrive at the final equation of the median AD.
Topper's Solved these Questions
- STRAIGHT LINESBOOK - VMC MODULES ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - Level -2
43 Videos
- STRAIGHT LINESBOOK - VMC MODULES ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - Level -2 Passage
5 Videos
- STRAIGHT LINESBOOK - VMC MODULES ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - Level - 0 (True or False)
7 Videos
- SEQUENCE AND SERIES BOOK - VMC MODULES ENGLISHCHAPTER - SEQUENCE AND SERIES EXERCISE - JEE MAIN & Advance ( ARCHIVE)
46 Videos
- THREE DIMENSIONAL GEOMETRY BOOK - VMC MODULES ENGLISHCHAPTER - THREE DIMENSIONAL GEOMETRY EXERCISE - JEE ADVANCED (ARCHIVE)
34 Videos
Similar Questions
In a triangle ABC, if A is (1,2) and the equations of the medians through B and c are x+y=5 and x=4 respectively then B must be:
View SolutionIn an isosceles triangle ABC, the coordinates of the points Band C on the base BC are respectively (1,2) and (2.1). If the equation of the line AB is y=2x, then the equation of the line AC is
View SolutionD is the mid-point of side BC of a triangle ABC.AD is bisected at the point E and BE produced cuts AC at the point X. Prove that BE:EX=3:1.
View SolutionLet the area of a given triangle ABC be Δ. Points A1,B1,andC1 , are the mid points of the sides BC,CA and AB respectively. Point A2 is the mid point of CA1. Lines C1A1andAA2 meet the median BB1 points E and D respectively. If Δ1 be the area of the quadrilateral A1A2DE, using vectors or otherwise find the value of Δ1Δ
View SolutionIn ΔABC,D,E and F are mid-point of sides AB ,BC and AC respectively , Prove that AE and DF bisect each other.
View SolutionIn the figure, given, ABC is a triangle and BC is parallel to the y-axis. AB and AC intersect the y-axis at P and Q respectively.
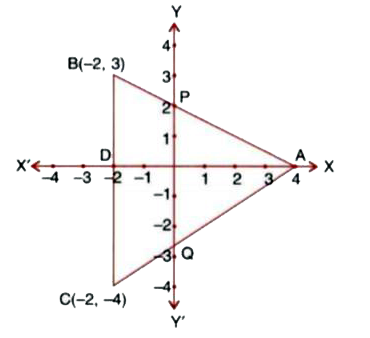
Find the equation of the line AC.
View SolutionABC is a triangle A line is drawn parallel to BC to meet AB and AC in D and E respectively. Prove that the median through A bisects DE.
View SolutionFind the equation of the side BC of the triangle ABC whose vertices are A(−1,−2), B(0,1)andC(2,0) respectivley. Also, find the equation of the median through A(−1, −2).
View SolutionIn a triangle ABC , if the equation of sides AB,BC and CA are 2x−y+4=0,x−2y−1=0andx+3y−3=0 respectively ,
The equation of external bisector of angle B is
View SolutionIn ΔABC,AB=AC.D,Eand F are mid-points of the sides BC, CA and AB respectively . Show that :
AD and FE bisect each other.
View Solution
