A small sphere of mass 1 kg is rolling without slipping with linear speed
v=√2007m/s

It leaves the inclined plane at point C.
Find the linear speed at point C.
√1007m/s
√507m/s
√10035m/s
√20035m/s
The correct Answer is:A
In case of solid sphere, KRKT=25. If pure rolling (v=Rω) is taking place.
∴ Energy at bottom EB=75KT=75(12mv2)=75×12×(√2007)2=20J
Kinetic energy at top KC=EB−mgh=20−(1)(10)(1)=10J
Again the ratio of KRKT will be 25
∴KT=57×10=507J or 12mv2c=507∴vc=√1007m/n (as m=1 kg)
Topper's Solved these Questions
- ROTATIONAL MOTION BOOK - VMC MODULES ENGLISHCHAPTER - ROTATIONAL MOTION EXERCISE - LEVEL - 2 (Angular Momentum, Collision)
20 Videos
- ROTATIONAL MOTION BOOK - VMC MODULES ENGLISHCHAPTER - ROTATIONAL MOTION EXERCISE - JEE MAIN (ARCHIVE)
58 Videos
- ROTATIONAL MOTION BOOK - VMC MODULES ENGLISHCHAPTER - ROTATIONAL MOTION EXERCISE - LEVEL - 2 (Torque, Fixed and Rotation)
10 Videos
- REVISION TEST-2 JEEBOOK - VMC MODULES ENGLISHCHAPTER - REVISION TEST-2 JEEEXERCISE - PHYSICS
25 Videos
- SIMPLE HARMONIC MOTION BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - 7-previous year question
46 Videos
Similar Questions
A small sphere of mass 1 kg is rolling without slipping with linear speed
v=√2007m/s

It leaves the inclined plane at point C.
Find ratio of rotational and translational kinetic energy of the sphere when it strikes the ground after leaving from point C.
View Solution
A ring of mass m is rolling without slipping with linear speed v as shown in figure. Four particles each of mass m are also attached at points A, B , C and D find total kinetic energy of the system.
View SolutionA circular disc of mass 2 kg and radius 10 cm rolls without slipping with a speed 2 m/s. The total kinetic energy of disc is
View SolutionA solid sphere of mas 2kg rolls on a table with linear speed of 1m/s. Its total kinetic energy is
View SolutionA solid sphere of mass m rolls without slipping on an inclined plane of inclination θ. The linear acceleration of the sphere is
View SolutionA sphere of mass m rolls without slipping on an inclined plane of inclination θ. Find the linear acceleration of the sphere and the force of friction acting on it. What should be the minimum coefficient of static friction to support pure rolling?
View Solution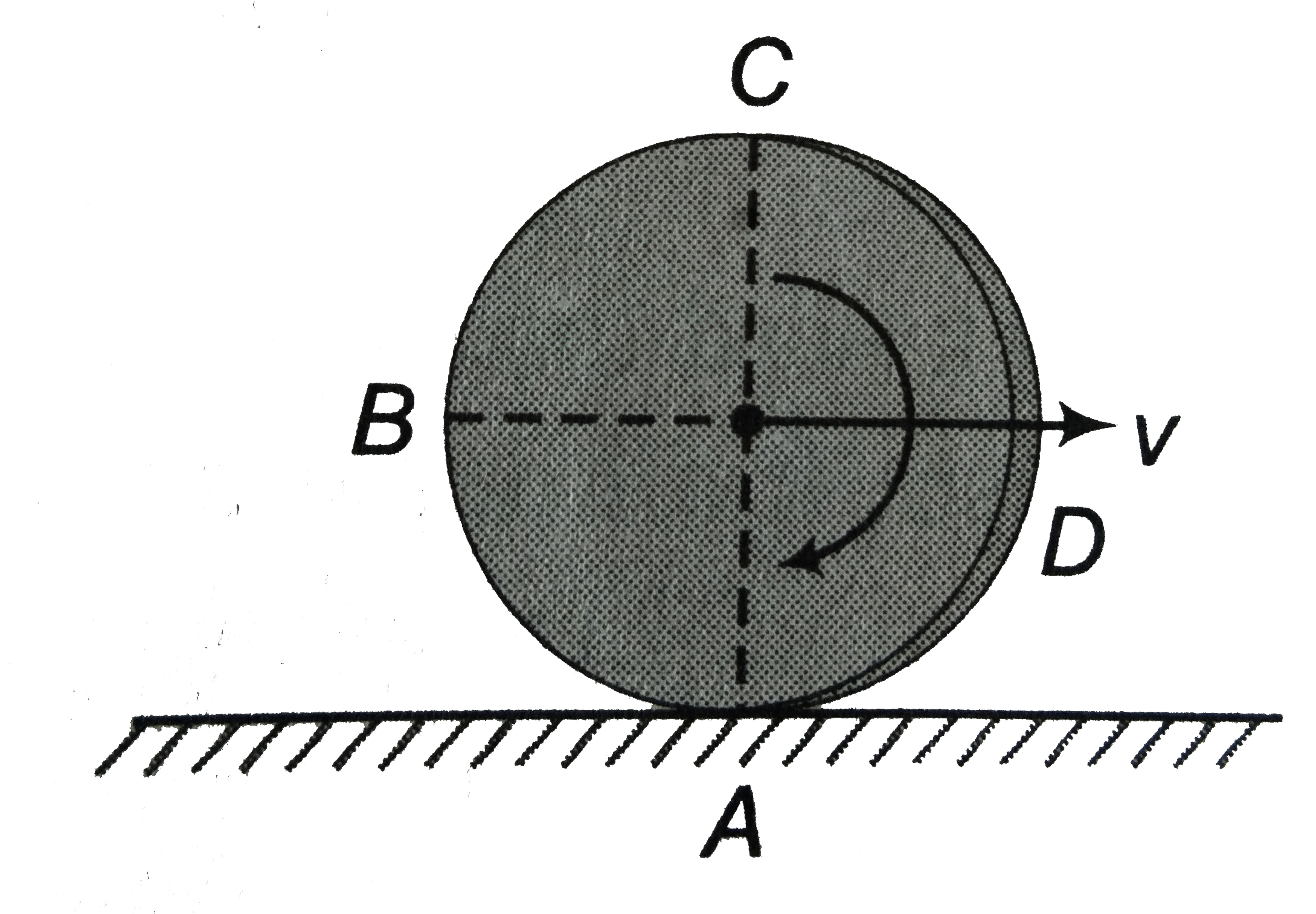
A disc is rolling without slipping with linear velocity v as shown in figure. With the concept of instantaneous axis of rotation, find velocities of point A, B, C and D.
View SolutionA thick walled hollow sphere has outer radius R. It down an inclined plane without slipping and its -speed the bottom is v. If the inclined plane is frictionless and the sphere slides down without rolling, its speed at the bottom 5v/4. What is the radius of gyration of the sphere?
View SolutionA hoop rolls on a horizontal ground without slipping with linear speed v. Speed of a particle P on the circumference of the hoop at angle θ is :
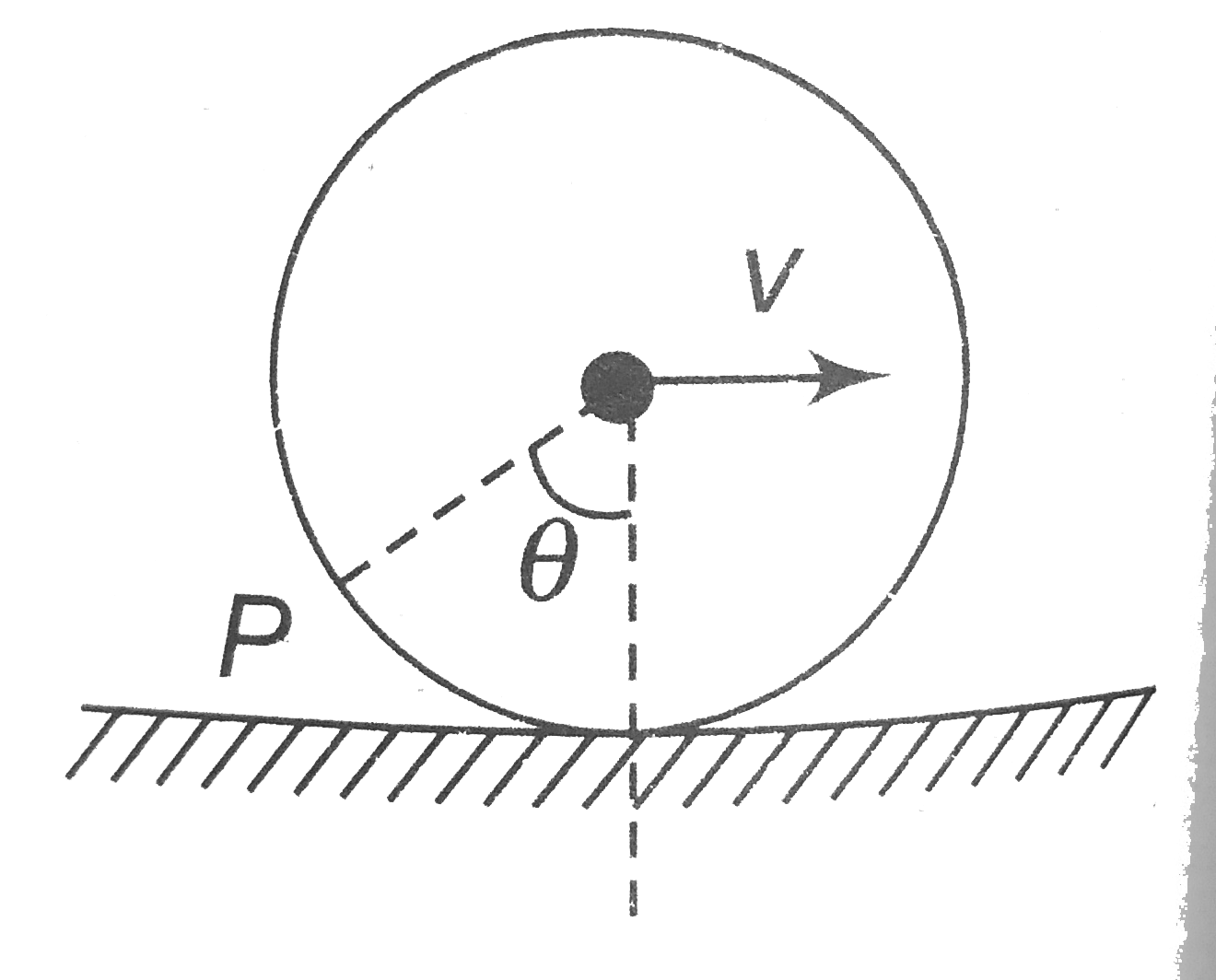
View SolutionA uniform sphere of mass 200 g rolls without slipping on a plane surface so that its centre moves at a speed of 2.00 cm/s. Find its kinetic energy.
View Solution
