A uniform disc of radius R is spinned to the angular velocity ω and then carefully placed on a horizontal surface. How long will the disc be rotating on the surface if the friction coeffiecient is equal to k? The pressure exerted by the disc on the surface can be regarded as uniform.
4ωR3μg
3ωR4μg
ωR3μg
4ωRμg
The correct Answer is:B
To solve the problem of how long a uniform disc of radius R spinning with an angular velocity ω will continue to rotate on a horizontal surface with a friction coefficient k, we can follow these steps:
Step 1: Identify Forces Acting on the Disc
When the disc is placed on the surface, the forces acting on it include:
- The gravitational force mg acting downward.
- The normal force N acting upward.
- The frictional force Ff acting tangentially at the edge of the disc, opposing the motion.
Since there is no vertical acceleration, we can write:
N=mg
Step 2: Determine the Frictional Force
The frictional force can be expressed in terms of the coefficient of friction k:
Ff=kN=k(mg)
Step 3: Calculate the Torque Due to Friction
The frictional force creates a torque τ about the center of the disc. The torque due to the frictional force is given by:
τ=Ff⋅R=k(mg)⋅R
Step 4: Relate Torque to Angular Acceleration
According to Newton's second law for rotation, the net torque is also related to angular acceleration α by:
τ=Iα
where I is the moment of inertia of the disc. For a uniform disc, the moment of inertia is:
I=12mR2
Setting the two expressions for torque equal gives:
k(mg)R=(12mR2)α
Step 5: Solve for Angular Acceleration
We can simplify this equation to find α:
α=2kgR
Step 6: Use Angular Motion Equation
Since the disc starts with an initial angular velocity ω and comes to rest, we can use the angular motion equation:
ωf=ωi+αt
where ωf=0 (final angular velocity), ωi=ω (initial angular velocity), and α is negative (deceleration):
0=ω−(2kgR)t
Step 7: Solve for Time t
Rearranging the equation gives:
t=ωR2kg
Final Answer
Thus, the time for which the disc will continue to rotate on the surface is:
t=ωR2kg
---
When the disc is placed on the surface, the forces acting on it include:
- The gravitational force mg acting downward.
- The normal force N acting upward.
- The frictional force Ff acting tangentially at the edge of the disc, opposing the motion.
N=mg
The frictional force can be expressed in terms of the coefficient of friction k:
Ff=kN=k(mg)
The frictional force creates a torque τ about the center of the disc. The torque due to the frictional force is given by:
τ=Ff⋅R=k(mg)⋅R
According to Newton's second law for rotation, the net torque is also related to angular acceleration α by:
τ=Iα
where I is the moment of inertia of the disc. For a uniform disc, the moment of inertia is:
I=12mR2
k(mg)R=(12mR2)α
We can simplify this equation to find α:
α=2kgR
Since the disc starts with an initial angular velocity ω and comes to rest, we can use the angular motion equation:
ωf=ωi+αt
where ωf=0 (final angular velocity), ωi=ω (initial angular velocity), and α is negative (deceleration):
0=ω−(2kgR)t
Rearranging the equation gives:
t=ωR2kg
Thus, the time for which the disc will continue to rotate on the surface is:
t=ωR2kg
Topper's Solved these Questions
- ROTATIONAL MOTION BOOK - VMC MODULES ENGLISHCHAPTER - ROTATIONAL MOTION EXERCISE - JEE MAIN (ARCHIVE)
58 Videos
- ROTATIONAL MOTION BOOK - VMC MODULES ENGLISHCHAPTER - ROTATIONAL MOTION EXERCISE - JEE Advanced (Archive) (SINGLE OPTION CORRECT TYPE)
26 Videos
- ROTATIONAL MOTION BOOK - VMC MODULES ENGLISHCHAPTER - ROTATIONAL MOTION EXERCISE - LEVEL - 2 (General rigid body motion, Rolling)
10 Videos
- REVISION TEST-2 JEEBOOK - VMC MODULES ENGLISHCHAPTER - REVISION TEST-2 JEEEXERCISE - PHYSICS
25 Videos
- SIMPLE HARMONIC MOTION BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - 7-previous year question
46 Videos
Similar Questions
A ring of radius R is first rotated with an angular velocity ω and then carefully placed on a rough horizontal surface. The coefficient of friction between the surface and the ring is μ. Time after which its angular speed is reduced to half is
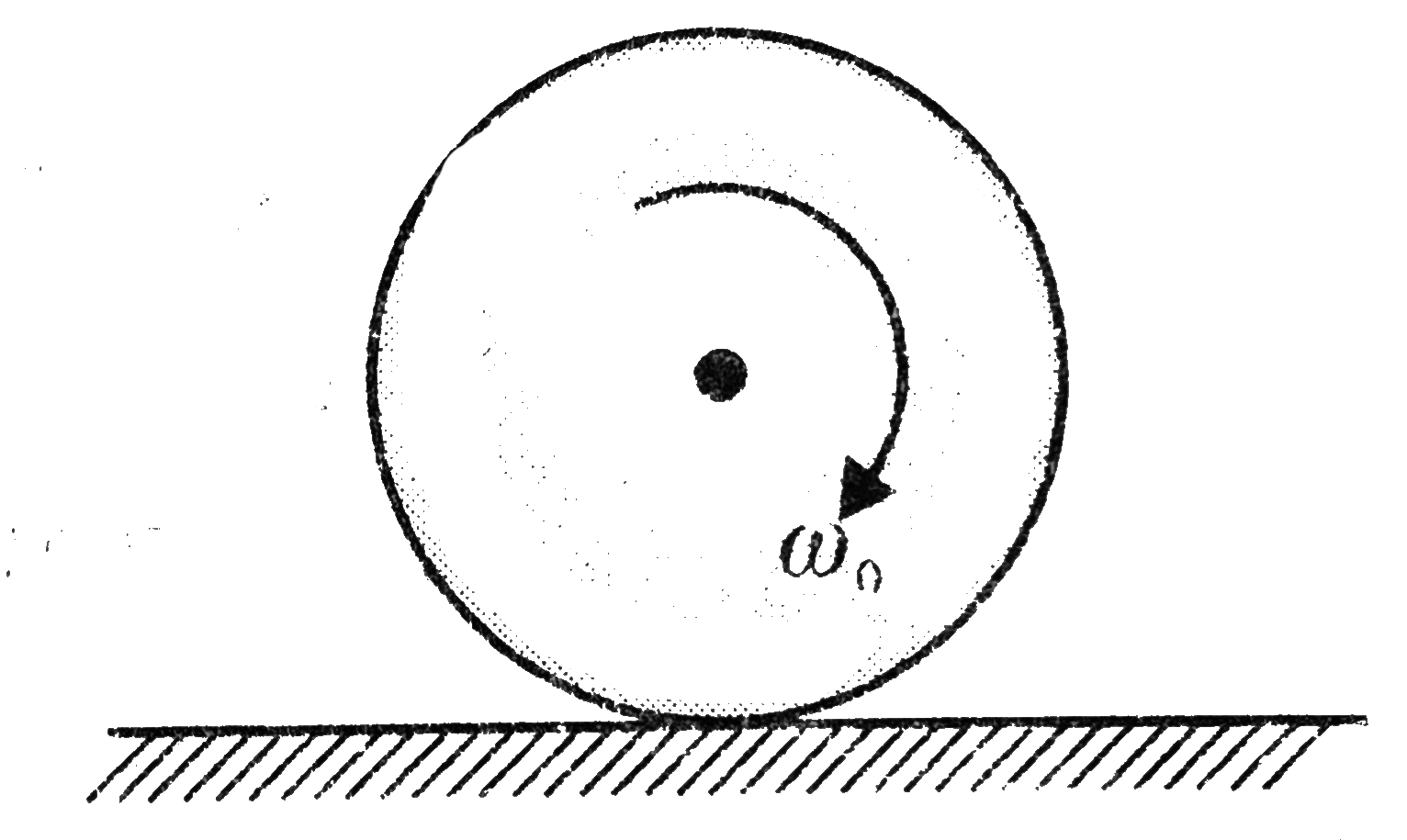
View SolutionA disc is given an initial angular velocity ω0 and placed on a rough horizontal surface as shown Fig. The quantities which will not depend on the coefficient of friction is/are
View SolutionA uniform disc of radius r0 lies on a smooth horizontal plane. A similar disc spinning with the angular velocity ω0 is carefully lowered onto the first disc. How soon do both discs spin with the same angular-velocity if the friction coefficient between them is equal to μ?
View SolutionWe have a disc of neglligible thickness and whose surface mass density varies as radial distance from centre as σ=σ0(1+rR), where R is the radius of the disc. Specific heat of the material of the disc isC.
Disc is given an angular velocity ω0 and placed on a horizontal rough surface such that the plane of the disc is parallel to the surface. Coefficient of friction between disc and suface is μ. The temperature of the disc is T0.
Answer the following question on the base of information provided in the above paragraph
If the kinetic energy lost due to the friction between disc and surface is absorbed by the disc the moment heat is generated, by the mass lying at the point where the energy is lost. Assume that there is no radial heat flow in betwen disc particles. Rate of change in temperature at a point lying at r distance away from centre with time, before disc stops rotating, will be (α-magnitude of angular acceleration of rod)
View SolutionWe have a disc of neglligible thickness and whose surface mass density varies as radial disance from centre as σ=σ0(1+rR), where R is the radius of the disc. Specific heat of the material of the disc isC.
Disc is given an angular velocity ω0 and placed on a horizontal rough surface such that the plane of the disc is parallel to the surface. Coefficient of friction between disc and suface is μ. The temperature of the disc is T0.
Answer the following question on the base of information provided in the above paragraph
Magnitude of angular acceleration of disc is
View SolutionA uniiform disc of radius r spins with angular velocity ω and angular acceleration α. If the centre of mass of the disc has linear acceleration a, find the magnitude and direction of aceeleration of the point 1,2, and 3.
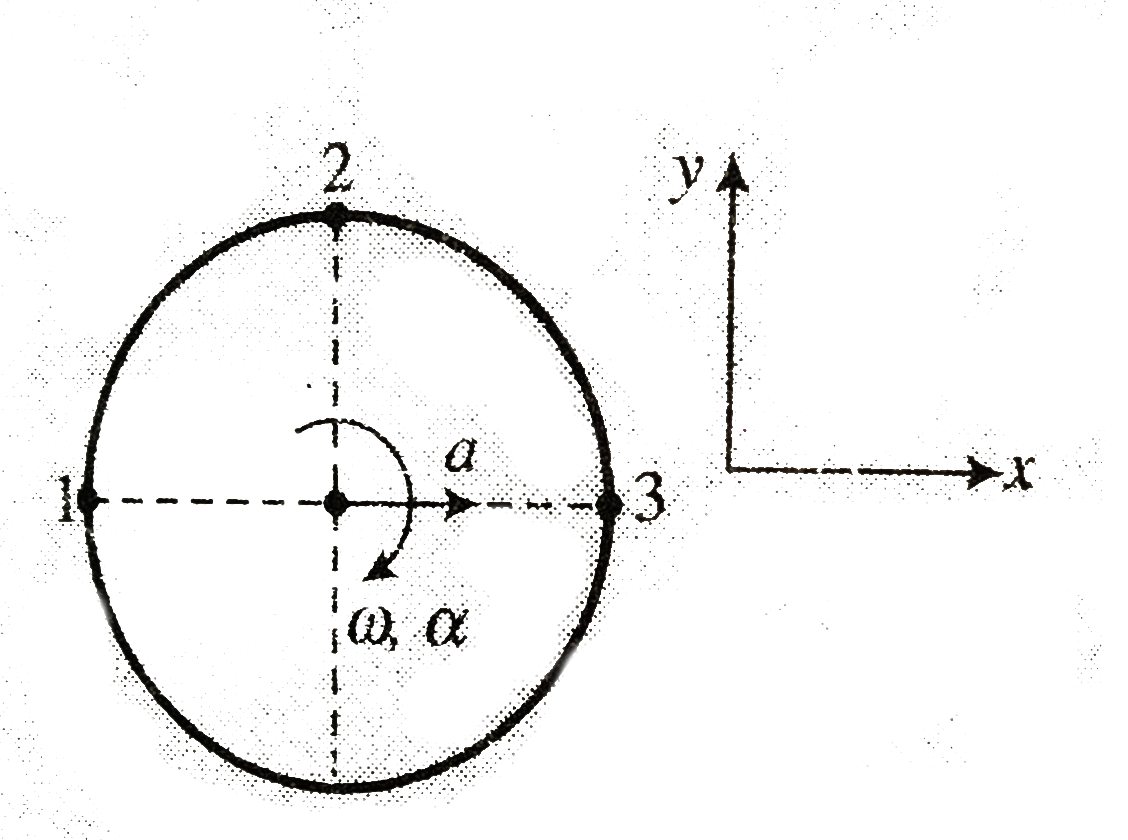
View SolutionA uniform disc of mass m and radius R is rotated about an axis passing through its center and perpendicular to its plane with an angular velocity ω. It is placed on a rough horizontal plane with the axis of the disc keeping vertical. Coefficient of friction between the disc and the surface is μ, find
(a). The time when disc stops rotating
(b). The angle rotated by the disc before stopping.
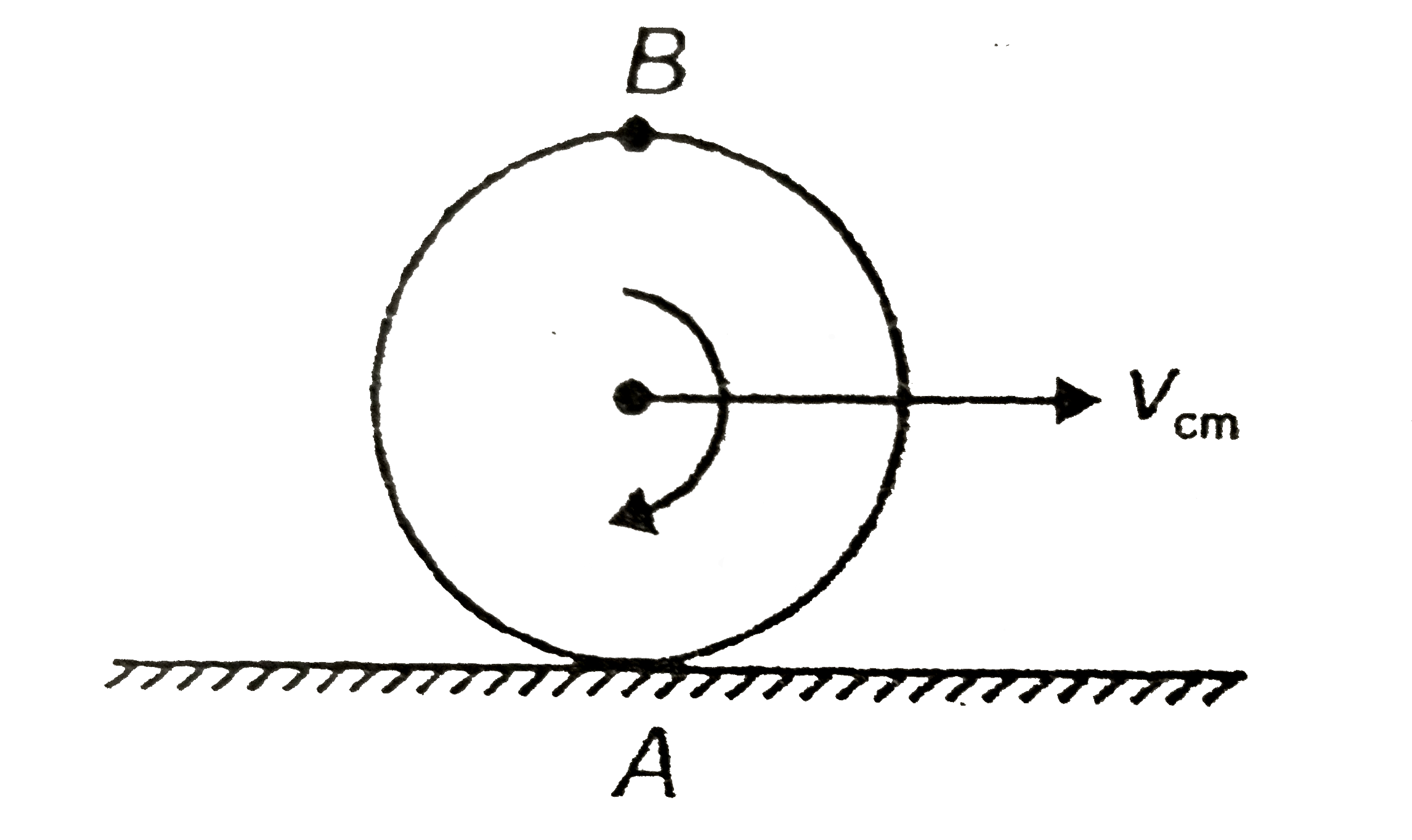
View SolutionA disc is undergoing rolling on a horizontal surface. Which of the statement about angular momentum of the disc is incorrect ?
View SolutionA disc of mass m and radius R rotating with angular speed ω0 is placed on a rough surface (co-officient of friction =μ). Then

View SolutionA solid uniform disc of mass m rols without slipping down a fixed inclined plank with an acceleration a. The frictional force on the disc due to surface of the plane is
View Solution
