The speed (v) of ripples on the surface of waterdepends on surface tension (σ), density (ρ) and wavelength (λ). The square of speed (v) is proportional to
σρλ
ρσλ
λσρ
ρλσ
The correct Answer is:A
To solve the problem of determining how the square of the speed v of ripples on the surface of water depends on surface tension σ, density ρ, and wavelength λ, we will use dimensional analysis. Here’s a step-by-step solution:
Step 1: Identify the relationship
We know that the speed v depends on σ, ρ, and λ. We can express this relationship as:
v∝σA⋅ρB⋅λC
where A, B, and C are the powers we need to determine.
Step 2: Write the dimensions
We need to write down the dimensions of each quantity:
- The dimension of speed v is (v)=M0L1T−1.
- The dimension of surface tension σ is (σ)=M1T−2.
- The dimension of density ρ is (ρ)=M1L−3.
- The dimension of wavelength λ is (λ)=L1.
Step 3: Write the dimensional equation
Now we can substitute the dimensions into our proportionality:
(M0L1T−1)=(M1T−2)A⋅(M1L−3)B⋅(L1)C
This expands to:
M0L1T−1=MA+BL−3B+CT−2A
Step 4: Set up equations for each dimension
Now we equate the powers of M, L, and T on both sides:
1. For mass M:
0=A+B(Equation 1)
2. For length L:
1=−3B+C(Equation 2)
3. For time T:
−1=−2A(Equation 3)
Step 5: Solve for A
From Equation 3:
−1=−2A⟹A=12
Step 6: Solve for B
Substituting A into Equation 1:
0=12+B⟹B=−12
Step 7: Solve for C
Now substitute B into Equation 2:
1=−3(−12)+C⟹1=32+C⟹C=1−32=−12
Step 8: Write the final expression for v
Now we have:
v∝σ12⋅ρ−12⋅λ−12
This can be rewritten as:
v∝√σ√ρ⋅√λ
Step 9: Find v2
To find v2, we square both sides:
v2∝σρ⋅λ
Final Answer
Thus, the square of the speed v2 is proportional to:
v2∝σρλ
We know that the speed v depends on σ, ρ, and λ. We can express this relationship as:
v∝σA⋅ρB⋅λC
where A, B, and C are the powers we need to determine.
We need to write down the dimensions of each quantity:
- The dimension of speed v is (v)=M0L1T−1.
- The dimension of surface tension σ is (σ)=M1T−2.
- The dimension of density ρ is (ρ)=M1L−3.
- The dimension of wavelength λ is (λ)=L1.
Now we can substitute the dimensions into our proportionality:
(M0L1T−1)=(M1T−2)A⋅(M1L−3)B⋅(L1)C
This expands to:
M0L1T−1=MA+BL−3B+CT−2A
Now we equate the powers of M, L, and T on both sides:
1. For mass M:
0=A+B(Equation 1)
2. For length L:
1=−3B+C(Equation 2)
3. For time T:
−1=−2A(Equation 3)
From Equation 3:
−1=−2A⟹A=12
Substituting A into Equation 1:
0=12+B⟹B=−12
Now substitute B into Equation 2:
1=−3(−12)+C⟹1=32+C⟹C=1−32=−12
Now we have:
v∝σ12⋅ρ−12⋅λ−12
This can be rewritten as:
v∝√σ√ρ⋅√λ
To find v2, we square both sides:
v2∝σρ⋅λ
Thus, the square of the speed v2 is proportional to:
v2∝σρλ
Topper's Solved these Questions
- UNITS, MEASUREMENTS & ERRORSBOOK - VMC MODULES ENGLISHCHAPTER - UNITS, MEASUREMENTS & ERRORSEXERCISE - Impeccable
51 Videos
- UNITS, MEASUREMENTS & ERRORSBOOK - VMC MODULES ENGLISHCHAPTER - UNITS, MEASUREMENTS & ERRORSEXERCISE - Illustration
24 Videos
- UNITS, MEASUREMENTS & ERRORSBOOK - VMC MODULES ENGLISHCHAPTER - UNITS, MEASUREMENTS & ERRORSEXERCISE - Enable
50 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - IMPECCABLE
56 Videos
- WAVE MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - WAVE MOTIONEXERCISE - JEE ADVANCED ARCHIVE LEVEL 2 (TRUE FALSE TYPE)
4 Videos
Similar Questions
Knowledge Check
The viscous drag on a spherical body moving with a speed v is proportional to
A√vBvC1/√vDv2
It has been observed that velocity of ripple waves produced in water (ρ) , and surface tension (T) . Prove that V2∝T/λρ.
View SolutionObtain the dimensions of linear momentum and surface tension in terms of velocity (v), density (ρ) and frequency(ν) as fundamental units.
View SolutionIf rho is density of falling body and sigma is the density of fluid then the terminal velocity of the falling body is proportional to
View SolutionThe surface density on a copper sphere is σ. The electric field strength on the surface of the sphere is
View SolutionA needle of length l and density ρ will float on a liquid of surface tension σ if its radius r is less than or equal to:
View SolutionA driver is riding a car with velocity vc between two vertical walls on a horizontal surface as shown in figure.A source of sound of frequency 'f' is situated on the car. (vc<<v, where v is the speed of sound in air )
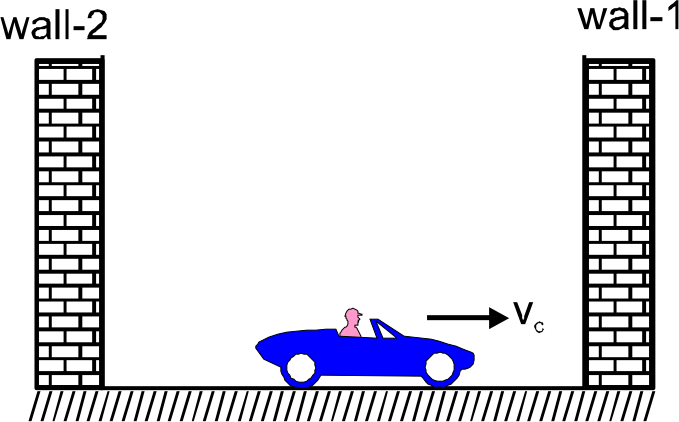
Consider the sound wave observed by the driver directly from car has a wavelength λ1 and the sound wave after reflection from wall-1 observed by the driver has wavelength λ2 then λ1−λ2λ1+λ2 is :
View SolutionChoose the correct option:
A capillary tube of radius r is lowered into a liquid of surface tension T and density ρ. Given angle of contact =0∘.
The work done by surface tension will be
View Solution
