A monkey of mass 20 kg rides on a 40 kg trolley moving at a constant speed of 8 m/s along a horizontal track. Frictional force can be neglected. If the monkey jumps vertically, with respect to the moving trolley, the catch the overhanging branch of tree, the speed of the trolley after the monkey has jumped off is :-
8 m/s
1 m/s
4 m/s
12 m/s
The correct Answer is:D
To solve the problem, we will use the principle of conservation of momentum. Here’s a step-by-step breakdown of the solution:
Step 1: Identify the initial conditions
- The mass of the monkey (m1) = 20 kg
- The mass of the trolley (m2) = 40 kg
- The initial speed of the trolley (v_initial) = 8 m/s
Step 2: Calculate the total initial momentum
The total initial momentum (p_initial) of the system (monkey + trolley) can be calculated using the formula:
pinitial=(m1+m2)×vinitial
Substituting the values:
pinitial=(20kg+40kg)×8m/s
pinitial=60kg×8m/s=480kg m/s
Step 3: Analyze the final conditions after the monkey jumps
After the monkey jumps, the monkey will have a vertical velocity but no horizontal momentum (since it jumps straight up). The trolley will continue to move horizontally with a new speed (v_final).
Step 4: Write the equation for final momentum
The final momentum (p_final) of the system can be expressed as:
pfinal=m1×vmonkey+m2×vtrolley
Since the monkey has no horizontal velocity after jumping, we can say:
pfinal=0+40kg×vtrolley
So,
pfinal=40kg×vtrolley
Step 5: Apply conservation of momentum
According to the conservation of momentum:
pinitial=pfinal
Thus,
480kg m/s=40kg×vtrolley
Step 6: Solve for the final speed of the trolley
Now, we can solve for vtrolley:
vtrolley=480kg m/s40kg
vtrolley=12m/s
Conclusion
The speed of the trolley after the monkey has jumped off is 12 m/s.
---
- The mass of the monkey (m1) = 20 kg
- The mass of the trolley (m2) = 40 kg
- The initial speed of the trolley (v_initial) = 8 m/s
The total initial momentum (p_initial) of the system (monkey + trolley) can be calculated using the formula:
pinitial=(m1+m2)×vinitial
Substituting the values:
pinitial=(20kg+40kg)×8m/s
pinitial=60kg×8m/s=480kg m/s
After the monkey jumps, the monkey will have a vertical velocity but no horizontal momentum (since it jumps straight up). The trolley will continue to move horizontally with a new speed (v_final).
The final momentum (p_final) of the system can be expressed as:
pfinal=m1×vmonkey+m2×vtrolley
Since the monkey has no horizontal velocity after jumping, we can say:
pfinal=0+40kg×vtrolley
So,
pfinal=40kg×vtrolley
According to the conservation of momentum:
pinitial=pfinal
Thus,
480kg m/s=40kg×vtrolley
Now, we can solve for vtrolley:
vtrolley=480kg m/s40kg
vtrolley=12m/s
The speed of the trolley after the monkey has jumped off is 12 m/s.
Topper's Solved these Questions
- WORK ENERGY AND POWER Book:VMC MODULES ENGLISHChapter:WORK ENERGY AND POWER Exercise:ENABLE
Explore 50 Videos
- WORK ENERGY AND POWER Book:VMC MODULES ENGLISHChapter:WORK ENERGY AND POWER Exercise:EFFICIENT
Explore 50 Videos
- WAVE MOTIONBook:VMC MODULES ENGLISHChapter:WAVE MOTIONExercise:JEE ADVANCED ARCHIVE LEVEL 2 (TRUE FALSE TYPE)
Explore 4 Videos
Similar Questions
A trolley of mass 300 ks carrying a sand bag of 25 kg is moving uniformly with a speed of 27km/h on a frictionless track. After a while, sand starts leaking out of a hole on the trolley's floor at the rate of 0.05kgs−1. What is the speed of the trolley after the entire sand bag is empty ?
View SolutionA body of mass 10 kg moves with a constant speed v of 2 ms−1 along a circular path of radius 8 m. The power produced by the body will be
View Solution
Knowledge Check
A trolley of mass 10 kg carries 16 kg grain and moves on a horizontal smooth and straight track at 20ms−1. If the grain start lenking out of a hole at the bottom at time t = 0sec. At the rate of 0.5 kgs−1 the speed of the trolley at t=22s will be nearly
A13.5ms−1B15ms−1C24ms−1D26ms−1
Two particles of mass 1 kg and 0.5 kg are moving in the same direction with speed of 2m/s and 6 m/s respectively on a smooth horizontal surface. The speed of centre of mass of the system is
View SolutionAn object of mass 5 kg moves at a constant speed of 6 m/s in a circular path of radius 2m. Find the magnitude of the object's acceleration and the net force responsible for its motion.
View SolutionA mass of 0.5kg moving with a speed of 1.5m/s on a horizontal smooth surface, collides with a nearly weightless spring of force constant k=50N/m The maximum compression of the spring would be.
View SolutionA car of mass 1000 kg moves on a circular path with constant speed of 16 m/s. It is turned by 90º after travelling 628 m on the road. The centripetal force acting on the car is-
View SolutionOn an object of mass 1 kg moving along x-axis with constant speed 8 m/s, a constant force 2 N is applied in positive y-direction. Find its speed after 4 seconds.
View SolutionA body of mass 5 kg moving with a speed of 1.5 m/s on a horizontal smooth surface collides with a nearly weightless spring of force constant k = 5 N/m. The maximum compression of the spring would be

View SolutionA ball of mass in m=1kg is hung vertically by a thread of length l=1.50m. Upper end of the thread is attached to the ceiling of a trolley of mass M=4kg. Initially, the trolley is stationary and it is free to move along horizontal rails without friction. A shell of mass m=1kg, moving horizontally with velocity v0=6m/s collides with the ball and gets stuck with it. As a result, the thread starts to deflect towards right.
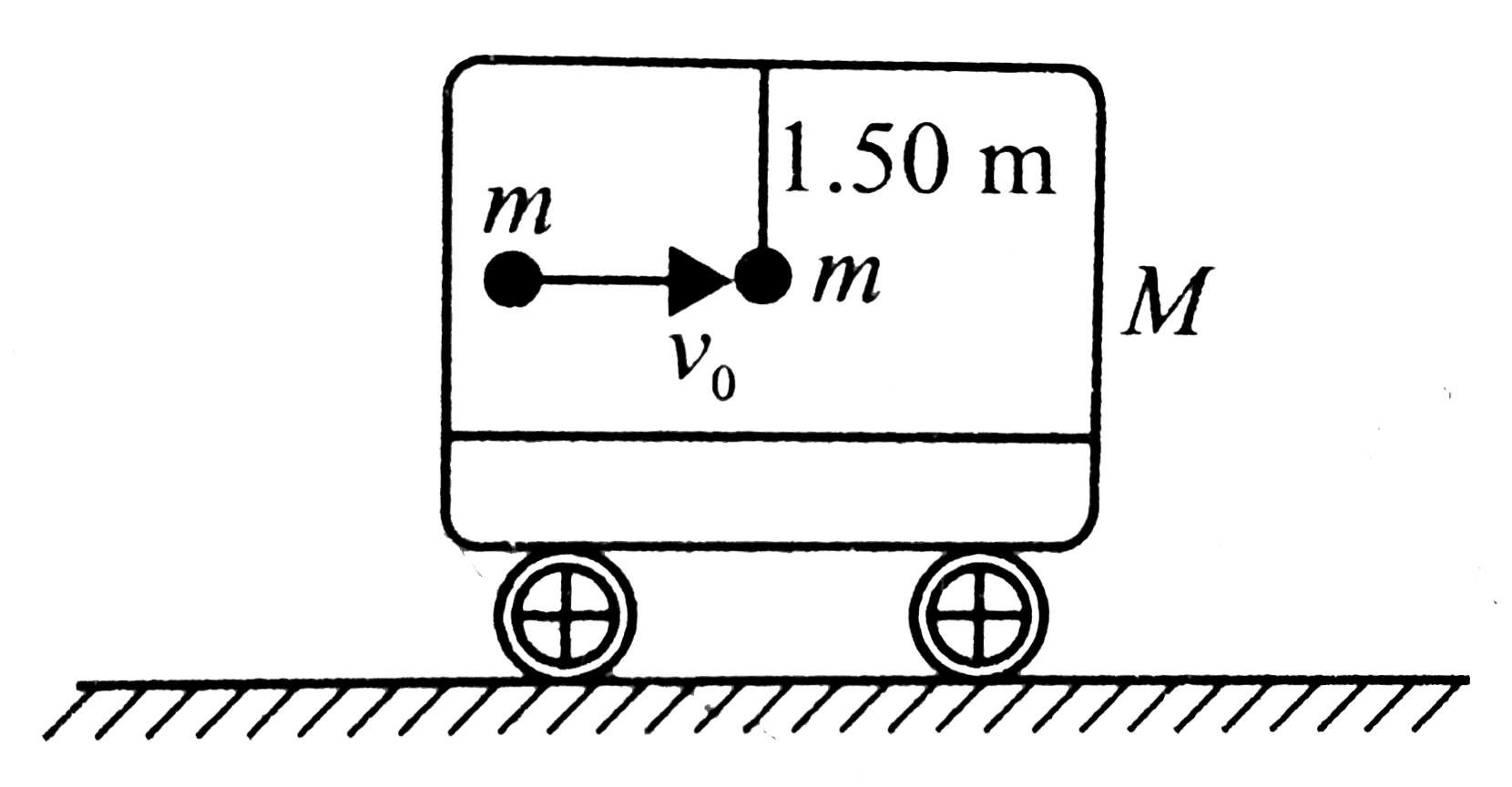
At the time of maximum deflection of the thread with vertical, the trolley will move with velocity
View Solution
